Question and Answers Forum

All Questions      Topic List

Differential Equation Questions

Previous in All Question      Next in All Question      

Previous in Differential Equation      Next in Differential Equation      

Question Number 16475 by Sai dadon. last updated on 22/Jun/17

use L{f′(t)} to find laplace transform of d^2 x/dt^2 +n^2 x=kcoswt given that x=0 and dx/dt=0 when t=0 PLZ HELP.

$${use}\:{L}\left\{{f}'\left({t}\right)\right\}\:{to}\:{find}\:{laplace}\: \\ $$$${transform}\:{of}\:{d}^{\mathrm{2}} {x}/{dt}^{\mathrm{2}} +{n}^{\mathrm{2}} {x}={kcoswt} \\ $$$${given}\:{that}\:{x}=\mathrm{0}\:{and}\:{dx}/{dt}=\mathrm{0}\:{when}\:{t}=\mathrm{0} \\ $$$${PLZ}\:{HELP}. \\ $$$$ \\ $$

Answered by sma3l2996 last updated on 23/Jun/17

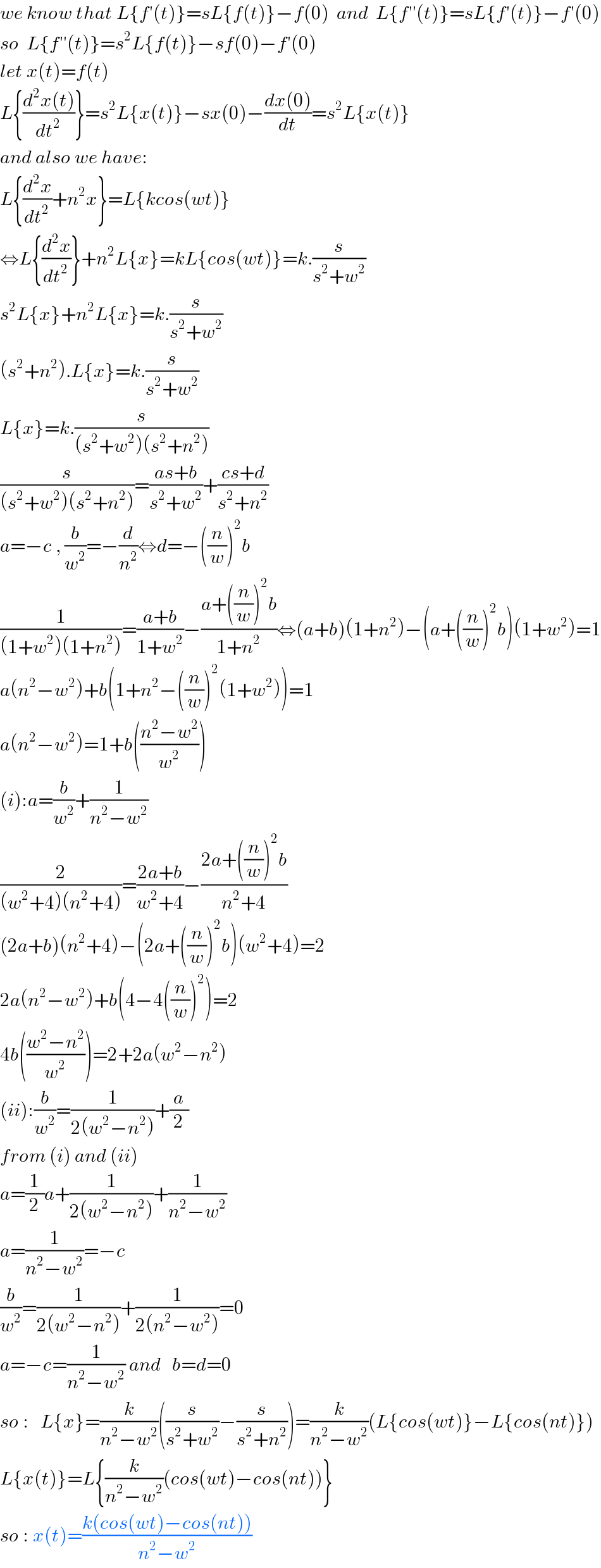
we know that L{f′(t)}=sL{f(t)}−f(0) and L{f′′(t)}=sL{f′(t)}−f′(0) so L{f′′(t)}=s^2 L{f(t)}−sf(0)−f′(0) let x(t)=f(t) L{((d^2 x(t))/dt^2 )}=s^2 L{x(t)}−sx(0)−((dx(0))/dt)=s^2 L{x(t)} and also we have: L{(d^2 x/dt^2 )+n^2 x}=L{kcos(wt)} ⇔L{(d^2 x/dt^2 )}+n^2 L{x}=kL{cos(wt)}=k.(s/(s^2 +w^2 )) s^2 L{x}+n^2 L{x}=k.(s/(s^2 +w^2 )) (s^2 +n^2 ).L{x}=k.(s/(s^2 +w^2 )) L{x}=k.(s/((s^2 +w^2 )(s^2 +n^2 ))) (s/((s^2 +w^2 )(s^2 +n^2 )))=((as+b)/(s^2 +w^2 ))+((cs+d)/(s^2 +n^2 )) a=−c , (b/w^2 )=−(d/n^2 )⇔d=−((n/w))^2 b (1/((1+w^2 )(1+n^2 )))=((a+b)/(1+w^2 ))−((a+((n/w))^2 b)/(1+n^2 ))⇔(a+b)(1+n^2 )−(a+((n/w))^2 b)(1+w^2 )=1 a(n^2 −w^2 )+b(1+n^2 −((n/w))^2 (1+w^2 ))=1 a(n^2 −w^2 )=1+b(((n^2 −w^2 )/w^2 )) (i):a=(b/w^2 )+(1/(n^2 −w^2 )) (2/((w^2 +4)(n^2 +4)))=((2a+b)/(w^2 +4))−((2a+((n/w))^2 b)/(n^2 +4)) (2a+b)(n^2 +4)−(2a+((n/w))^2 b)(w^2 +4)=2 2a(n^2 −w^2 )+b(4−4((n/w))^2 )=2 4b(((w^2 −n^2 )/w^2 ))=2+2a(w^2 −n^2 ) (ii):(b/w^2 )=(1/(2(w^2 −n^2 )))+(a/2) from (i) and (ii) a=(1/2)a+(1/(2(w^2 −n^2 )))+(1/(n^2 −w^2 )) a=(1/(n^2 −w^2 ))=−c (b/w^2 )=(1/(2(w^2 −n^2 )))+(1/(2(n^2 −w^2 )))=0 a=−c=(1/(n^2 −w^2 )) and b=d=0 so : L{x}=(k/(n^2 −w^2 ))((s/(s^2 +w^2 ))−(s/(s^2 +n^2 )))=(k/(n^2 −w^2 ))(L{cos(wt)}−L{cos(nt)}) L{x(t)}=L{(k/(n^2 −w^2 ))(cos(wt)−cos(nt))} so : x(t)=((k(cos(wt)−cos(nt)))/(n^2 −w^2 ))

$${we}\:{know}\:{that}\:{L}\left\{{f}'\left({t}\right)\right\}={sL}\left\{{f}\left({t}\right)\right\}−{f}\left(\mathrm{0}\right)\:\:{and}\:\:{L}\left\{{f}''\left({t}\right)\right\}={sL}\left\{{f}'\left({t}\right)\right\}−{f}'\left(\mathrm{0}\right) \\ $$$${so}\:\:{L}\left\{{f}''\left({t}\right)\right\}={s}^{\mathrm{2}} {L}\left\{{f}\left({t}\right)\right\}−{sf}\left(\mathrm{0}\right)−{f}'\left(\mathrm{0}\right) \\ $$$${let}\:{x}\left({t}\right)={f}\left({t}\right) \\ $$$${L}\left\{\frac{{d}^{\mathrm{2}} {x}\left({t}\right)}{{dt}^{\mathrm{2}} }\right\}={s}^{\mathrm{2}} {L}\left\{{x}\left({t}\right)\right\}−{sx}\left(\mathrm{0}\right)−\frac{{dx}\left(\mathrm{0}\right)}{{dt}}={s}^{\mathrm{2}} {L}\left\{{x}\left({t}\right)\right\} \\ $$$${and}\:{also}\:{we}\:{have}: \\ $$$${L}\left\{\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }+{n}^{\mathrm{2}} {x}\right\}={L}\left\{{kcos}\left({wt}\right)\right\} \\ $$$$\Leftrightarrow{L}\left\{\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }\right\}+{n}^{\mathrm{2}} {L}\left\{{x}\right\}={kL}\left\{{cos}\left({wt}\right)\right\}={k}.\frac{{s}}{{s}^{\mathrm{2}} +{w}^{\mathrm{2}} } \\ $$$${s}^{\mathrm{2}} {L}\left\{{x}\right\}+{n}^{\mathrm{2}} {L}\left\{{x}\right\}={k}.\frac{{s}}{{s}^{\mathrm{2}} +{w}^{\mathrm{2}} } \\ $$$$\left({s}^{\mathrm{2}} +{n}^{\mathrm{2}} \right).{L}\left\{{x}\right\}={k}.\frac{{s}}{{s}^{\mathrm{2}} +{w}^{\mathrm{2}} } \\ $$$${L}\left\{{x}\right\}={k}.\frac{{s}}{\left({s}^{\mathrm{2}} +{w}^{\mathrm{2}} \right)\left({s}^{\mathrm{2}} +{n}^{\mathrm{2}} \right)} \\ $$$$\frac{{s}}{\left({s}^{\mathrm{2}} +{w}^{\mathrm{2}} \right)\left({s}^{\mathrm{2}} +{n}^{\mathrm{2}} \right)}=\frac{{as}+{b}}{{s}^{\mathrm{2}} +{w}^{\mathrm{2}} }+\frac{{cs}+{d}}{{s}^{\mathrm{2}} +{n}^{\mathrm{2}} } \\ $$$${a}=−{c}\:,\:\frac{{b}}{{w}^{\mathrm{2}} }=−\frac{{d}}{{n}^{\mathrm{2}} }\Leftrightarrow{d}=−\left(\frac{{n}}{{w}}\right)^{\mathrm{2}} {b} \\ $$$$\frac{\mathrm{1}}{\left(\mathrm{1}+{w}^{\mathrm{2}} \right)\left(\mathrm{1}+{n}^{\mathrm{2}} \right)}=\frac{{a}+{b}}{\mathrm{1}+{w}^{\mathrm{2}} }−\frac{{a}+\left(\frac{{n}}{{w}}\right)^{\mathrm{2}} {b}}{\mathrm{1}+{n}^{\mathrm{2}} }\Leftrightarrow\left({a}+{b}\right)\left(\mathrm{1}+{n}^{\mathrm{2}} \right)−\left({a}+\left(\frac{{n}}{{w}}\right)^{\mathrm{2}} {b}\right)\left(\mathrm{1}+{w}^{\mathrm{2}} \right)=\mathrm{1} \\ $$$${a}\left({n}^{\mathrm{2}} −{w}^{\mathrm{2}} \right)+{b}\left(\mathrm{1}+{n}^{\mathrm{2}} −\left(\frac{{n}}{{w}}\right)^{\mathrm{2}} \left(\mathrm{1}+{w}^{\mathrm{2}} \right)\right)=\mathrm{1} \\ $$$${a}\left({n}^{\mathrm{2}} −{w}^{\mathrm{2}} \right)=\mathrm{1}+{b}\left(\frac{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} }{{w}^{\mathrm{2}} }\right) \\ $$$$\left({i}\right):{a}=\frac{{b}}{{w}^{\mathrm{2}} }+\frac{\mathrm{1}}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} } \\ $$$$\frac{\mathrm{2}}{\left({w}^{\mathrm{2}} +\mathrm{4}\right)\left({n}^{\mathrm{2}} +\mathrm{4}\right)}=\frac{\mathrm{2}{a}+{b}}{{w}^{\mathrm{2}} +\mathrm{4}}−\frac{\mathrm{2}{a}+\left(\frac{{n}}{{w}}\right)^{\mathrm{2}} {b}}{{n}^{\mathrm{2}} +\mathrm{4}} \\ $$$$\left(\mathrm{2}{a}+{b}\right)\left({n}^{\mathrm{2}} +\mathrm{4}\right)−\left(\mathrm{2}{a}+\left(\frac{{n}}{{w}}\right)^{\mathrm{2}} {b}\right)\left({w}^{\mathrm{2}} +\mathrm{4}\right)=\mathrm{2} \\ $$$$\mathrm{2}{a}\left({n}^{\mathrm{2}} −{w}^{\mathrm{2}} \right)+{b}\left(\mathrm{4}−\mathrm{4}\left(\frac{{n}}{{w}}\right)^{\mathrm{2}} \right)=\mathrm{2} \\ $$$$\mathrm{4}{b}\left(\frac{{w}^{\mathrm{2}} −{n}^{\mathrm{2}} }{{w}^{\mathrm{2}} }\right)=\mathrm{2}+\mathrm{2}{a}\left({w}^{\mathrm{2}} −{n}^{\mathrm{2}} \right) \\ $$$$\left({ii}\right):\frac{{b}}{{w}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}\left({w}^{\mathrm{2}} −{n}^{\mathrm{2}} \right)}+\frac{{a}}{\mathrm{2}} \\ $$$${from}\:\left({i}\right)\:{and}\:\left({ii}\right) \\ $$$${a}=\frac{\mathrm{1}}{\mathrm{2}}{a}+\frac{\mathrm{1}}{\mathrm{2}\left({w}^{\mathrm{2}} −{n}^{\mathrm{2}} \right)}+\frac{\mathrm{1}}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} } \\ $$$${a}=\frac{\mathrm{1}}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} }=−{c} \\ $$$$\frac{{b}}{{w}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}\left({w}^{\mathrm{2}} −{n}^{\mathrm{2}} \right)}+\frac{\mathrm{1}}{\mathrm{2}\left({n}^{\mathrm{2}} −{w}^{\mathrm{2}} \right)}=\mathrm{0} \\ $$$${a}=−{c}=\frac{\mathrm{1}}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} }\:{and}\:\:\:{b}={d}=\mathrm{0} \\ $$$${so}\::\:\:\:{L}\left\{{x}\right\}=\frac{{k}}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} }\left(\frac{{s}}{{s}^{\mathrm{2}} +{w}^{\mathrm{2}} }−\frac{{s}}{{s}^{\mathrm{2}} +{n}^{\mathrm{2}} }\right)=\frac{{k}}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} }\left({L}\left\{{cos}\left({wt}\right)\right\}−{L}\left\{{cos}\left({nt}\right)\right\}\right) \\ $$$${L}\left\{{x}\left({t}\right)\right\}={L}\left\{\frac{{k}}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} }\left({cos}\left({wt}\right)−{cos}\left({nt}\right)\right)\right\} \\ $$$${so}\::\:{x}\left({t}\right)=\frac{{k}\left({cos}\left({wt}\right)−{cos}\left({nt}\right)\right)}{{n}^{\mathrm{2}} −{w}^{\mathrm{2}} } \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com