
Question and Answers Forum
Question Number 165098 by mnjuly1970 last updated on 26/Jan/22

Answered by Eulerian last updated on 26/Jan/22

Commented by Rasheed.Sindhi last updated on 26/Jan/22

Answered by Sheenaynay last updated on 26/Jan/22
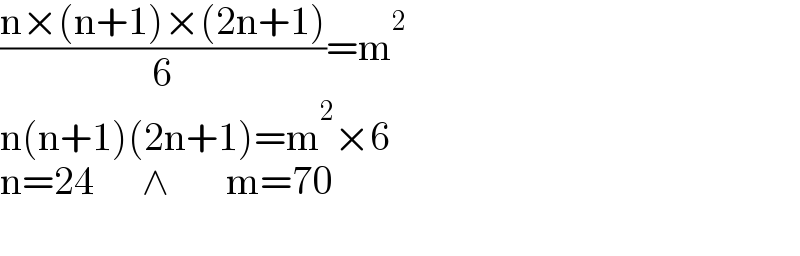
| ||
Question and Answers Forum | ||
Question Number 165098 by mnjuly1970 last updated on 26/Jan/22 | ||
 | ||
Answered by Eulerian last updated on 26/Jan/22 | ||
 | ||
| ||
Commented by Rasheed.Sindhi last updated on 26/Jan/22 | ||
 | ||
Answered by Sheenaynay last updated on 26/Jan/22 | ||
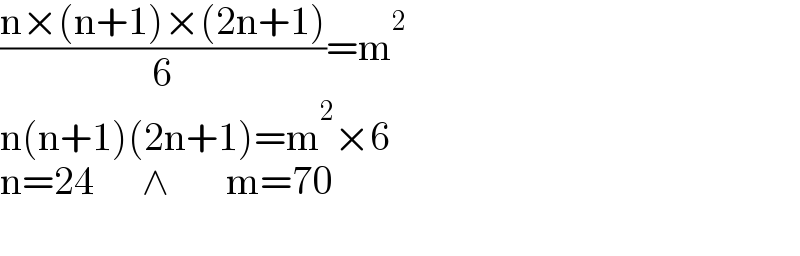 | ||
| ||