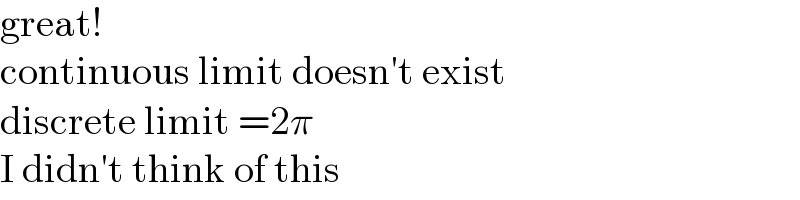Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 167335 by Jamshidbek last updated on 13/Mar/22

x_n =n∙sin(2πen!) Calculate lim_(n→∞) x_n

$$\mathrm{x}_{\mathrm{n}} =\mathrm{n}\centerdot\mathrm{sin}\left(\mathrm{2}\pi\mathrm{en}!\right) \\ $$$$\mathrm{Calculate} \\ $$$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}x}_{\mathrm{n}} \\ $$

Commented by MJS_new last updated on 13/Mar/22

limit doesn′t exist −1≤sin (2πen!) ≤1

$$\mathrm{limit}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{exist} \\ $$$$−\mathrm{1}\leqslant\mathrm{sin}\:\left(\mathrm{2}\pi\mathrm{e}{n}!\right)\:\leqslant\mathrm{1} \\ $$

Commented by Jamshidbek last updated on 13/Mar/22

You are wrong thinking

$$\mathrm{You}\:\mathrm{are}\:\mathrm{wrong}\:\mathrm{thinking} \\ $$

Commented by MJS_new last updated on 13/Mar/22

then explain

$$\mathrm{then}\:\mathrm{explain} \\ $$

Answered by abdomsup last updated on 14/Mar/22

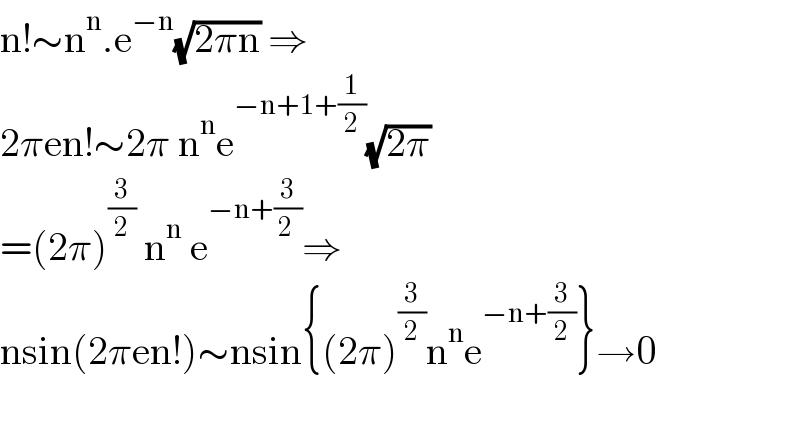
n!∼n^n .e^(−n) (√(2πn)) ⇒ 2πen!∼2π n^n e^(−n+1+(1/2)) (√(2π)) =(2π)^(3/2) n^n e^(−n+(3/(2 ))) ⇒ nsin(2πen!)∼nsin{(2π)^(3/2) n^n e^(−n+(3/2)) }→0

$$\mathrm{n}!\sim\mathrm{n}^{\mathrm{n}} .\mathrm{e}^{−\mathrm{n}} \sqrt{\mathrm{2}\pi\mathrm{n}}\:\Rightarrow \\ $$$$\mathrm{2}\pi\mathrm{en}!\sim\mathrm{2}\pi\:\mathrm{n}^{\mathrm{n}} \mathrm{e}^{−\mathrm{n}+\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}} \sqrt{\mathrm{2}\pi} \\ $$$$=\left(\mathrm{2}\pi\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \:\mathrm{n}^{\mathrm{n}} \:\mathrm{e}^{−\mathrm{n}+\frac{\mathrm{3}}{\mathrm{2}\:}} \Rightarrow \\ $$$$\mathrm{nsin}\left(\mathrm{2}\pi\mathrm{en}!\right)\sim\mathrm{nsin}\left\{\left(\mathrm{2}\pi\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \mathrm{n}^{\mathrm{n}} \mathrm{e}^{−\mathrm{n}+\frac{\mathrm{3}}{\mathrm{2}}} \right\}\rightarrow\mathrm{0} \\ $$$$ \\ $$

Commented by MJS_new last updated on 14/Mar/22

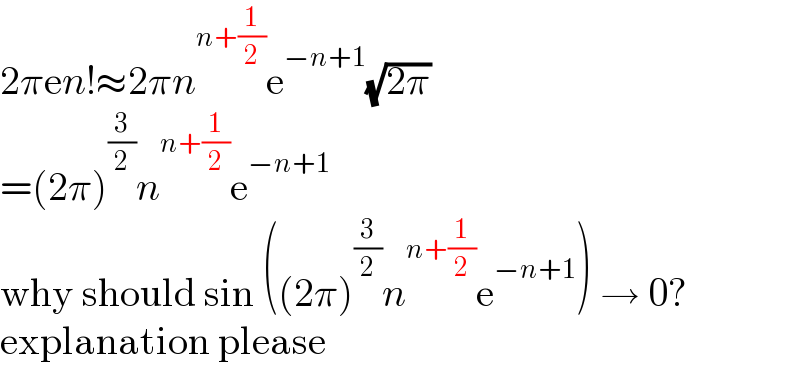
2πen!≈2πn^(n+(1/2)) e^(−n+1) (√(2π)) =(2π)^(3/2) n^(n+(1/2)) e^(−n+1) why should sin ((2π)^(3/2) n^(n+(1/2)) e^(−n+1) ) → 0? explanation please

$$\mathrm{2}\pi\mathrm{e}{n}!\approx\mathrm{2}\pi{n}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \mathrm{e}^{−{n}+\mathrm{1}} \sqrt{\mathrm{2}\pi} \\ $$$$=\left(\mathrm{2}\pi\right)^{\frac{\mathrm{3}}{\mathrm{2}}} {n}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \mathrm{e}^{−{n}+\mathrm{1}} \\ $$$$\mathrm{why}\:\mathrm{should}\:\mathrm{sin}\:\left(\left(\mathrm{2}\pi\right)^{\frac{\mathrm{3}}{\mathrm{2}}} {n}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \mathrm{e}^{−{n}+\mathrm{1}} \right)\:\rightarrow\:\mathrm{0}? \\ $$$$\mathrm{explanation}\:\mathrm{please} \\ $$

Commented by Mathspace last updated on 14/Mar/22

e^(−n) defeat all the function

$${e}^{−{n}} \:{defeat}\:{all}\:{the}\:{function} \\ $$

Commented by MJS_new last updated on 14/Mar/22

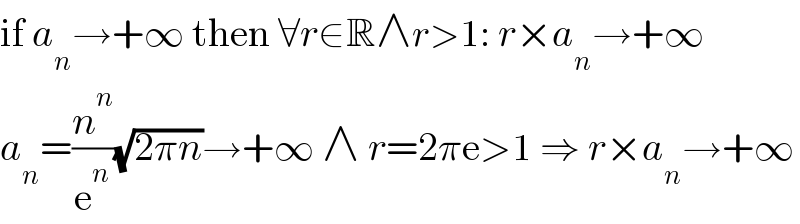
if a_n →+∞ then ∀r∈R∧r>1: r×a_n →+∞ a_n =(n^n /e^n )(√(2πn))→+∞ ∧ r=2πe>1 ⇒ r×a_n →+∞

$$\mathrm{if}\:{a}_{{n}} \rightarrow+\infty\:\mathrm{then}\:\forall{r}\in\mathbb{R}\wedge{r}>\mathrm{1}:\:{r}×{a}_{{n}} \rightarrow+\infty \\ $$$${a}_{{n}} =\frac{{n}^{{n}} }{\mathrm{e}^{{n}} }\sqrt{\mathrm{2}\pi{n}}\rightarrow+\infty\:\wedge\:{r}=\mathrm{2}\pi\mathrm{e}>\mathrm{1}\:\Rightarrow\:{r}×{a}_{{n}} \rightarrow+\infty \\ $$

Answered by qaz last updated on 15/Mar/22

en!=Σ_(k=0) ^n ((n!)/(k!))+Σ_(k=n+1) ^∞ ((n!)/(k!)) =N^+ +(1/(n+1))+(1/((n+1)(n+2)))+... =N^+ +(1/n)(1−(1/n)+(1/n^2 )−...)+(1/n^2 )(1−(1/n)+(1/n^2 )−...)(1−(2/n)+(4/n^2 )−...)+... =N^+ +(1/n)−(1/n^3 )+... ⇒lim_(n→∞) n∙sin (2πen!)=lim_(n→∞) n∙sin [2π(N^+ +(1/n)−(1/n^3 )+...)] =lim_(n→∞) n∙sin [2π((1/n)−(1/n^3 )+...)] =lim_(n→∞) n∙[2π((1/n)−(1/n^3 )+...)−(((2π)^3 )/(3!))∙((1/n)−(1/n^3 )+...)^3 +...] =lim_(n→∞) [2π−((6π+4π^3 )/(3n^2 ))+O((1/n^3 ))] =2π

$$\mathrm{en}!=\underset{\mathrm{k}=\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\frac{\mathrm{n}!}{\mathrm{k}!}+\underset{\mathrm{k}=\mathrm{n}+\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{n}!}{\mathrm{k}!} \\ $$$$=\mathbb{N}^{+} +\frac{\mathrm{1}}{\mathrm{n}+\mathrm{1}}+\frac{\mathrm{1}}{\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{n}+\mathrm{2}\right)}+... \\ $$$$=\mathrm{N}^{+} +\frac{\mathrm{1}}{\mathrm{n}}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{n}}+\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }−...\right)+\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{n}}+\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }−...\right)\left(\mathrm{1}−\frac{\mathrm{2}}{\mathrm{n}}+\frac{\mathrm{4}}{\mathrm{n}^{\mathrm{2}} }−...\right)+... \\ $$$$=\mathrm{N}^{+} +\frac{\mathrm{1}}{\mathrm{n}}−\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{3}} }+... \\ $$$$\Rightarrow\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}n}\centerdot\mathrm{sin}\:\left(\mathrm{2}\pi\mathrm{en}!\right)=\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}n}\centerdot\mathrm{sin}\:\left[\mathrm{2}\pi\left(\mathrm{N}^{+} +\frac{\mathrm{1}}{\mathrm{n}}−\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{3}} }+...\right)\right] \\ $$$$=\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}n}\centerdot\mathrm{sin}\:\left[\mathrm{2}\pi\left(\frac{\mathrm{1}}{\mathrm{n}}−\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{3}} }+...\right)\right] \\ $$$$=\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}n}\centerdot\left[\mathrm{2}\pi\left(\frac{\mathrm{1}}{\mathrm{n}}−\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{3}} }+...\right)−\frac{\left(\mathrm{2}\pi\right)^{\mathrm{3}} }{\mathrm{3}!}\centerdot\left(\frac{\mathrm{1}}{\mathrm{n}}−\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{3}} }+...\right)^{\mathrm{3}} +...\right] \\ $$$$=\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\left[\mathrm{2}\pi−\frac{\mathrm{6}\pi+\mathrm{4}\pi^{\mathrm{3}} }{\mathrm{3n}^{\mathrm{2}} }+\mathcal{O}\left(\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{3}} }\right)\right] \\ $$$$=\mathrm{2}\pi \\ $$

Commented by MJS_new last updated on 15/Mar/22

great! continuous limit doesn′t exist discrete limit =2π I didn′t think of this

$$\mathrm{great}! \\ $$$$\mathrm{continuous}\:\mathrm{limit}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{exist} \\ $$$$\mathrm{discrete}\:\mathrm{limit}\:=\mathrm{2}\pi \\ $$$$\mathrm{I}\:\mathrm{didn}'\mathrm{t}\:\mathrm{think}\:\mathrm{of}\:\mathrm{this} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com