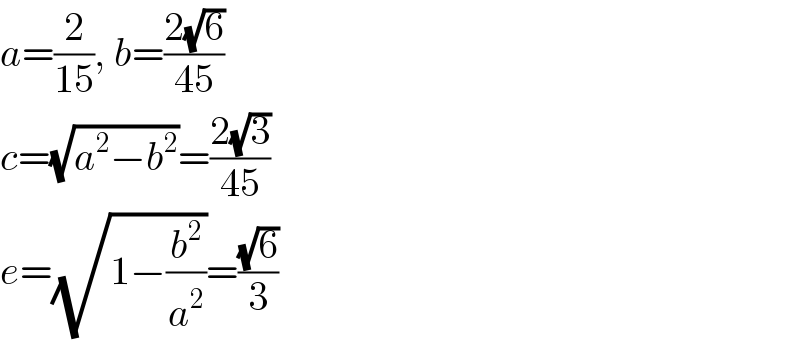91x^2 +84y^2 −24xy+406x−392y+799=0 find the eccentricity,focus,length of major & minor axis,directrix & length of eccentric perpendicular


Question and Answers Forum

All Questions Topic List
Coordinate Geometry Questions
Previous in All Question Next in All Question
Previous in Coordinate Geometry Next in Coordinate Geometry
Question Number 168367 by MdNafiz last updated on 09/Apr/22

$91 x^{2} + 84 y^{2} - 24 x y + 406 x - 392 y + 799 = 0$ $f i n d t h e e c c e n t r i c i t y, f o c u s, l e n g t h o f m a j o r & m i n o r a x i s, d i r e c t r i x & l e n g t h o f e c c e n t r i c p e r p e n d i c u l a r$
	Answered by mr W last updated on 10/Apr/22

	$91 x^{2} - 2 (12 y - 203) x + 84 y^{2} - 392 y + 799 = 0$ $Δ = {(12 y - 203)}^{2} - 91 (84 y^{2} - 392 y + 799) ⩾ 0$ $75 y^{2} - 308 y + 315 ⩽ 0$ $\frac{154 - \sqrt{91}}{75} ⩽ y ⩽ \frac{154 + \sqrt{91}}{75}$ $\Rightarrow y_{C} = \frac{154}{75}$ $84 y^{2} - 2 (12 x + 196) y + 91 x^{2} + 406 x + 799 = 0$ $Δ = {(12 x + 196)}^{2} - 84 (91 x^{2} + 406 x + 799) ⩾ 0$ $75 x^{2} + 294 x + 287 ⩽ 0$ $\frac{- 147 - 2 \sqrt{21}}{75} ⩽ x ⩽ \frac{- 147 + 2 \sqrt{21}}{75}$ $\Rightarrow x_{C} = - \frac{147}{75}$ $x = - \frac{147}{75} + ρ \cos θ$ $y = \frac{154}{75} + ρ \sin θ$ $91 {(- \frac{147}{75} + ρ \cos θ)}^{2} + 84 {(\frac{154}{75} + ρ \sin θ)}^{2} - 24 (- \frac{147}{75} + ρ \cos θ) (\frac{154}{75} + ρ \sin θ) + 406 (- \frac{147}{75} + ρ \cos θ) - 392 (\frac{154}{75} + ρ \sin θ) + 799 = 0$ $(175 + 7 \cos 2 θ - 24 \sin 2 θ) ρ^{2} - \frac{8}{3} = 0$ $ρ^{2} = \frac{8}{3 (175 + 7 \cos 2 θ - 24 \sin 2 θ)}$ $ρ^{2} = \frac{8}{3 [175 + 25 (\frac{7}{25} \cos 2 θ - \frac{24}{25} \sin 2 θ)]}$ $ρ^{2} = \frac{8}{75 [7 - \sin (2 θ - \tan^{- 1} \frac{7}{24})]}$ $ρ_{m a x}^{2} = \frac{8}{75 (7 - 1)}$ $\Rightarrow ρ_{m a x} = \frac{2}{15} = s e m i m a j o r a x i s a$ $a t 2 θ - \tan^{- 1} \frac{7}{24} = \frac{π}{2} o r θ_{1} = \frac{π}{4} + \frac{1}{2} \tan^{- 1} \frac{7}{24}$ $ρ_{m i n}^{2} = \frac{8}{75 (7 + 1)}$ $\Rightarrow ρ_{m i n} = \frac{2 \sqrt{6}}{45} = s e m i m i n o r a x i s b$ $a t 2 θ - \tan^{- 1} \frac{7}{24} = - \frac{π}{2} o r θ_{2} = - \frac{π}{4} + \frac{1}{2} \tan^{- 1} \frac{7}{24}$ $\tan θ_{1} = \frac{4}{3}$ $\tan θ_{2} = - \frac{3}{4}$ $. . .$
	Commented byTawa11 last updated on 10/Apr/22

	$Great sir .$
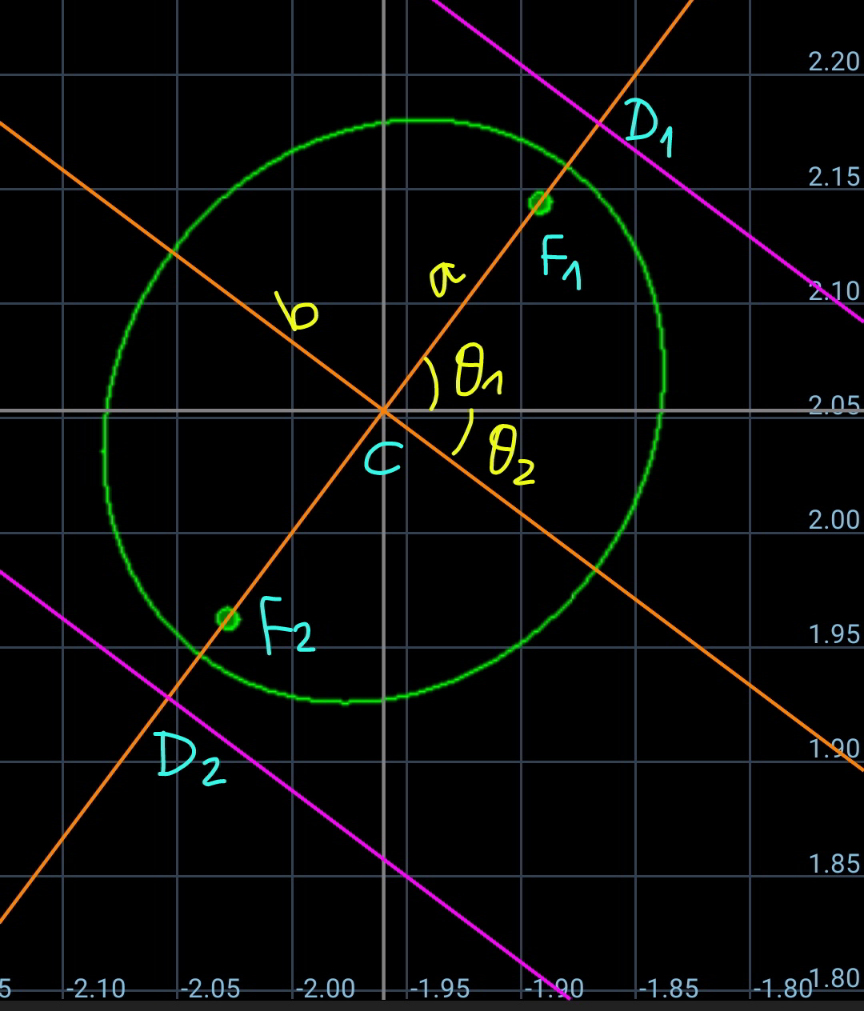
	Commented bymr W last updated on 10/Apr/22

	Commented bymr W last updated on 10/Apr/22

	$a = \frac{2}{15}, b = \frac{2 \sqrt{6}}{45}$ $c = \sqrt{a^{2} - b^{2}} = \frac{2 \sqrt{3}}{45}$ $e = \sqrt{1 - \frac{b^{2}}{a^{2}}} = \frac{\sqrt{6}}{3}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum