ABC is a triangle in which the bisector of angle at B meet the side AC at D, and the bisector of the angle BDC is parallel to the side AB. Prove that the △ABC is issoceles triangle.


Question and Answers Forum

All Questions Topic List
None Questions
Previous in All Question Next in All Question
Previous in None Next in None
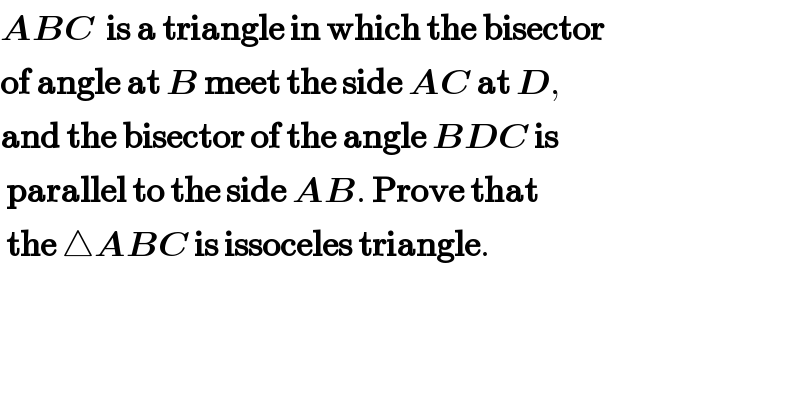
Question Number 169145 by MathsFan last updated on 24/Apr/22

$A B C is a triangle in which the bisector$ $of angle at B meet the side A C at D,$ $and the bisector of the angle B D C is$ $parallel to the side A B . Prove that$ $the △ A B C is issoceles triangle .$
	Answered by som(math1967) last updated on 25/Apr/22

	$l e t b i s e c t o r o f ∠ B D C m e e t t h e$ $s i d e B C a t E$ $∠ A B D = ∠ D B E = α [B D b i s e c t o r]$ $∠ A B D = ∠ B D E [a l t e r n a t e]$ $∠ B D E = ∠ E D C = α [D E b i s e c t o r]$ $A B ∥ D E ∴ ∠ E D C = ∠ B A C = α$ $A C = b, A B = c, B C = a$ $∴ \frac{a}{s i n α} = \frac{b}{s i n 2 α}$ $\Rightarrow \frac{a}{s i n α} = \frac{b}{2 s i n α c o s α}$ $\Rightarrow c o s α = \frac{b}{2 a}$ $\Rightarrow \frac{b^{2} + c^{2} - a^{2}}{2 b c} = \frac{b}{2 a}$ $\Rightarrow b^{2} a + c^{2} a - a^{3} - b^{2} c = 0$ $\Rightarrow b^{2} (a - c) - a (a^{2} - c^{2}) = 0$ $\Rightarrow (a - c) (b^{2} - a c - a^{2}) = 0$ $a - c = 0 \Rightarrow a = c$ $t r i a n g l e i s i s o s c a l e$
	Commented by som(math1967) last updated on 25/Apr/22

	Commented by som(math1967) last updated on 25/Apr/22

	$a n y o t h e r m e t h o d w i t h o u t$ $t r i g o n o m e t r y ?$
	Commented by MathsFan last updated on 25/Apr/22

	$t h a n k y o u s i r$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum