
Question and Answers Forum
Question Number 169815 by mr W last updated on 09/May/22
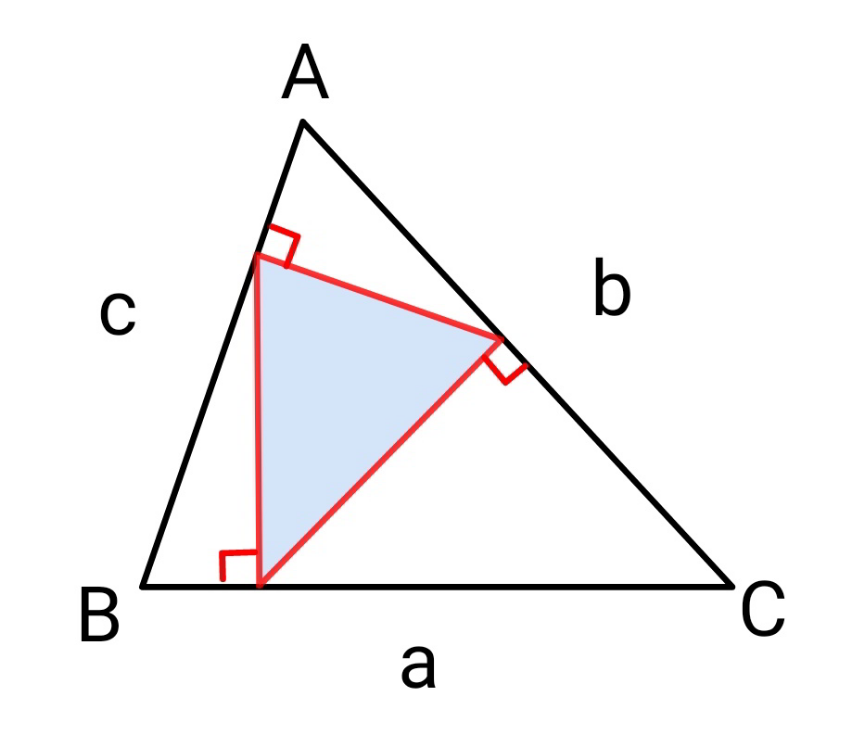
Commented by mr W last updated on 10/May/22
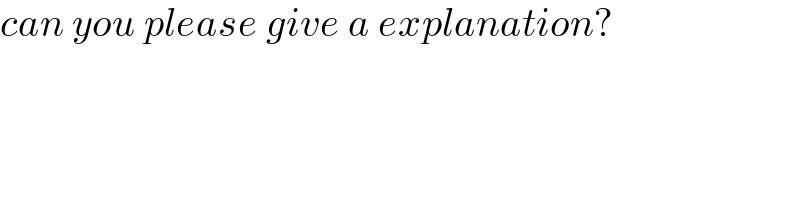
Commented by mr W last updated on 09/May/22

Commented by Rasheed.Sindhi last updated on 10/May/22
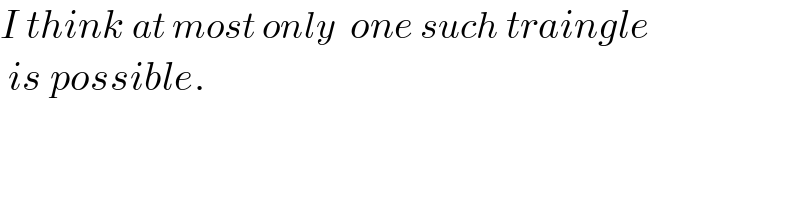
Commented by mr W last updated on 10/May/22

Commented by Rasheed.Sindhi last updated on 10/May/22

Commented by Rasheed.Sindhi last updated on 10/May/22

Commented by mr W last updated on 10/May/22
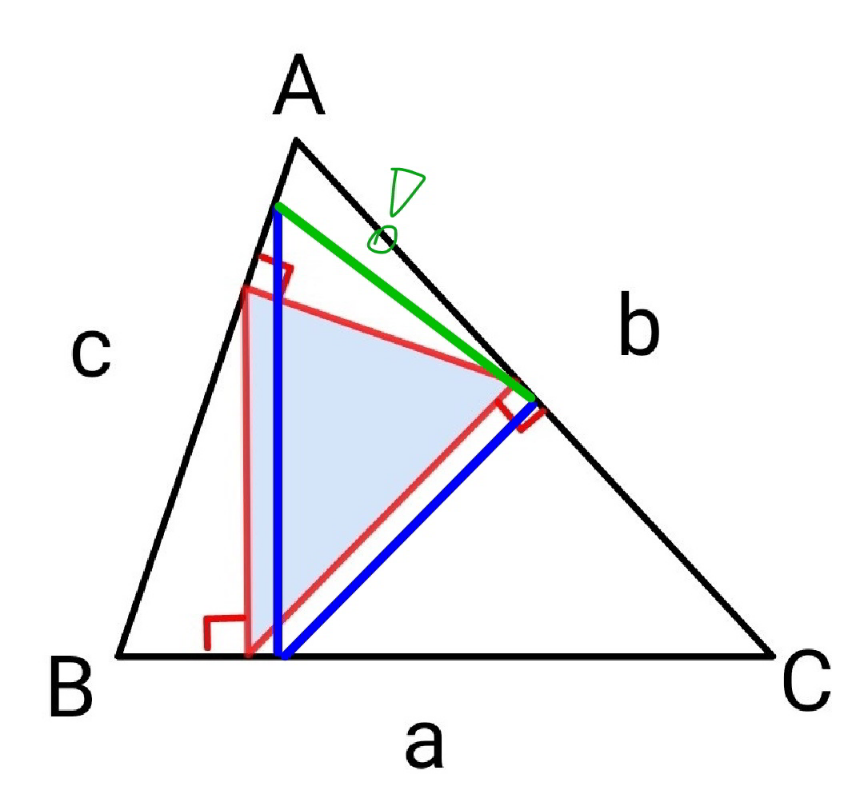
Commented by mr W last updated on 10/May/22

Answered by mr W last updated on 10/May/22
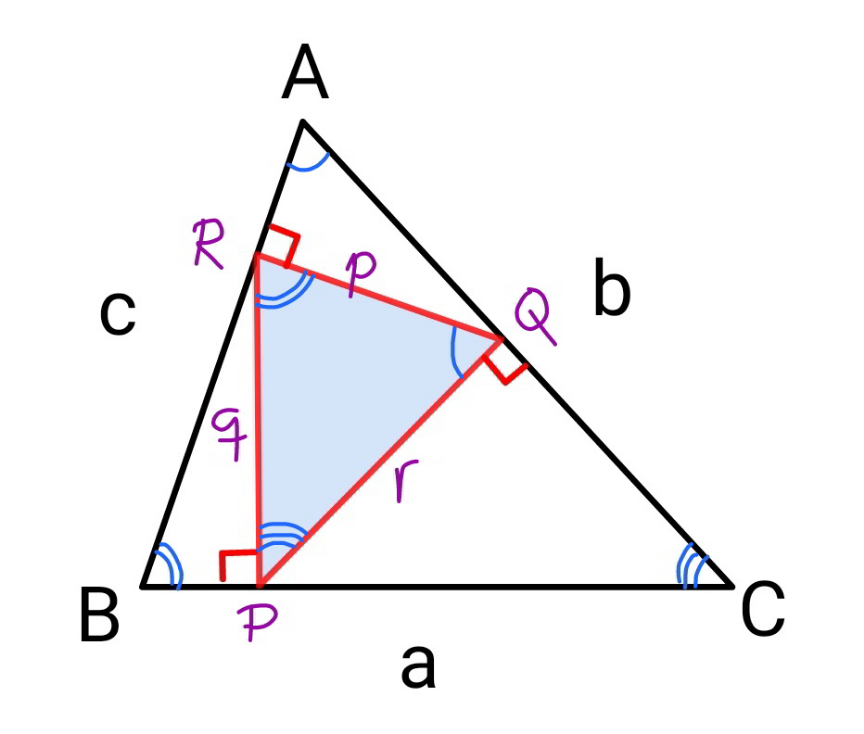
Commented by mr W last updated on 10/May/22

Commented by Tawa11 last updated on 08/Oct/22

