Question Number 170349 by antoineop last updated on 21/May/22
$$ \\ $$$${help}\:{please}\:\: \\ $$$$\overset{\infty} {\int}_{\mathrm{1}} \left(\frac{\mathrm{1}}{{t}}−\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{{t}}\right)\right)\:{dt} \\ $$$${I}\:{can}\:{show}\:{that}\:{it}\:{is}\:{equal}\:{to} \\ $$$$−\underset{{n}\geqslant\mathrm{0}} {\sum}\left(−\mathrm{1}\right)^{{n}} \frac{\left(\mathrm{2}{n}−\mathrm{1}\right)!}{\mathrm{4}^{{n}} \left({n}!\right)^{\mathrm{2}} \left(\mathrm{2}{n}+\mathrm{1}\right)} \\ $$$${but}\:{I}\:{cant}\:{calculate}\:{it}... \\ $$
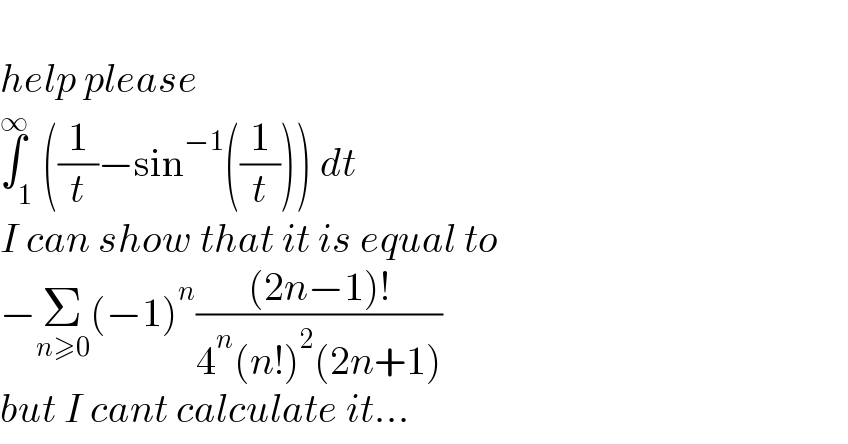
Answered by aleks041103 last updated on 21/May/22
![(1/( (√(1−x^2 ))))=(1+(−x^2 ))^(−1/2) (1+z)^a =1+az+((a(a−1))/2)z^2 +((a(a−1)(a−2))/(3!))z^3 +...= =1+Σ_(i=1) ^∞ (z^i /(i!))Π_(j=0) ^(i−1) (a−j) (1/( (√(1−x^2 ))))=1+Σ_(i=1) ^∞ (((−x^2 )^i )/(i!))Π_(j=0) ^(i−1) (−(1/2)−j)= =1+Σ_(i=1) ^∞ (((−1)^i x^(2i) )/(i!)) Π_(j=0) ^(i−1) (−(1/2))(2j+1)= Π_(j=0) ^(i−1) (−(1/2))(2j+1)=(−(1/2))^i (1.3. ... .(2i−1)) 1.3. ... .(2i−1)=(((2i)!)/(2^i i!)) (1/( (√(1−x^2 ))))=1+Σ_(i=1) ^∞ (((2i)!)/(4^i (i!)^2 ))x^(2i) =Σ_(i=0) ^∞ (((2i)!)/(4^i (i!)^2 ))x^(2i) sin^(−1) (x)=∫_0 ^( x) (dx/( (√(1−x^2 ))))= =Σ_(i=0) ^∞ (((2i)!)/(4^i (i!)^2 ))∫_0 ^( x) x^(2i) dx=Σ_(i=0) ^∞ (((2i)!)/(4^i (2i+1)(i!)^2 ))x^(2i+1) (1/t)−sin^(−1) (t)= =(1/t)−Σ_(i=0) ^∞ (((2i)!)/(4^i (2i+1)(i!)^2 )) (1/t^(2i+1) )= =−Σ_(i=1) ^∞ (((2i)!)/(4^i (2i+1)(i!)^2 )) (1/t^(2i+1) ) ⇒∫_1 ^∞ ((1/t)−asin((1/t)))dt= =−Σ_(i=1) ^∞ (((2i)!)/(4^i (2i+1)(i!)^2 )) ∫_1 ^∞ t^(−2i−1) dt= =−Σ_(i=1) ^∞ (((2i)!)/(4^i (2i+1)(i!)^2 )) ((t^(−2i) /(−2i))]_1 ^∞ = =−Σ_(i=1) ^∞ (((2i−1)!)/(4^i (2i+1)(i!)^2 ))](Q170357.png)
$$\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}=\left(\mathrm{1}+\left(−{x}^{\mathrm{2}} \right)\right)^{−\mathrm{1}/\mathrm{2}} \\ $$$$\left(\mathrm{1}+{z}\right)^{{a}} =\mathrm{1}+{az}+\frac{{a}\left({a}−\mathrm{1}\right)}{\mathrm{2}}{z}^{\mathrm{2}} +\frac{{a}\left({a}−\mathrm{1}\right)\left({a}−\mathrm{2}\right)}{\mathrm{3}!}{z}^{\mathrm{3}} +...= \\ $$$$=\mathrm{1}+\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{z}^{{i}} }{{i}!}\underset{{j}=\mathrm{0}} {\overset{{i}−\mathrm{1}} {\prod}}\left({a}−{j}\right) \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}=\mathrm{1}+\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−{x}^{\mathrm{2}} \right)^{{i}} }{{i}!}\underset{{j}=\mathrm{0}} {\overset{{i}−\mathrm{1}} {\prod}}\left(−\frac{\mathrm{1}}{\mathrm{2}}−{j}\right)= \\ $$$$=\mathrm{1}+\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{i}} {x}^{\mathrm{2}{i}} }{{i}!}\:\underset{{j}=\mathrm{0}} {\overset{{i}−\mathrm{1}} {\prod}}\left(−\frac{\mathrm{1}}{\mathrm{2}}\right)\left(\mathrm{2}{j}+\mathrm{1}\right)= \\ $$$$\:\underset{{j}=\mathrm{0}} {\overset{{i}−\mathrm{1}} {\prod}}\left(−\frac{\mathrm{1}}{\mathrm{2}}\right)\left(\mathrm{2}{j}+\mathrm{1}\right)=\left(−\frac{\mathrm{1}}{\mathrm{2}}\right)^{{i}} \:\left(\mathrm{1}.\mathrm{3}.\:...\:.\left(\mathrm{2}{i}−\mathrm{1}\right)\right) \\ $$$$\mathrm{1}.\mathrm{3}.\:...\:.\left(\mathrm{2}{i}−\mathrm{1}\right)=\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{2}^{{i}} {i}!} \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}=\mathrm{1}+\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left({i}!\right)^{\mathrm{2}} }{x}^{\mathrm{2}{i}} =\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left({i}!\right)^{\mathrm{2}} }{x}^{\mathrm{2}{i}} \\ $$$${sin}^{−\mathrm{1}} \left({x}\right)=\int_{\mathrm{0}} ^{\:{x}} \frac{{dx}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}= \\ $$$$=\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left({i}!\right)^{\mathrm{2}} }\int_{\mathrm{0}} ^{\:{x}} {x}^{\mathrm{2}{i}} {dx}=\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left(\mathrm{2}{i}+\mathrm{1}\right)\left({i}!\right)^{\mathrm{2}} }{x}^{\mathrm{2}{i}+\mathrm{1}} \\ $$$$\frac{\mathrm{1}}{{t}}−{sin}^{−\mathrm{1}} \left({t}\right)= \\ $$$$=\frac{\mathrm{1}}{{t}}−\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left(\mathrm{2}{i}+\mathrm{1}\right)\left({i}!\right)^{\mathrm{2}} }\:\frac{\mathrm{1}}{{t}^{\mathrm{2}{i}+\mathrm{1}} }= \\ $$$$=−\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left(\mathrm{2}{i}+\mathrm{1}\right)\left({i}!\right)^{\mathrm{2}} }\:\frac{\mathrm{1}}{{t}^{\mathrm{2}{i}+\mathrm{1}} } \\ $$$$\Rightarrow\int_{\mathrm{1}} ^{\infty} \left(\frac{\mathrm{1}}{{t}}−{asin}\left(\frac{\mathrm{1}}{{t}}\right)\right){dt}= \\ $$$$=−\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left(\mathrm{2}{i}+\mathrm{1}\right)\left({i}!\right)^{\mathrm{2}} }\:\int_{\mathrm{1}} ^{\infty} {t}^{−\mathrm{2}{i}−\mathrm{1}} {dt}= \\ $$$$=−\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}\right)!}{\mathrm{4}^{{i}} \left(\mathrm{2}{i}+\mathrm{1}\right)\left({i}!\right)^{\mathrm{2}} }\:\left(\frac{{t}^{−\mathrm{2}{i}} }{−\mathrm{2}{i}}\right]_{\mathrm{1}} ^{\infty} = \\ $$$$=−\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{i}−\mathrm{1}\right)!}{\mathrm{4}^{{i}} \left(\mathrm{2}{i}+\mathrm{1}\right)\left({i}!\right)^{\mathrm{2}} } \\ $$
Commented by aleks041103 last updated on 21/May/22
Commented by antoineop last updated on 21/May/22
Yep thats what I did, thanks ! (I'm sorry my English is really bad) but I'm supposed to calculate this integral, so, I'm supposed to calculate this sum and I don't know how..
Commented by aleks041103 last updated on 21/May/22
$${The}\:{main}\:{part}\:{of}\:{the}\:{solution}\:{is} \\ $$$${finding}\:{a}\:{series}\:{representation}\:{for} \\ $$$$\:{the}\:{inverse}\:{sine}\:{function}. \\ $$$${After}\:{that}\:{it}'{s}\:{easy}. \\ $$
Commented by antoineop last updated on 21/May/22
My exercise just tell me "Justify the existence of this integral and then, calculate it" I dont know how to calculate this sum, I've also tried IBP but .. still impossible
Commented by aleks041103 last updated on 21/May/22
$${I}\:{dont}\:{think}\:{that}\:{there}\:{is}\:{a}\:{closed}\:{form}. \\ $$
Commented by antoineop last updated on 21/May/22
That's what I think too, I'll ask my teacher next week and share it if he has a way (of not) to calculate that ! thanks :)
Commented by aleks041103 last updated on 21/May/22
$${Your}\:{task}\:{was}\:{to}\:{prove}\:{that}\:{the}\:{imtegral} \\ $$$${converges}\:{and}\:{then}\:{calculate}\:{it},\:{which} \\ $$$${you}'{ve}\:{already}\:{done}\:{by}\:{finding}\:{this}\:{sum} \\ $$
Commented by aleks041103 last updated on 21/May/22
![f(x)=sin^(−1) (x)−x we analyze x∈[0,1] we know that x≥sin(x), x≥0 ⇒sin^(−1) x≥x≥0 ⇒sin^(−1) x−x≥0 ⇒∫_1 ^∞ −f((1/t))dt≤0](Q170367.png)
$${f}\left({x}\right)={sin}^{−\mathrm{1}} \left({x}\right)−{x} \\ $$$${we}\:{analyze}\:{x}\in\left[\mathrm{0},\mathrm{1}\right] \\ $$$${we}\:{know}\:{that} \\ $$$${x}\geqslant{sin}\left({x}\right),\:{x}\geqslant\mathrm{0} \\ $$$$\Rightarrow{sin}^{−\mathrm{1}} {x}\geqslant{x}\geqslant\mathrm{0} \\ $$$$\Rightarrow{sin}^{−\mathrm{1}} {x}−{x}\geqslant\mathrm{0} \\ $$$$\Rightarrow\int_{\mathrm{1}} ^{\infty} −{f}\left(\frac{\mathrm{1}}{{t}}\right){dt}\leqslant\mathrm{0} \\ $$
Commented by antoineop last updated on 22/May/22
yeah but in France in 99% of analysis exercises we are able to calculate directly the sum
Commented by Tawa11 last updated on 08/Oct/22
$$\mathrm{Great}\:\mathrm{sir} \\ $$