
Question and Answers Forum
Question Number 170878 by 0731619 last updated on 02/Jun/22

Answered by thfchristopher last updated on 02/Jun/22
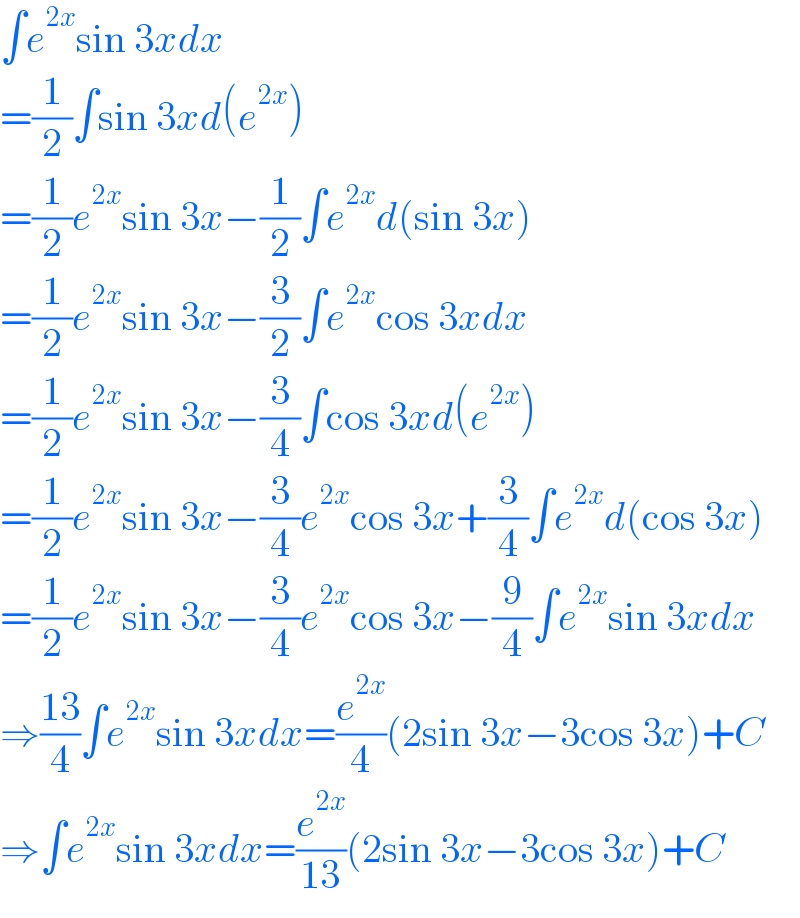
| ||
Question and Answers Forum | ||
Question Number 170878 by 0731619 last updated on 02/Jun/22 | ||
 | ||
Answered by thfchristopher last updated on 02/Jun/22 | ||
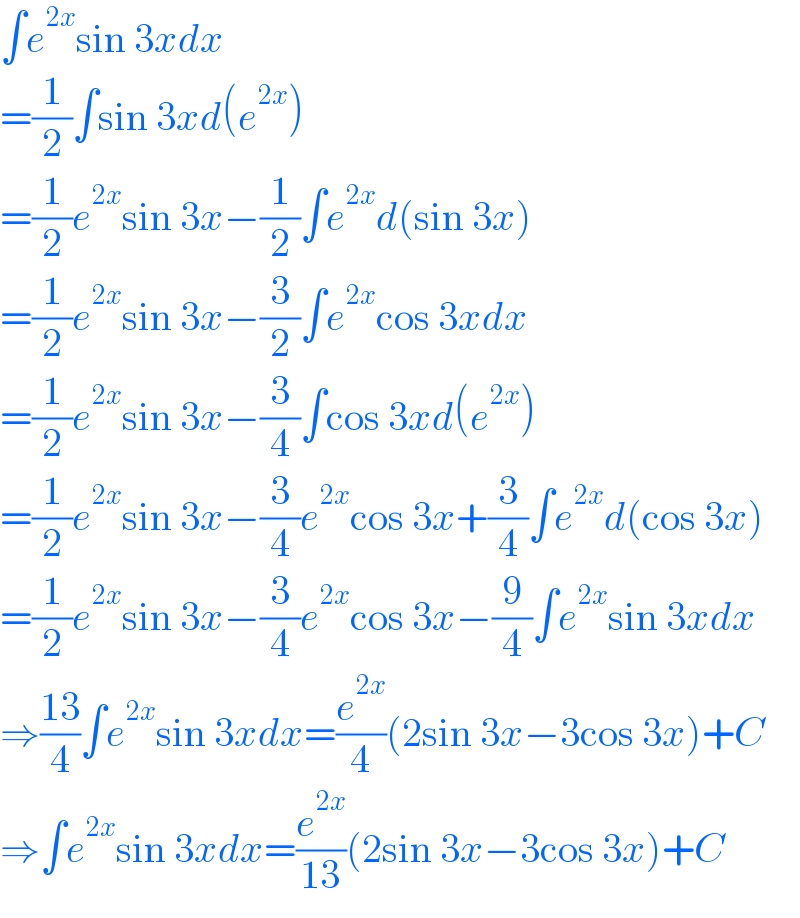 | ||
| ||