Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 171402 by mnjuly1970 last updated on 14/Jun/22

$$solve...$$$$\lfloor x^2\rfloor +\lfloor x{\rfloor }^2=x^2+x+1$$$$x=?$$

Answered by floor(10²Eta[1]) last updated on 14/Jun/22

$$\lfloor {\mathrm{x}}^2\rfloor =\mathrm{a}\in \mathbb{Z}\Rightarrow {\mathrm{x}}^2=\mathrm{a}+\alpha ,0⩽\alpha <1$$$$\lfloor \mathrm{x}\rfloor =\mathrm{b}\in \mathbb{Z}\Rightarrow \mathrm{x}=\mathrm{b}+\beta ,0⩽\beta <1$$$$$$$$\mathrm{a}+{\mathrm{b}}^2=\mathrm{a}+\alpha +\mathrm{b}+\beta +1$$$$\Rightarrow {\mathrm{b}}^2-\mathrm{b}=\alpha +\beta +1\Rightarrow 1⩽{\mathrm{b}}^2-\mathrm{b}<3$$$$\Rightarrow {\mathrm{b}}^2-\mathrm{b}\in \{1,2\}\Rightarrow {\mathrm{b}}^2-\mathrm{b}=2\Rightarrow \mathrm{b}=2\mathrm{or}\mathrm{b}=-1$$$$1\mathrm{case}:\mathrm{b}=2$$$$\lfloor \mathrm{x}\rfloor =2,\mathrm{x}=2+\alpha \Rightarrow {\mathrm{x}}^2=4+4\alpha +{\alpha }^2\Rightarrow 4⩽{\mathrm{x}}^2<9$$$${\mathrm{x}}^2\in [4,5)\therefore \lfloor {\mathrm{x}}^2\rfloor =4$$$$4+2^2={\mathrm{x}}^2+\mathrm{x}+1\Rightarrow {\mathrm{x}}^2+\mathrm{x}-7=0$$$$\Rightarrow \mathrm{x}=\frac{-1+\sqrt{29}}{2}$$$${\mathrm{x}}^2\in [5,6)\therefore \lfloor {\mathrm{x}}^2\rfloor =5$$$$5+2^2={\mathrm{x}}^2+\mathrm{x}+1\Rightarrow {\mathrm{x}}^2+\mathrm{x}-8=0$$$$\Rightarrow \mathrm{x}=\frac{-1+\sqrt{33}}{2}$$$${\mathrm{x}}^2\in [6,7)\therefore \lfloor {\mathrm{x}}^2\rfloor =6$$$$6+2^2={\mathrm{x}}^2+\mathrm{x}+1\Rightarrow {\mathrm{x}}^2+\mathrm{x}-9=0$$$$\mathrm{x}=\frac{-1+\sqrt{37}}{2}$$$${\mathrm{x}}^2\in [7,8)\therefore \lfloor {\mathrm{x}}^2\rfloor =7$$$$7+2^2={\mathrm{x}}^2+\mathrm{x}+1\Rightarrow {\mathrm{x}}^2+\mathrm{x}-10=0$$$$\Rightarrow \mathrm{x}=\frac{-1+\sqrt{41}}{2}$$$${\mathrm{x}}^2\in [7,8)\therefore \lfloor {\mathrm{x}}^2\rfloor =8$$$$\Rightarrow \mathrm{x}=\frac{-1+\sqrt{45}}{2}$$$$2\mathrm{case}:\mathrm{b}=-1$$$$\lfloor \mathrm{x}\rfloor =-1,\mathrm{x}=-1+\alpha \Rightarrow {\mathrm{x}}^2=1-2\alpha +{\alpha }^2\Rightarrow 0<{\mathrm{x}}^2⩽1$$$${\mathrm{x}}^2\in (0,1)\therefore \lfloor {\mathrm{x}}^2\rfloor =0$$$$0+{(-1)}^2={\mathrm{x}}^2+\mathrm{x}+1\Rightarrow {\mathrm{x}}^2+\mathrm{x}=0$$$$\Rightarrow \mathrm{x}=-1\Rightarrow {\mathrm{x}}^2\notin (0,1)\left(\mathrm{dont}\mathrm{work}\right)$$$${\mathrm{x}}^2=1\therefore \lfloor {\mathrm{x}}^2\rfloor =1$$$$1+{(-1)}^2={\mathrm{x}}^2+\mathrm{x}+1\Rightarrow {\mathrm{x}}^2+\mathrm{x}-1=0$$$$\mathrm{x}=\frac{-1-\sqrt{5}}{2}⩽-1\left(\mathrm{dont}\mathrm{work}\right)$$$$$$$$\mathrm{solutions}:$$$$\mathrm{x}=\frac{-1+\sqrt{25+4\mathrm{n}}}{2},1⩽\mathrm{n}⩽5,\mathrm{n}\in \mathbb{N}$$$$$$

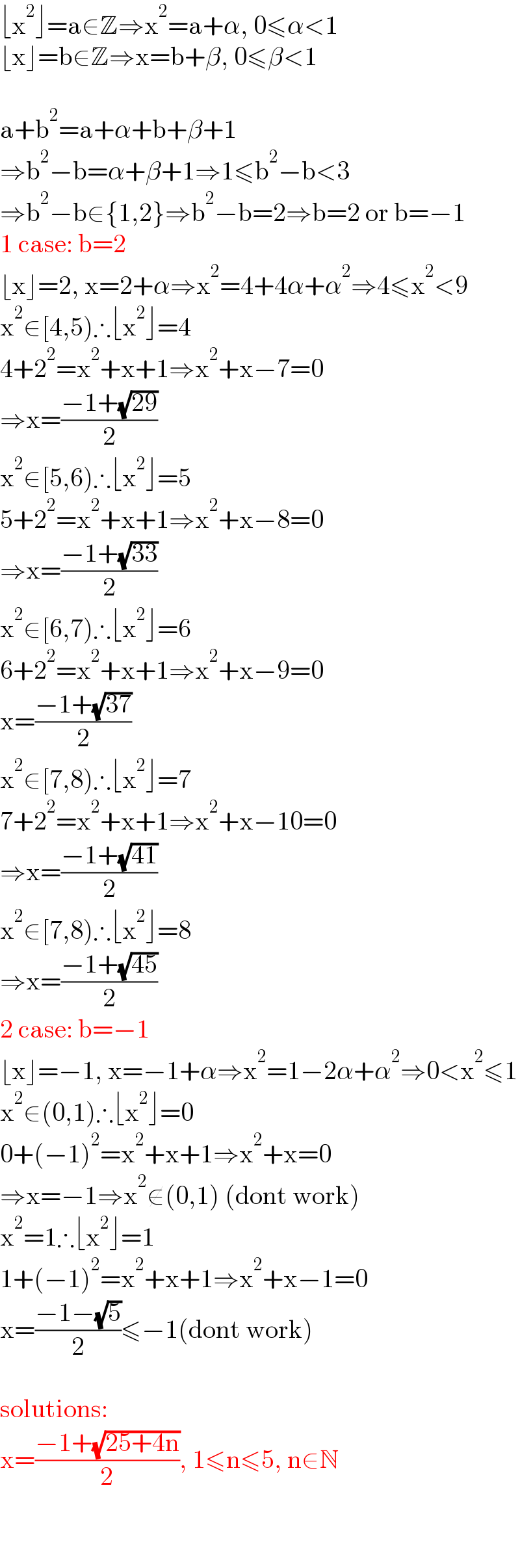
Answered by mr W last updated on 14/Jun/22

$$x=n+f$$$$\lfloor x{\rfloor }^2=n^2$$$$\lfloor x^2\rfloor =n^2+\lfloor (2n+1)f\rfloor$$$$x^2+x+1=n^2+n+1+(2n+1)f+f^2$$$$2n^2+\lfloor (2n+1)f\rfloor =n^2+n+1+(2n+1)f+f^2$$$$n^2-n-1=(2n+1)f+f^2-\lfloor (2n+1)f\rfloor$$$$n^2-n-1=\left\{(2n+1)f\right\}+f^2⩾0$$$$\Rightarrow n⩽-1orn⩾2$$$$n^2-n-1=\left\{(2n+1)f\right\}+f^2<2$$$$\Rightarrow -1⩽n⩽2$$$$\Rightarrow n=-1orn=2$$$$withn=-1,i.e.-1⩽x<0:$$$$1+1=x^2+x+1$$$$x^2+x-1=0$$$$\Rightarrow x=\frac{-1-\sqrt{5}}{2}<-1rejected.$$$$withn=2,i.e.2⩽x<3:$$$$\lfloor x^2\rfloor =kwith4⩽k⩽8$$$$k+4=x^2+x+1$$$$x^2+x-(k+3)=0$$$$\Rightarrow x=\frac{-1+\sqrt{13+4k}}{2}✓$$$$i.e.x=\frac{-1+\sqrt{29}}{2},\frac{-1+\sqrt{33}}{2},\frac{-1+\sqrt{37}}{2},\frac{-1+\sqrt{41}}{2},\frac{-1+\sqrt{45}}{2}$$

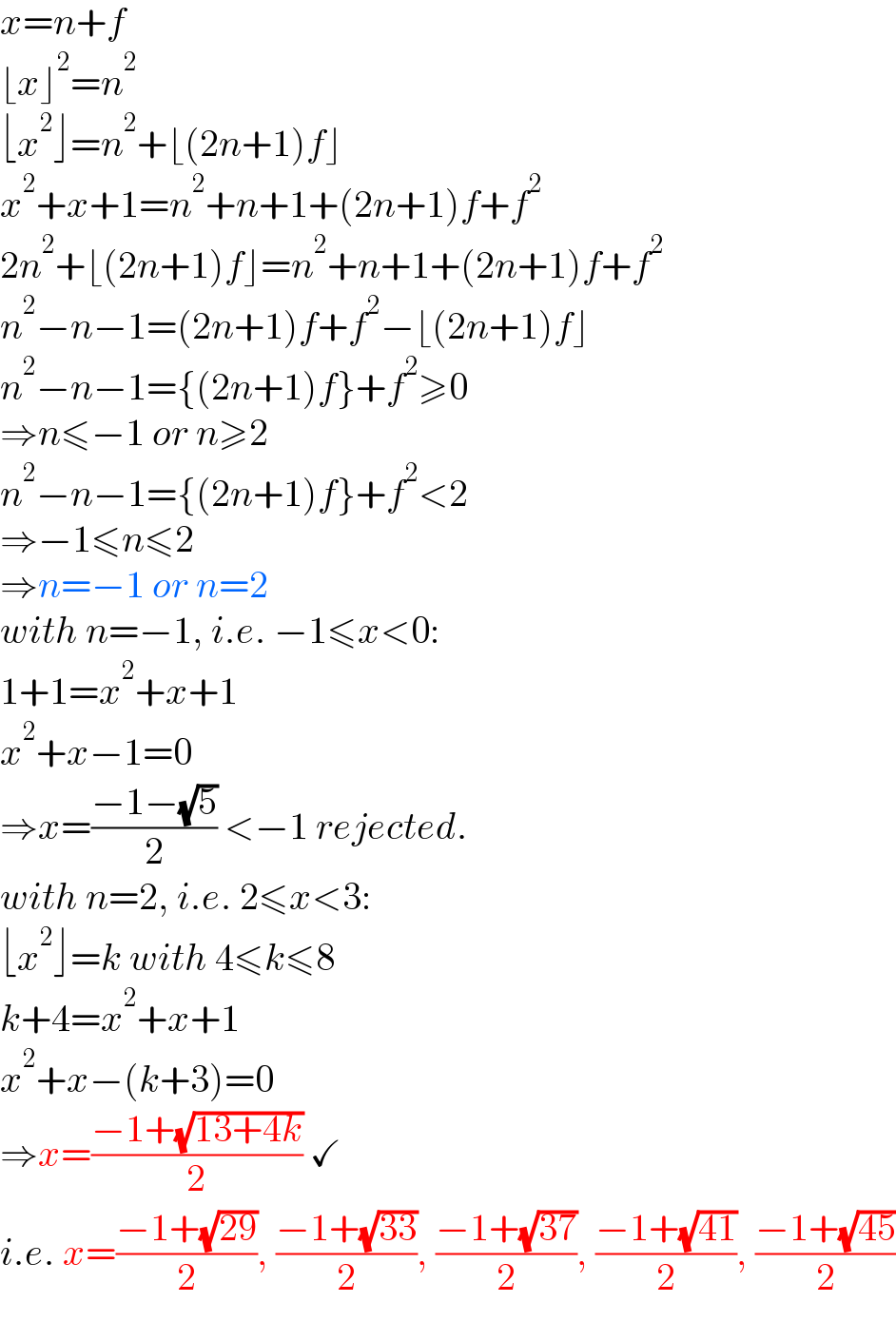
Commented by floor(10²Eta[1]) last updated on 14/Jun/22

$$\mathrm{if}\mathrm{x}=-1\Rightarrow {\mathrm{x}}^2=1$$$$\lfloor {\mathrm{x}}^2\rfloor +\lfloor \mathrm{x}{\rfloor }^2=2\ne {\mathrm{x}}^2+\mathrm{x}+1=1$$

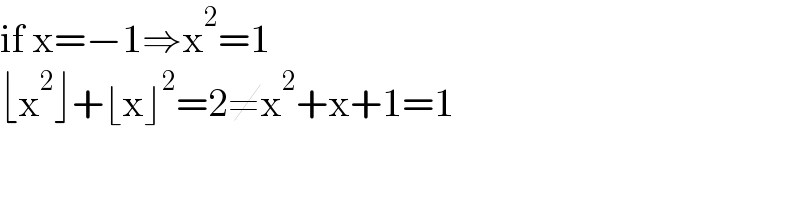
Commented by mr W last updated on 14/Jun/22

$$ihaverejectedx=-1.$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com