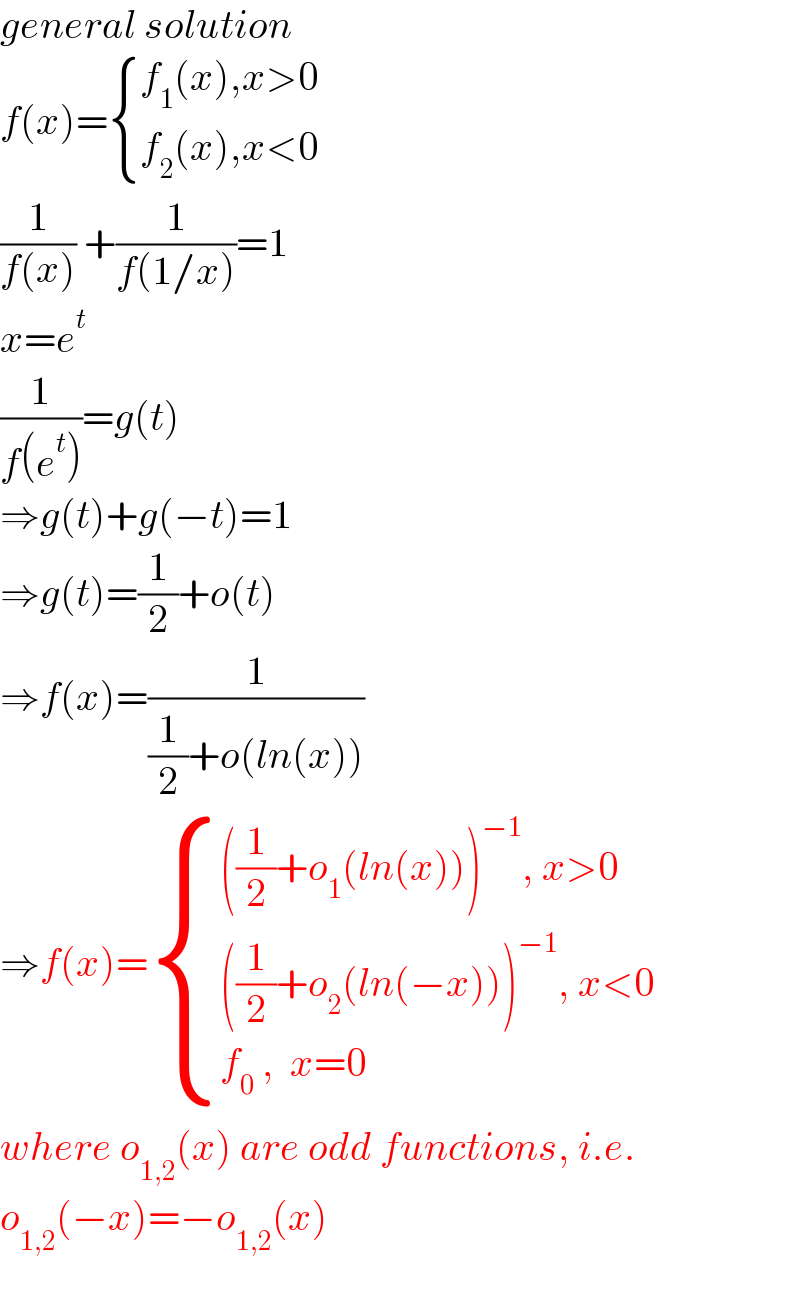Question and Answers Forum
Previous in Relation and Functions Next in Relation and Functions
Question Number 171435 by cortano1 last updated on 15/Jun/22
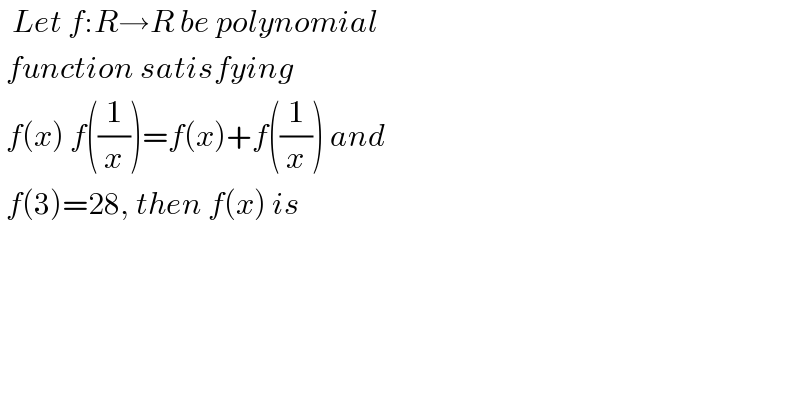
Commented by infinityaction last updated on 15/Jun/22

Commented by infinityaction last updated on 15/Jun/22

Commented by mr W last updated on 15/Jun/22
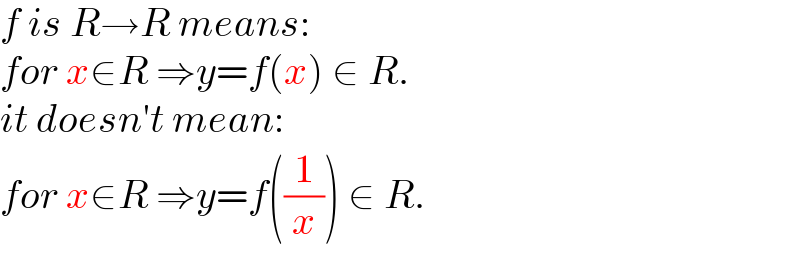
Commented by infinityaction last updated on 15/Jun/22

Commented by floor(10²Eta[1]) last updated on 15/Jun/22

Commented by floor(10²Eta[1]) last updated on 15/Jun/22

Commented by mr W last updated on 15/Jun/22
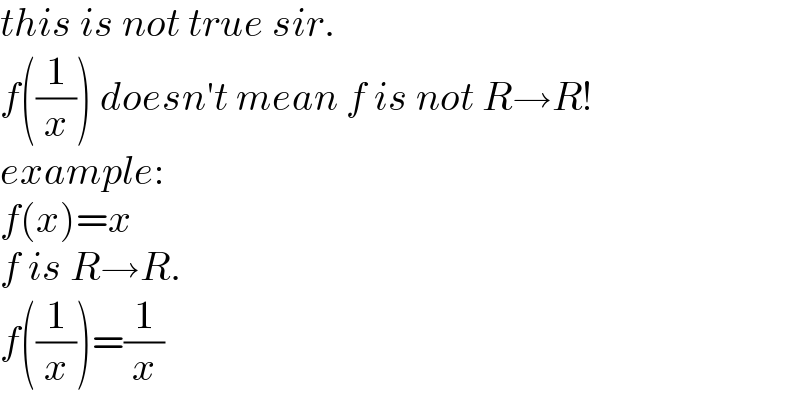
Answered by aleks041103 last updated on 15/Jun/22