
Question and Answers Forum
Question Number 171560 by mnjuly1970 last updated on 17/Jun/22
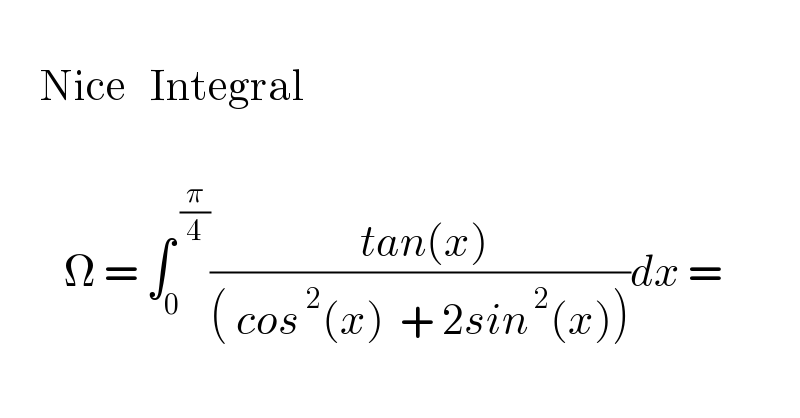
Commented by infinityaction last updated on 17/Jun/22
![Ω = ∫_0 ^(π/4) ((tan x sec^2 x)/(1+2tan^2 x))dx tan x = p → sec^2 x dx = dp Ω = ∫_0 ^1 (p/(1+2p^2 ))dp 1+2p^2 = t → 4pdp = dt Ω = (1/4)∫_1 ^3 (dt/(t )) ⇒ (1/4)[log t]_1 ^3 Ω = (1/4)log 3](Q171561.png)
Answered by floor(10²Eta[1]) last updated on 18/Jun/22
![t=tg((x/2)) cos(x)=1−2sin^2 ((x/2)) 1−(2/(csc^2 ((x/2))))=1−(2/(1+cotg^2 ((x/2)))) 1−(2/(1+(1/t^2 )))=1−((2t^2 )/(t^2 +1))=((1−t^2 )/(1+t^2 )) ⇒sin(x)=((2t)/(1+t^2 )) ⇒((tgx)/(cos^2 x+2sin^2 x))=((tgx)/(1+sin^2 x))=((tgx)/(2−cos^2 x)) =((sinx)/(2cosx−cos^3 x))=(((2t)/(1+t^2 ))/(2(((1−t^2 )/(1+t^2 )))−(((1−t^2 )/(1+t^2 )))^3 )) =((2t(1+t^2 )^2 )/(2(1−t^2 )(1+t^2 )^2 −(1−t^2 )^3 ))=((2t(1+t^2 )^2 )/((1−t^2 )[1+6t^2 +t^4 ])) ⇒∫_0 ^(tg(π/8)) ((2t(1+t^2 )^2 )/((1−t^2 )(t^4 +6t^2 +1)))dx t^2 =u 2tdt=du ∫_0 ^(tg^2 (π/8)) (((1+u)^2 )/((1−u)(u^2 +6u+1)))du =(1/2)∫_0 ^(tg^2 (π/8)) (du/(1−u))−(1/2)∫_0 ^(tg^2 (π/8)) ((u−1)/(u^2 +6u+1))du =−(1/2)ln∣1−u∣_0 ^(tg^2 (π/8)) −(1/2)∫_0 ^(tg^2 (π/8)) ((u−1)/((u+3)^2 −8))du =−(1/2)ln∣1−tg^2 (π/8)∣−(1/2)∫_0 ^(tg^2 (π/8)) ((u−1)/((u+3+2(√2))(u+3−2(√2))))du =−(1/2)ln∣1−tg^2 (π/8)∣−(1/2)(((1+(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3+2(√2)))−(1/2)(((1−(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3−2(√2))) =−(1/2)ln∣1−tg^2 (π/8)∣−(1/2)(((1+(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3+2(√2)))−(1/2)(((1−(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3−2(√2))) =−(1/2)ln∣1−tg^2 (π/8)∣−((1+(√2))/4)[ln∣u+3+2(√2)∣]_0 ^(tg^2 (π/8)) −((1−(√2))/4)[ln∣u+3−2(√2)∣]_0 ^(tg^2 (π/8)) =−(1/2)ln∣1−tg^2 (π/8)∣−((1+(√2))/4)ln∣tg^2 (π/8)+3+2(√2)∣−((1−(√2))/4)ln∣tg^2 (π/8)+3−2(√2)∣+((√2)/2)ln(3+2(√2))](Q171571.png)
| ||
Question and Answers Forum | ||
Question Number 171560 by mnjuly1970 last updated on 17/Jun/22 | ||
 | ||
Commented by infinityaction last updated on 17/Jun/22 | ||
![Ω = ∫_0 ^(π/4) ((tan x sec^2 x)/(1+2tan^2 x))dx tan x = p → sec^2 x dx = dp Ω = ∫_0 ^1 (p/(1+2p^2 ))dp 1+2p^2 = t → 4pdp = dt Ω = (1/4)∫_1 ^3 (dt/(t )) ⇒ (1/4)[log t]_1 ^3 Ω = (1/4)log 3](Q171561.png) | ||
Answered by floor(10²Eta[1]) last updated on 18/Jun/22 | ||
![t=tg((x/2)) cos(x)=1−2sin^2 ((x/2)) 1−(2/(csc^2 ((x/2))))=1−(2/(1+cotg^2 ((x/2)))) 1−(2/(1+(1/t^2 )))=1−((2t^2 )/(t^2 +1))=((1−t^2 )/(1+t^2 )) ⇒sin(x)=((2t)/(1+t^2 )) ⇒((tgx)/(cos^2 x+2sin^2 x))=((tgx)/(1+sin^2 x))=((tgx)/(2−cos^2 x)) =((sinx)/(2cosx−cos^3 x))=(((2t)/(1+t^2 ))/(2(((1−t^2 )/(1+t^2 )))−(((1−t^2 )/(1+t^2 )))^3 )) =((2t(1+t^2 )^2 )/(2(1−t^2 )(1+t^2 )^2 −(1−t^2 )^3 ))=((2t(1+t^2 )^2 )/((1−t^2 )[1+6t^2 +t^4 ])) ⇒∫_0 ^(tg(π/8)) ((2t(1+t^2 )^2 )/((1−t^2 )(t^4 +6t^2 +1)))dx t^2 =u 2tdt=du ∫_0 ^(tg^2 (π/8)) (((1+u)^2 )/((1−u)(u^2 +6u+1)))du =(1/2)∫_0 ^(tg^2 (π/8)) (du/(1−u))−(1/2)∫_0 ^(tg^2 (π/8)) ((u−1)/(u^2 +6u+1))du =−(1/2)ln∣1−u∣_0 ^(tg^2 (π/8)) −(1/2)∫_0 ^(tg^2 (π/8)) ((u−1)/((u+3)^2 −8))du =−(1/2)ln∣1−tg^2 (π/8)∣−(1/2)∫_0 ^(tg^2 (π/8)) ((u−1)/((u+3+2(√2))(u+3−2(√2))))du =−(1/2)ln∣1−tg^2 (π/8)∣−(1/2)(((1+(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3+2(√2)))−(1/2)(((1−(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3−2(√2))) =−(1/2)ln∣1−tg^2 (π/8)∣−(1/2)(((1+(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3+2(√2)))−(1/2)(((1−(√2))/2))∫_0 ^(tg^2 (π/8)) (du/(u+3−2(√2))) =−(1/2)ln∣1−tg^2 (π/8)∣−((1+(√2))/4)[ln∣u+3+2(√2)∣]_0 ^(tg^2 (π/8)) −((1−(√2))/4)[ln∣u+3−2(√2)∣]_0 ^(tg^2 (π/8)) =−(1/2)ln∣1−tg^2 (π/8)∣−((1+(√2))/4)ln∣tg^2 (π/8)+3+2(√2)∣−((1−(√2))/4)ln∣tg^2 (π/8)+3−2(√2)∣+((√2)/2)ln(3+2(√2))](Q171571.png) | ||
| ||