
Question and Answers Forum
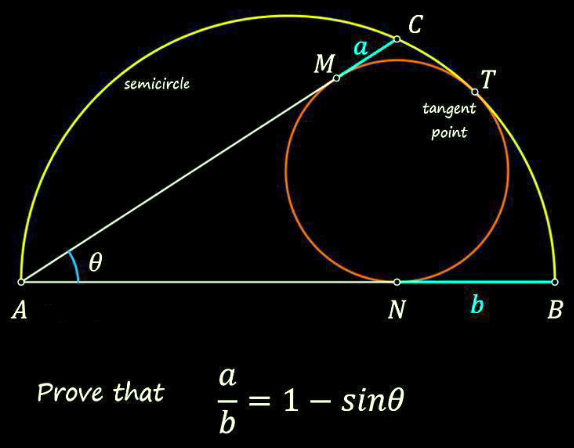
Question Number 172437 by infinityaction last updated on 26/Jun/22
Answered by mr W last updated on 27/Jun/22
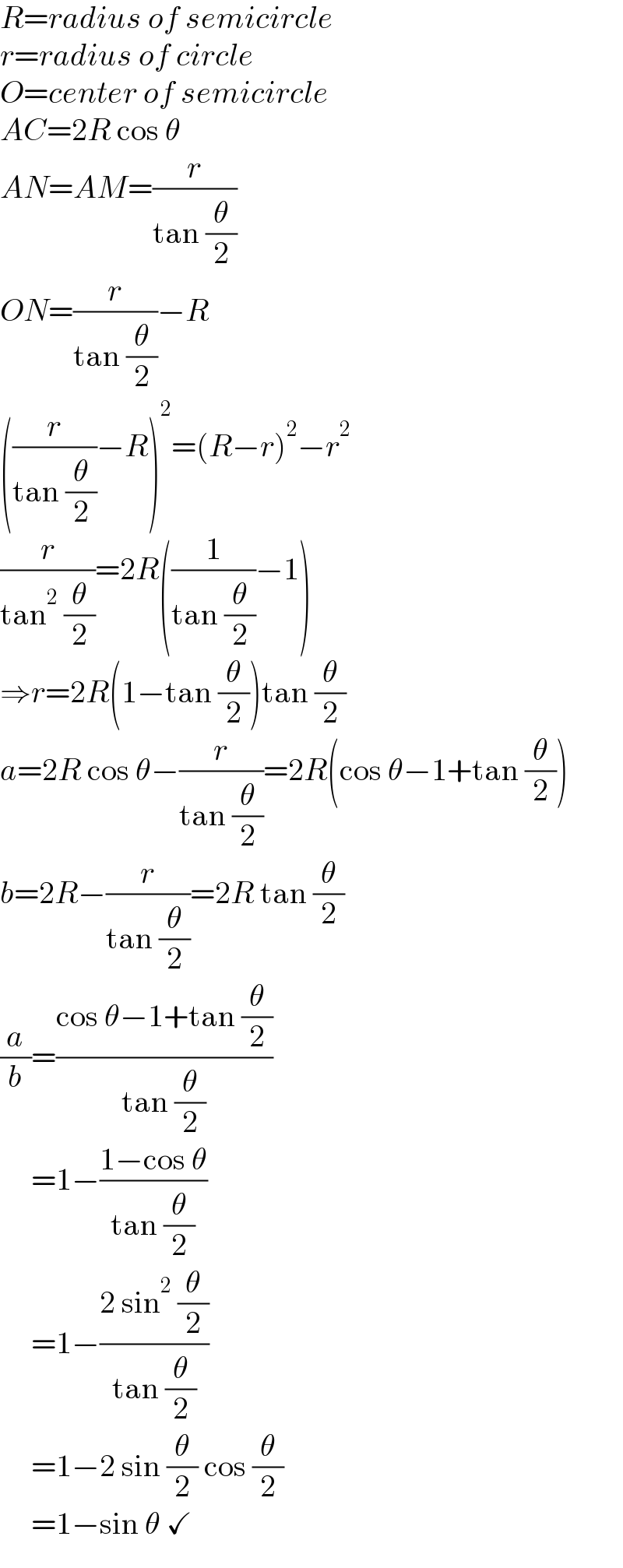
Commented by infinityaction last updated on 27/Jun/22

Commented by Tawa11 last updated on 27/Jun/22

| ||
Question and Answers Forum | ||
Question Number 172437 by infinityaction last updated on 26/Jun/22 | ||
 | ||
Answered by mr W last updated on 27/Jun/22 | ||
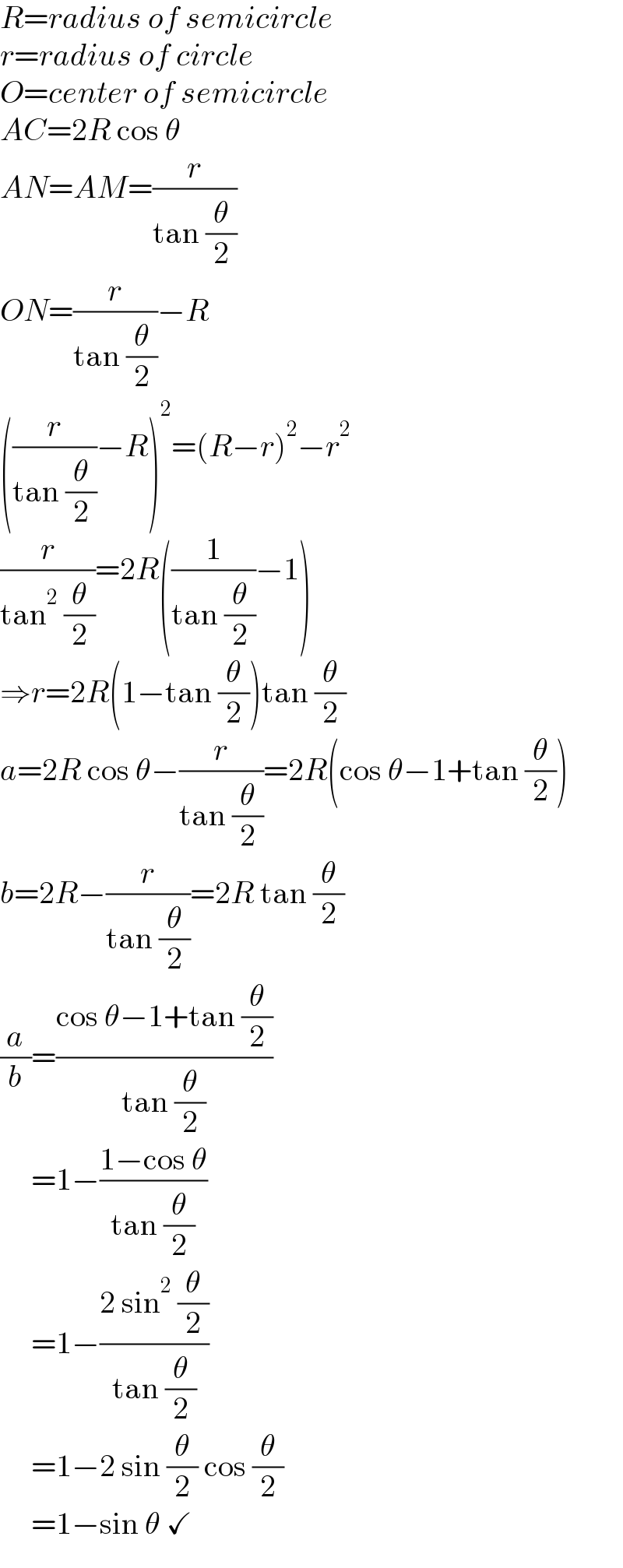 | ||
| ||
Commented by infinityaction last updated on 27/Jun/22 | ||
 | ||
Commented by Tawa11 last updated on 27/Jun/22 | ||
 | ||