Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 173485 by mnjuly1970 last updated on 12/Jul/22

$$$$$$Solve(x\in \mathbb{R})$$$$x{2}^{\frac{1}{x}}+\frac{1}{x}{2}^x=4$$$$-Source:L.Panaitopol$$

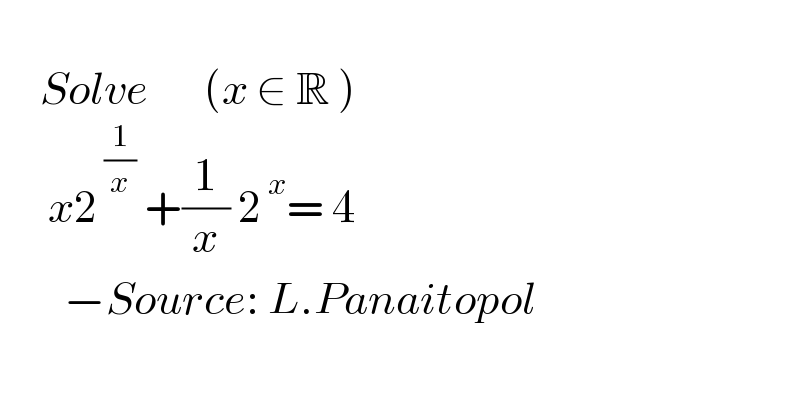
Answered by aleks041103 last updated on 12/Jul/22

$$obvx>0forx{2}^{1/x}+2^x/x=4$$$$f\left(x\right)=x{2}^{1/x}$$$$\Rightarrow g\left(x\right)=f\left(x\right)+f\left(1/x\right)$$$$\Rightarrow g\left(1/x\right)=f\left(1/x\right)+f\left(x\right)$$$$\Rightarrow g\left(x\right)=g\left(1/x\right)=4$$$$\Rightarrow thereforeitisenoughtosearch$$$$forx>1andfor\mathrm{\forall }{x}_i>1,s.t.g\left(x_i\right)=4{we}^{\prime }ll$$$$have{\overline{x}}_i=\frac{1}{x_i},s.t.g\left({\overline{x}}_i\right)=4too.$$$$2^x=e^{ln\left(2\right)x}=\underset{i=0}{\sum ^{\mathrm{\infty }}}\frac{{\left(ln\left(2\right)x\right)}^i}{i!}$$$$\Rightarrow f\left(x\right)=\underset{i=0}{\sum ^{\mathrm{\infty }}}\frac{{\left(ln\left(2\right)\right)}^i}{i!}\frac{1}{x^{i-1}}$$$$\Rightarrow f\left(1/x\right)=\underset{i=0}{\sum ^{\mathrm{\infty }}}\frac{{\left(ln\left(2\right)\right)}^i}{i!}{x}^{i-1}$$$$\Rightarrow g\left(x\right)=\underset{i=0}{\sum ^{\mathrm{\infty }}}\frac{{\left(ln\left(2\right)\right)}^i}{i!}(x^{i-1}+\frac{1}{x^{i-1}})$$$$g\left(x\right)=\underset{i=0}{\sum ^{\mathrm{\infty }}}\frac{{\left(ln\left(2\right)\right)}^i}{i!}{s}_{i-1}\left(x\right)$$$$s_i\left(x\right)=x^i+x^{-i}$$$$s_i^{\prime }\left(x\right)={ix}^{i-1}-{ix}^{-i-1}=\frac{i}{x}(x^i-x^{-i})$$$$forx>1:$$$$i>0:x^i>1\Rightarrow x^{-i}<1\Rightarrow x^i-x^{-i}>0$$$$\Rightarrow s_{i>0}^{\prime }(x>1)>0$$$$i<0:x^{i<1}\Rightarrow x^{-i}>1\Rightarrow x^i-x^{-i}<0$$$$\Rightarrow i(x^i-x^{-i})>0$$$$\Rightarrow s_{i<0}^{\prime }(x>1)>0$$$$i=0:$$$$s_0^{\prime }=0$$$$\Rightarrow g^{\prime }(x>1)=\underset{i=0,i\ne 1}{\sum ^{\mathrm{\infty }}}\frac{{\left(ln\left(2\right)\right)}^i}{i!}{s}_{i-1}^{\prime }(x>1)$$$$since{s}_{i-1}^{\prime }(x>1)>0\Rightarrow g^{\prime }(x>1)>0$$$$\Rightarrow g\left(x\right)isstrictlyincreasingforx>1$$$$\Rightarrow forg\left(x\right)=4existsatmost1soln.x⩾1$$$$Andg\left(1\right)=4$$$$\Rightarrow Theonlysolutiontotheproblemis$$$$x=1$$

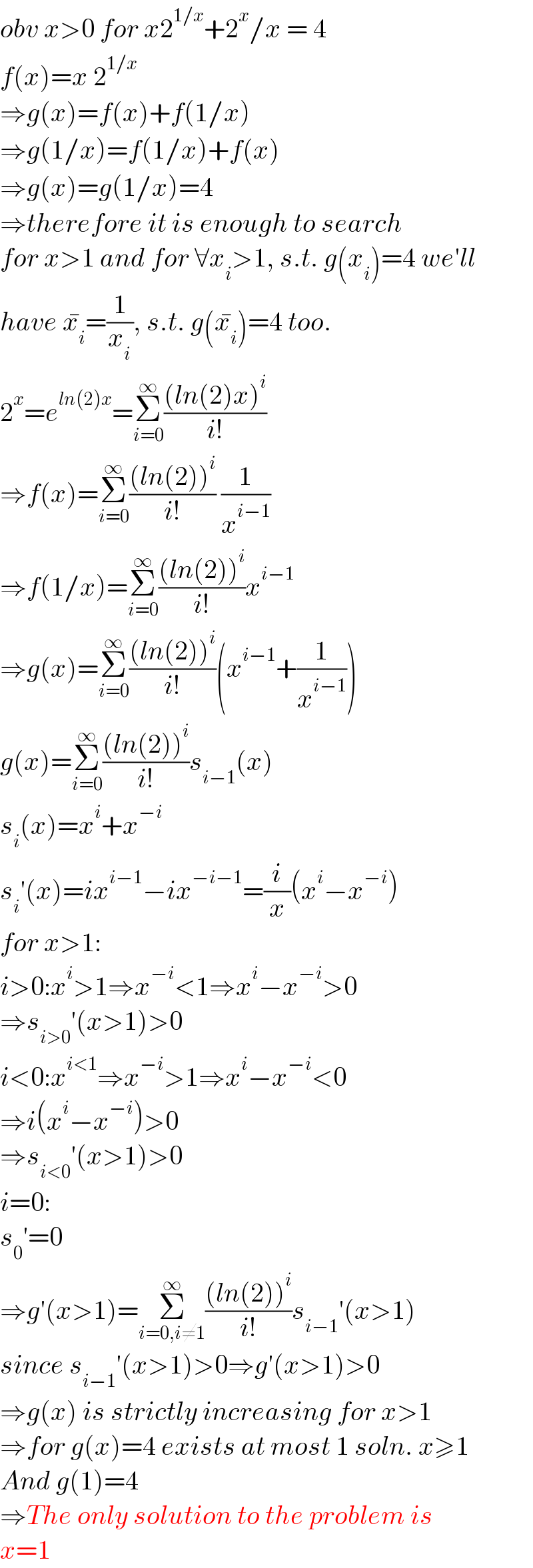
Commented by Tawa11 last updated on 13/Jul/22

$$\mathrm{Great}\mathrm{sir}$$

Answered by dragan91 last updated on 12/Jul/22

$$2^{{\log }_2\mathrm{x}+\frac{1}{\mathrm{x}}}+2^{{\log }_2\frac{1}{\mathrm{x}}+\mathrm{x}}=4$$$$2^{{\log }_2\mathrm{x}+\frac{1}{\mathrm{x}}}+2^{-{\log }_2\mathrm{x}+\mathrm{x}}=4$$$$2^{{\log }_2\mathrm{x}+\frac{1}{\mathrm{x}}}+2^{-{\log }_2\mathrm{x}+\mathrm{x}}\stackrel{\mathrm{AM}-\mathrm{GM}}{⩾}2\sqrt{2^{\mathrm{x}+\frac{1}{\mathrm{x}}}}$$$$2^{\mathrm{x}+\frac{1}{\mathrm{x}}}⩽2^2\mid {\log }_2$$$$\mathrm{x}+\frac{1}{\mathrm{x}}⩽2$$$$\mathrm{since}\mathrm{x}+\frac{1}{\mathrm{x}}\stackrel{\mathrm{AM}-\mathrm{GM}}{⩾}2\Rightarrow \mathrm{equation}\mathrm{has}\mathrm{solution}$$$$\mathrm{only}\mathrm{for}\mathrm{x}=1$$$$$$$$$$$$$$$$$$$$$$$$$$$$$$

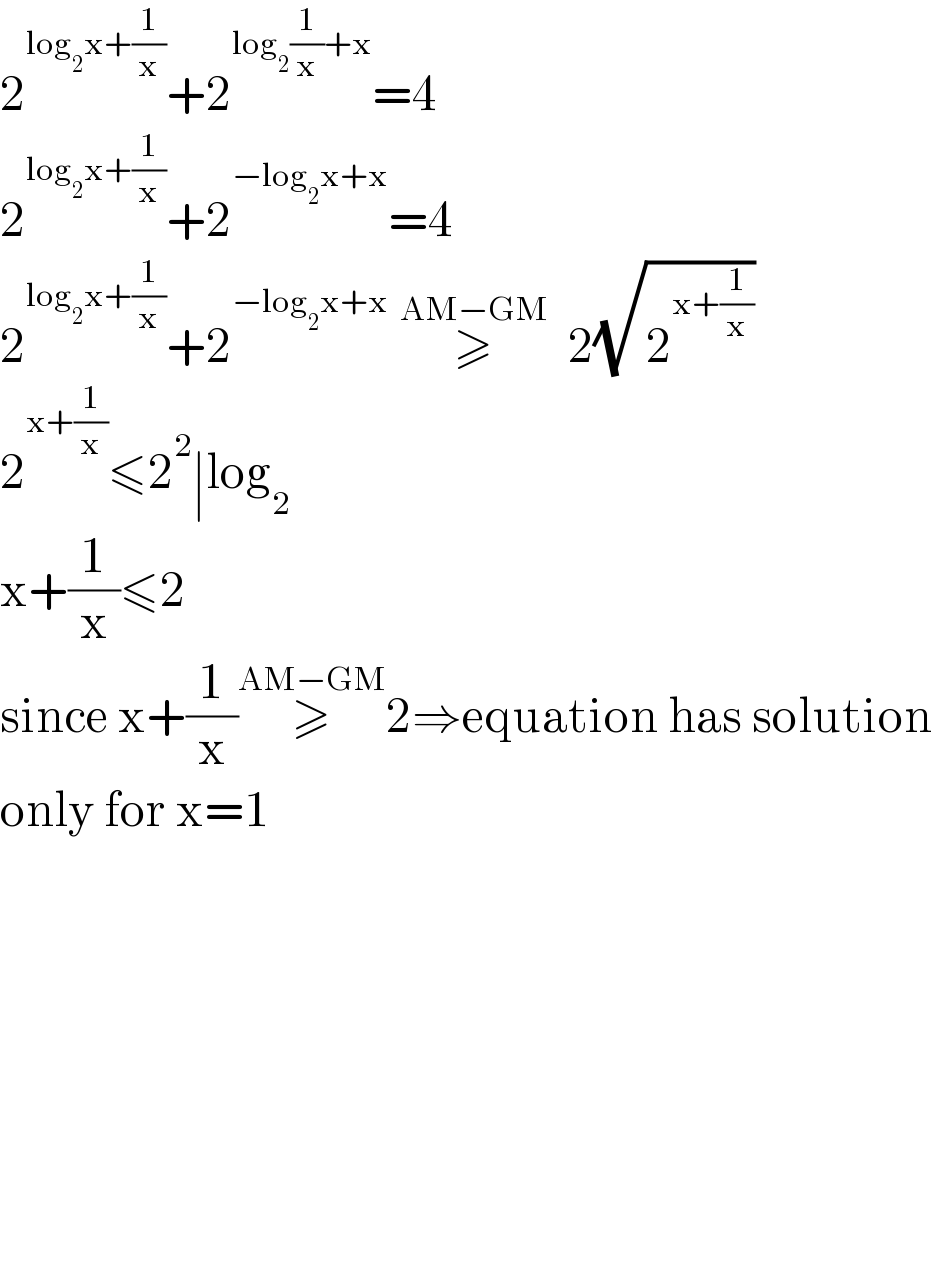
Commented by Tawa11 last updated on 13/Jul/22

$$\mathrm{Great}\mathrm{sir}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com