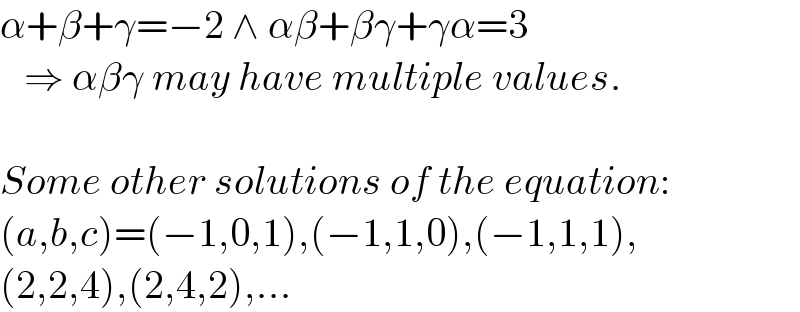Solve over integer a^3 +2a^2 +3a+4=b!+c!


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 173556 by dragan91 last updated on 13/Jul/22

$Solve over integer$ $a^{3} + {2 a}^{2} + 3 a + 4 = b! + c!$
	Answered by Rasheed.Sindhi last updated on 14/Jul/22

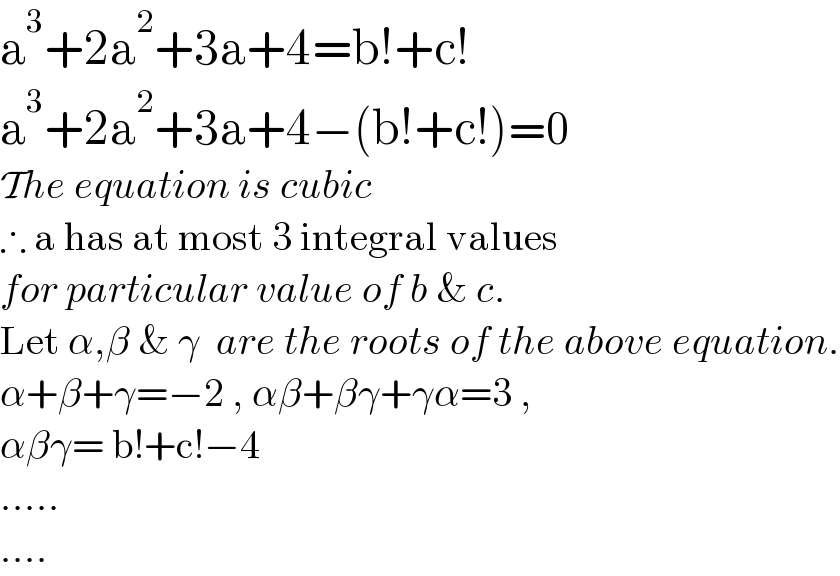
	$a^{3} + {2 a}^{2} + 3 a + 4 = b! + c!$ $a^{3} + {2 a}^{2} + 3 a + 4 - (b! + c!) = 0$ $T h e e q u a t i o n i s c u b i c$ $∴ a has at most 3 integral values$ $f o r p a r t i c u l a r v a l u e o f b & c .$ $Let α, β & γ a r e t h e r o o t s o f t h e a b o v e e q u a t i o n .$ $α + β + γ = - 2, α β + β γ + γ α = 3,$ $α β γ = b! + c! - 4$ $. . . . .$ $. . . .$
	Commented by dragan91 last updated on 16/Jul/22
	$a^3 +2a^2 +3a+4=b!+c! (a+1)(a^2 +a+2)=b!+c!−2 since b!+c!−2≥0 ∀b,c∈N ⇒(a+1)(a^2 +a+2)≥0⇒a≥−1 a^3 +2a^2 +3a+4 is never divisible by 3 then for 3≤b≤c and b!+c!=3 is no solution a^3 +2a^2 +3a+4 could have solutions for 1+c! (c≠2) or 2+c! (c≠1) We have to consider cases: I)for fixed b!=1⇒b=0=1 c≠2 a)assume a≤c⇒c!≡0(mod a) a^3 +2a^2 +3a+4=1+c! a^3 +2a^2 +3a+3=c! /:a a^2 +2a+3+^ (3/a)=((c!)/a) LHS is integer for a={(−1,1,2,3)} ⇒(a,b,c)={(−1,0,0),(−1,1,0),(−1,0,1) (−1,1,1),(2,4,2),(2,2,4)} b)assume c<a c!<a! a^3 +2a^2 +3a+3<a! its true for a≥6 for a≥6 let a=6+k (k≥0) (6+k)^3 +2(6+k)^2 +3(6+k)+3=c! k^3 +20k^2 +35k+309=c! LHS is not divisible by 2 for any inter ⇒ c=0 or c=1 ⇒k=−7 is no solution (k≥0) c)a=0 no solution II)For fixed b!=2⇒b=2 a^3 +2a^2 +3a+2=c! a)c≥a ⇒c!≡0(mod a) a^3 +2a^2 +3a+2=c!/:a a^2 +2a+3a+(2/a)=((c!)/a) LHS is integer for a={(−1,1,2)} which is already checked solutions b)c<a a^3 +2a^2 +3a+2<a! its true for a≥6⇒a=6+p p≥0 c<6+p (6+p)^3 +2(6+p)^2 +3(6+p)+2=c! p^3 +20p^2 +135p+308=c! p^3 +3p^2 ∙7+3p∙49+343−(p^2 +12p+35)=c! (p+7)^3 −(p+7)(p+5)=c! p+7=t t^3 −t(t−2)=c! Since c<p+6⇒c<t−1<t for t>1 c≢0(mod t)⇒c!≢0(mod t) t^3 −t(t−2)≡0(mod t) its only possible solution for t=1 c!=1^3 −1^2 +2⇒c=2 Its contradiction since c<t−1 c)a=0⇒c=2 (a,b,c)={(0,2,2)} All solutions (a,b,c)={(−1,0,0),(−1,0,1),(−1,1,0), (−1,1,1),(0,2,2),(2,2,4),(2,4,2)}$
	$\underset{―}{a^{3} + {2 a}^{2} + 3 a + 4 = b! + c!}$ $(a + 1) (a^{2} + a + 2) = b! + c! - 2$ $since b! + c! - 2 ⩾ 0 \forall b, c \in N$ $\Rightarrow (a + 1) (a^{2} + a + 2) ⩾ 0 \Rightarrow a ⩾ - 1$ $a^{3} + {2 a}^{2} + 3 a + 4 is never divisible by 3$ $then for 3 ⩽ b ⩽ c and b! + c! = 3$ $is no solution$ $a^{3} + {2 a}^{2} + 3 a + 4 could have solutions$ $for 1 + c! (c \neq 2) or 2 + c! (c \neq 1)$ $We have to consider cases :$ $I) for fixed b! = 1 \Rightarrow b = 0 = 1 c \neq 2$ $a) assume a ⩽ c \Rightarrow c! \equiv 0 (\mod a)$ $a^{3} + {2 a}^{2} + 3 a + 4 = 1 + c!$ $a^{3} + {2 a}^{2} + 3 a + 3 = c! / : a$ $a^{2} + 2 a + 3 +^{} \frac{3}{a} = \frac{c!}{a}$ $LHS is integer for a = {(- 1, 1, 2, 3)}$ $\Rightarrow (a, b, c) = {(- 1, 0, 0), (- 1, 1, 0), (- 1, 0, 1)$ $(- 1, 1, 1), (2, 4, 2), (2, 2, 4)}$ $b) assume c < a$ $c! < a!$ $a^{3} + {2 a}^{2} + 3 a + 3 < a!$ $its true for a ⩾ 6$ $for a ⩾ 6 let a = 6 + k (k ⩾ 0)$ ${(6 + k)}^{3} + 2 {(6 + k)}^{2} + 3 (6 + k) + 3 = c!$ $k^{3} + {20 k}^{2} + 35 k + 309 = c!$ $LHS is not divisible by 2$ $for any inter \Rightarrow c = 0 or c = 1$ $\Rightarrow k = - 7 is no solution (k ⩾ 0)$ $c) a = 0 no solution$ $II) For fixed b! = 2 \Rightarrow b = 2$ $a^{3} + {2 a}^{2} + 3 a + 2 = c!$ $a) c ⩾ a \Rightarrow c! \equiv 0 (\mod a)$ $a^{3} + {2 a}^{2} + 3 a + 2 = c! / : a$ $a^{2} + 2 a + 3 a + \frac{2}{a} = \frac{c!}{a}$ $LHS is integer for a = {(- 1, 1, 2)} which is$ $already checked solutions$ $b) c < a$ $a^{3} + {2 a}^{2} + 3 a + 2 < a!$ $its true for a ⩾ 6 \Rightarrow a = 6 + p p ⩾ 0$ $c < 6 + p$ ${(6 + p)}^{3} + 2 {(6 + p)}^{2} + 3 (6 + p) + 2 = c!$ $p^{3} + {20 p}^{2} + 135 p + 308 = c!$ $p^{3} + {3 p}^{2} \cdot 7 + 3 p \cdot 49 + 343 - (p^{2} + 12 p + 35) = c!$ ${(p + 7)}^{3} - (p + 7) (p + 5) = c!$ $p + 7 = t$ $t^{3} - t (t - 2) = c!$ $Since c < p + 6 \Rightarrow c < t - 1 < t$ $for t > 1 c ≢ 0 (\mod t) \Rightarrow c! ≢ 0 (\mod t)$ $t^{3} - t (t - 2) \equiv 0 (\mod t)$ $its only possible solution for t = 1$ $c! = 1^{3} - 1^{2} + 2 \Rightarrow c = 2$ $Its contradiction since c < t - 1$ $c) a = 0 \Rightarrow c = 2$ $(a, b, c) = {(0, 2, 2)}$ $\underset{―}{}$ $All solutions$ $(a, b, c) = {(- 1, 0, 0), (- 1, 0, 1), (- 1, 1, 0),$ $(- 1, 1, 1), (0, 2, 2), (2, 2, 4), (2, 4, 2)}$
	Commented by dragan91 last updated on 15/Jul/22

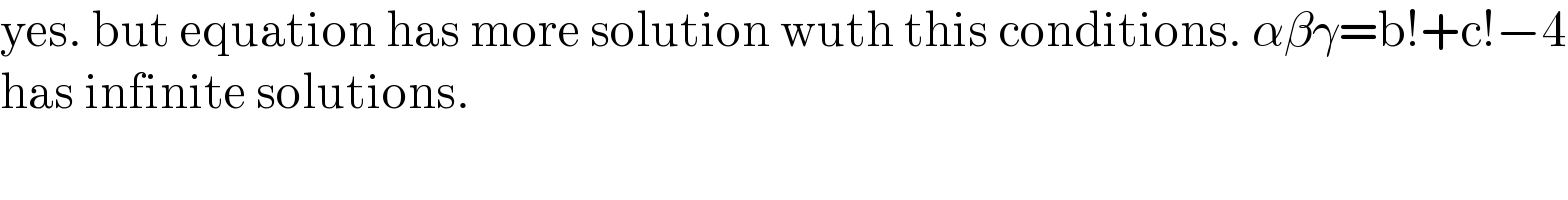
	$yes . but equation has more solution wuth this conditions . α β γ = b! + c! - 4$ $has infinite solutions .$
	Commented by Rasheed.Sindhi last updated on 16/Jul/22

	$α + β + γ = - 2 \land α β + β γ + γ α = 3$ $\Rightarrow α β γ m a y h a v e m u l t i p l e v a l u e s .$ $S o m e o t h e r s o l u t i o n s o f t h e e q u a t i o n :$ $(a, b, c) = (- 1, 0, 1), (- 1, 1, 0), (- 1, 1, 1),$ $(2, 2, 4), (2, 4, 2), . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum