Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 175049 by mnjuly1970 last updated on 17/Aug/22

Z_( p) , is a field ...( p is prime )

$$ \\ $$$$\:\:\:\:\:\:\mathbb{Z}_{\:{p}} \:,\:{is}\:\:{a}\:\:{field}\:...\left(\:\:{p}\:{is}\:{prime}\:\right) \\ $$$$\:\:\:\:\:\: \\ $$

Commented by kaivan.ahmadi last updated on 17/Aug/22

we show that every element of Z_p has inverse. let [a]∈Z_p ,0<a<p since (a,p)=1⇒∃r,s∈Z s.t ar+ps=1⇒ [a].[r]=[ar]+[0]=[ar]+[ps]= [ar+ps]=[1]⇒[a]^(−1) =[r].■

$${we}\:{show}\:{that}\:{every}\:{element} \\ $$$${of}\:\mathbb{Z}_{{p}} \:{has}\:{inverse}. \\ $$$${let}\:\left[{a}\right]\in\mathbb{Z}_{{p}} \:,\mathrm{0}<{a}<{p} \\ $$$${since}\:\left({a},{p}\right)=\mathrm{1}\Rightarrow\exists{r},{s}\in\mathbb{Z}\:{s}.{t} \\ $$$${ar}+{ps}=\mathrm{1}\Rightarrow \\ $$$$\left[{a}\right].\left[{r}\right]=\left[{ar}\right]+\left[\mathrm{0}\right]=\left[{ar}\right]+\left[{ps}\right]= \\ $$$$\left[{ar}+{ps}\right]=\left[\mathrm{1}\right]\Rightarrow\left[{a}\right]^{−\mathrm{1}} =\left[{r}\right].\blacksquare \\ $$

Commented by mnjuly1970 last updated on 17/Aug/22

thanks alot

$${thanks}\:{alot} \\ $$

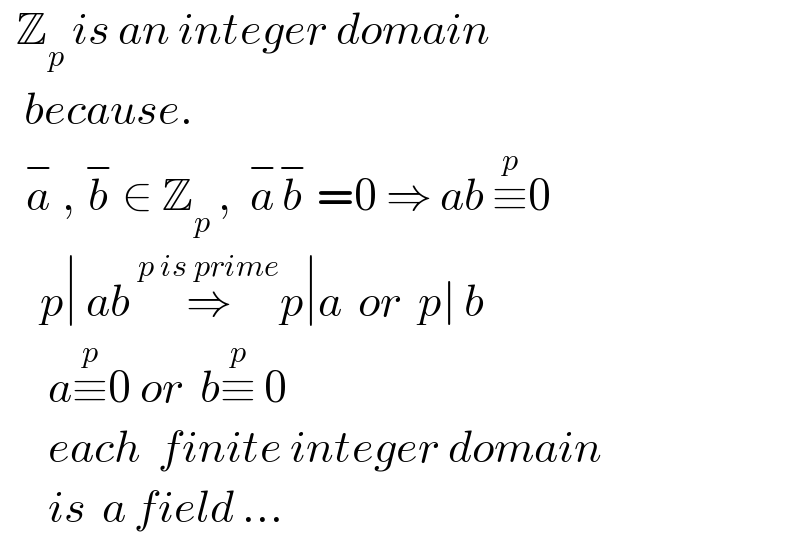
Answered by mnjuly1970 last updated on 17/Aug/22

Z_p is an integer domain because. a^− , b^− ∈ Z_p , a^− b^− =0 ⇒ a^ b ≡^p 0 p∣ ab ⇒^(p is prime) p∣a or p∣ b a≡^p 0 or b≡^p 0 each finite integer domain is a field ...

$$\:\:\mathbb{Z}_{{p}} \:{is}\:{an}\:{integer}\:{domain}\: \\ $$$$\:\:\:{because}. \\ $$$$\:\:\:\overset{−} {{a}}\:,\:\overset{−} {{b}}\:\in\:\mathbb{Z}_{{p}} \:,\:\:\overset{−} {{a}}\overset{−} {{b}}\:=\mathrm{0}\:\Rightarrow\:\overset{\:} {{a}b}\:\overset{{p}} {\equiv}\mathrm{0} \\ $$$$\:\:\:\:\:{p}\mid\:{ab}\:\overset{{p}\:{is}\:{prime}} {\Rightarrow}{p}\mid{a}\:\:{or}\:\:{p}\mid\:{b}\:\: \\ $$$$\:\:\:\:\:\:{a}\overset{{p}} {\equiv}\mathrm{0}\:{or}\:\:{b}\overset{{p}} {\equiv}\:\mathrm{0}\:\: \\ $$$$\:\:\:\:\:\:{each}\:\:{finite}\:{integer}\:{domain} \\ $$$$\:\:\:\:\:\:{is}\:\:{a}\:{field}\:... \\ $$

Commented by kaivan.ahmadi last updated on 19/Aug/22

ok its true. but do you know why each finite integer domain is a field? because every element in it has an inverse. let R={0,1,a_1 ,...,a_n } so a_i R=R⇒∃a_j ≠a_i s.t a_i a_j =1⇒a_i ^(−1) =a_j (or a_i =1).

$${ok}\:{its}\:{true}.\:{but}\:{do}\:{you}\:{know} \\ $$$${why}\:{each}\:{finite}\:{integer}\:{domain} \\ $$$${is}\:{a}\:{field}?\:{because}\:{every}\:{element} \\ $$$${in}\:{it}\:{has}\:{an}\:{inverse}. \\ $$$${let}\:{R}=\left\{\mathrm{0},\mathrm{1},{a}_{\mathrm{1}} ,...,{a}_{{n}} \right\} \\ $$$${so}\:{a}_{{i}} {R}={R}\Rightarrow\exists{a}_{{j}} \neq{a}_{{i}} \:{s}.{t} \\ $$$${a}_{{i}} {a}_{{j}} =\mathrm{1}\Rightarrow{a}_{{i}} ^{−\mathrm{1}} ={a}_{{j}} \:\left({or}\:{a}_{{i}} =\mathrm{1}\right). \\ $$

Commented by mnjuly1970 last updated on 17/Aug/22

thx sir

$$\:\:\:\:{thx}\:{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com