Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 175801 by Lekhraj last updated on 07/Sep/22

solve the follwing equation x(√(x )) + y(√(y )) = 3 and x(√(y )) + y(√(x )) = 2 someone solve the above equations in the following way x^3 + y^3 + 2xy(√(xy )) = 9.....(1) and x^2 y + y^2 x + 2xy(√(xy )) = 4......(2) (1) − (2) ⇒ (x − y)(x^2 − y^2 ) = 5 hence x = 3 and y = 2 which is obiviusly does not satisfy the original equations. where is the fallacy in the above solution? Please explain.

$${solve}\:{the}\:{follwing}\:{equation} \\ $$$${x}\sqrt{{x}\:}\:\:\:+\:\:{y}\sqrt{{y}\:}\:\:=\:\:\mathrm{3}\:\:\:{and}\:\:\:{x}\sqrt{{y}\:}\:\:+\:{y}\sqrt{{x}\:}\:\:=\:\:\mathrm{2} \\ $$$${someone}\:{solve}\:{the}\:{above}\:{equations}\:{in}\:{the}\:{following}\:{way}\: \\ $$$${x}^{\mathrm{3}} +\:{y}^{\mathrm{3}} +\:\mathrm{2}{xy}\sqrt{{xy}\:}\:\:=\:\mathrm{9}.....\left(\mathrm{1}\right)\:\:\:\:{and}\:\:\:{x}^{\mathrm{2}} {y}\:\:+\:\:{y}^{\mathrm{2}} {x}\:\:+\:\mathrm{2}{xy}\sqrt{{xy}\:}\:\:=\:\:\mathrm{4}......\left(\mathrm{2}\right) \\ $$$$\left(\mathrm{1}\right)\:−\:\left(\mathrm{2}\right)\:\:\:\Rightarrow\:\:\left({x}\:−\:{y}\right)\left({x}^{\mathrm{2}} \:−\:{y}^{\mathrm{2}} \:\right)\:=\:\mathrm{5} \\ $$$${hence}\:\:{x}\:=\:\mathrm{3}\:\:{and}\:\:{y}\:=\:\mathrm{2}\:\:{which}\:{is}\:{obiviusly}\:{does}\:{not}\:\:{satisfy}\:{the} \\ $$$$\:{original}\:{equations}. \\ $$$${where}\:{is}\:{the}\:{fallacy}\:\:{in}\:{the}\:{above}\:{solution}?\:\:\mathrm{Please}\:\mathrm{explain}. \\ $$

Commented by mr W last updated on 07/Sep/22

(x − y)(x^2 − y^2 ) = 5 has infinitesolutions! not every solutionfrom it fulfills the original equations. x=3 and y=2 is only one possible solution of (x−y)(x^2 −y^2 )=5.

$$\left({x}\:−\:{y}\right)\left({x}^{\mathrm{2}} \:−\:{y}^{\mathrm{2}} \:\right)\:=\:\mathrm{5} \\ $$$${has}\:{infinitesolutions}!\:{not}\:{every} \\ $$$${solutionfrom}\:{it}\:{fulfills}\:{the}\:{original} \\ $$$${equations}.\:{x}=\mathrm{3}\:{and}\:{y}=\mathrm{2}\:{is}\:{only}\:{one} \\ $$$${possible}\:{solution}\:{of}\:\left({x}−{y}\right)\left({x}^{\mathrm{2}} −{y}^{\mathrm{2}} \:\right)=\mathrm{5}. \\ $$

Commented by Frix last updated on 07/Sep/22

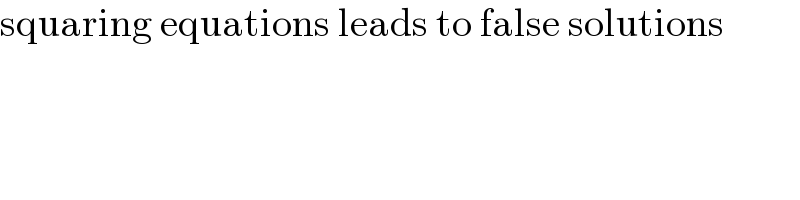
squaring equations leads to false solutions

$$\mathrm{squaring}\:\mathrm{equations}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{false}\:\mathrm{solutions} \\ $$

Commented by Lekhraj last updated on 08/Sep/22

Thank you.

$$\mathrm{Thank}\:\mathrm{you}. \\ $$

Commented by Lekhraj last updated on 08/Sep/22

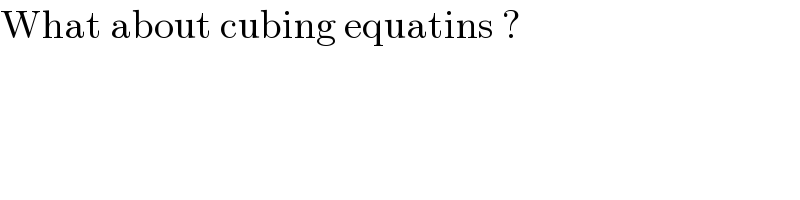
What about cubing equatins ?

$$\mathrm{What}\:\mathrm{about}\:\mathrm{cubing}\:\mathrm{equatins}\:? \\ $$

Commented by Frix last updated on 16/Sep/22

cubing is also dangerous for complex numbers

$$\mathrm{cubing}\:\mathrm{is}\:\mathrm{also}\:\mathrm{dangerous}\:\mathrm{for}\:\mathrm{complex}\:\mathrm{numbers} \\ $$

Answered by behi834171 last updated on 07/Sep/22

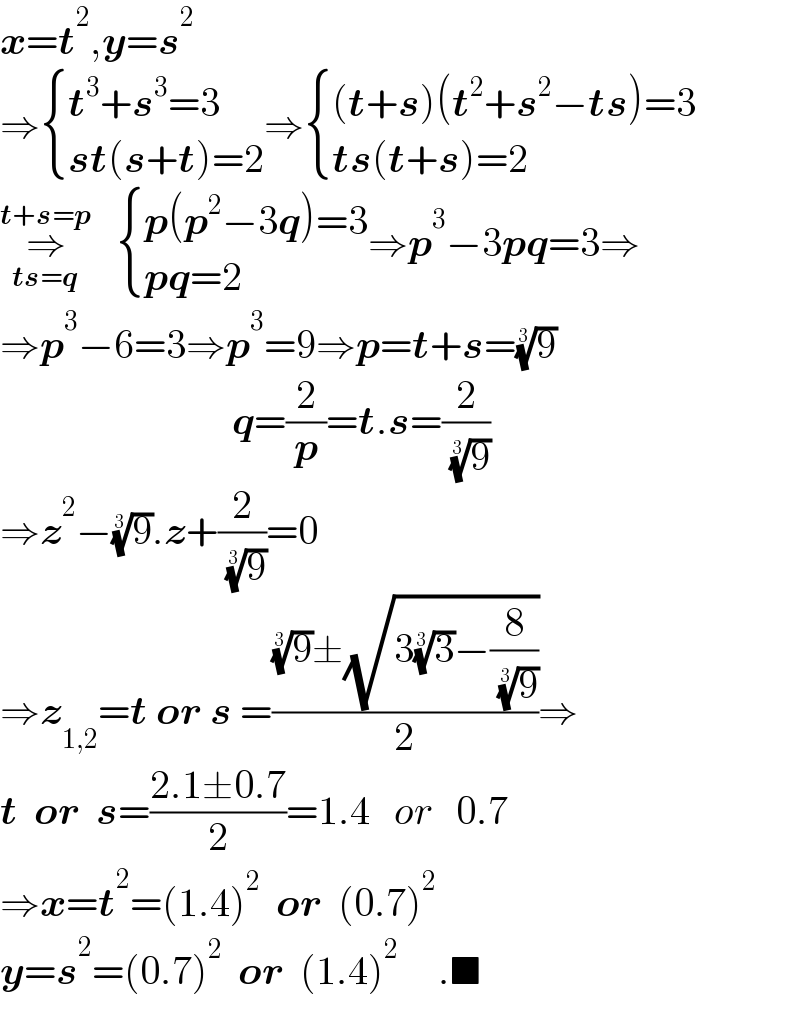
x=t^2 ,y=s^2 ⇒ { ((t^3 +s^3 =3)),((st(s+t)=2)) :}⇒ { (((t+s)(t^2 +s^2 −ts)=3)),((ts(t+s)=2)) :} ⇒_(ts=q) ^(t+s=p) { ((p(p^2 −3q)=3)),((pq=2)) :}⇒p^3 −3pq=3⇒ ⇒p^3 −6=3⇒p^3 =9⇒p=t+s=(9)^(1/3) q=(2/p)=t.s=(2/( (9)^(1/3) )) ⇒z^2 −(9)^(1/3) .z+(2/( (9)^(1/3) ))=0 ⇒z_(1,2) =t or s =(((9)^(1/3) ±(√(3(3)^(1/3) −(8/( (9)^(1/3) )))))/2)⇒ t or s=((2.1±0.7)/2)=1.4 or 0.7 ⇒x=t^2 =(1.4)^2 or (0.7)^2 y=s^2 =(0.7)^2 or (1.4)^2 .■

$$\boldsymbol{{x}}=\boldsymbol{{t}}^{\mathrm{2}} ,\boldsymbol{{y}}=\boldsymbol{{s}}^{\mathrm{2}} \\ $$$$\Rightarrow\begin{cases}{\boldsymbol{{t}}^{\mathrm{3}} +\boldsymbol{{s}}^{\mathrm{3}} =\mathrm{3}}\\{\boldsymbol{{st}}\left(\boldsymbol{{s}}+\boldsymbol{{t}}\right)=\mathrm{2}}\end{cases}\Rightarrow\begin{cases}{\left(\boldsymbol{{t}}+\boldsymbol{{s}}\right)\left(\boldsymbol{{t}}^{\mathrm{2}} +\boldsymbol{{s}}^{\mathrm{2}} −\boldsymbol{{ts}}\right)=\mathrm{3}}\\{\boldsymbol{{ts}}\left(\boldsymbol{{t}}+\boldsymbol{{s}}\right)=\mathrm{2}}\end{cases} \\ $$$$\underset{\boldsymbol{{ts}}=\boldsymbol{{q}}} {\overset{\boldsymbol{{t}}+\boldsymbol{{s}}=\boldsymbol{{p}}} {\Rightarrow}}\:\:\:\begin{cases}{\boldsymbol{{p}}\left(\boldsymbol{{p}}^{\mathrm{2}} −\mathrm{3}\boldsymbol{{q}}\right)=\mathrm{3}}\\{\boldsymbol{{pq}}=\mathrm{2}}\end{cases}\Rightarrow\boldsymbol{{p}}^{\mathrm{3}} −\mathrm{3}\boldsymbol{{pq}}=\mathrm{3}\Rightarrow \\ $$$$\Rightarrow\boldsymbol{{p}}^{\mathrm{3}} −\mathrm{6}=\mathrm{3}\Rightarrow\boldsymbol{{p}}^{\mathrm{3}} =\mathrm{9}\Rightarrow\boldsymbol{{p}}=\boldsymbol{{t}}+\boldsymbol{{s}}=\sqrt[{\mathrm{3}}]{\mathrm{9}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{q}}=\frac{\mathrm{2}}{\boldsymbol{{p}}}=\boldsymbol{{t}}.\boldsymbol{{s}}=\frac{\mathrm{2}}{\:\sqrt[{\mathrm{3}}]{\mathrm{9}}} \\ $$$$\Rightarrow\boldsymbol{{z}}^{\mathrm{2}} −\sqrt[{\mathrm{3}}]{\mathrm{9}}.\boldsymbol{{z}}+\frac{\mathrm{2}}{\:\sqrt[{\mathrm{3}}]{\mathrm{9}}}=\mathrm{0} \\ $$$$\Rightarrow\boldsymbol{{z}}_{\mathrm{1},\mathrm{2}} =\boldsymbol{{t}}\:\boldsymbol{{or}}\:\boldsymbol{{s}}\:=\frac{\sqrt[{\mathrm{3}}]{\mathrm{9}}\pm\sqrt{\mathrm{3}\sqrt[{\mathrm{3}}]{\mathrm{3}}−\frac{\mathrm{8}}{\:\sqrt[{\mathrm{3}}]{\mathrm{9}}}}}{\mathrm{2}}\Rightarrow \\ $$$$\boldsymbol{{t}}\:\:\boldsymbol{{or}}\:\:\boldsymbol{{s}}=\frac{\mathrm{2}.\mathrm{1}\pm\mathrm{0}.\mathrm{7}}{\mathrm{2}}=\mathrm{1}.\mathrm{4}\:\:\:{or}\:\:\:\mathrm{0}.\mathrm{7} \\ $$$$\Rightarrow\boldsymbol{{x}}=\boldsymbol{{t}}^{\mathrm{2}} =\left(\mathrm{1}.\mathrm{4}\right)^{\mathrm{2}} \:\:\boldsymbol{{or}}\:\:\left(\mathrm{0}.\mathrm{7}\right)^{\mathrm{2}} \\ $$$$\boldsymbol{{y}}=\boldsymbol{{s}}^{\mathrm{2}} =\left(\mathrm{0}.\mathrm{7}\right)^{\mathrm{2}} \:\:\boldsymbol{{or}}\:\:\left(\mathrm{1}.\mathrm{4}\right)^{\mathrm{2}} \:\:\:\:\:.\blacksquare \\ $$

Answered by Frix last updated on 07/Sep/22

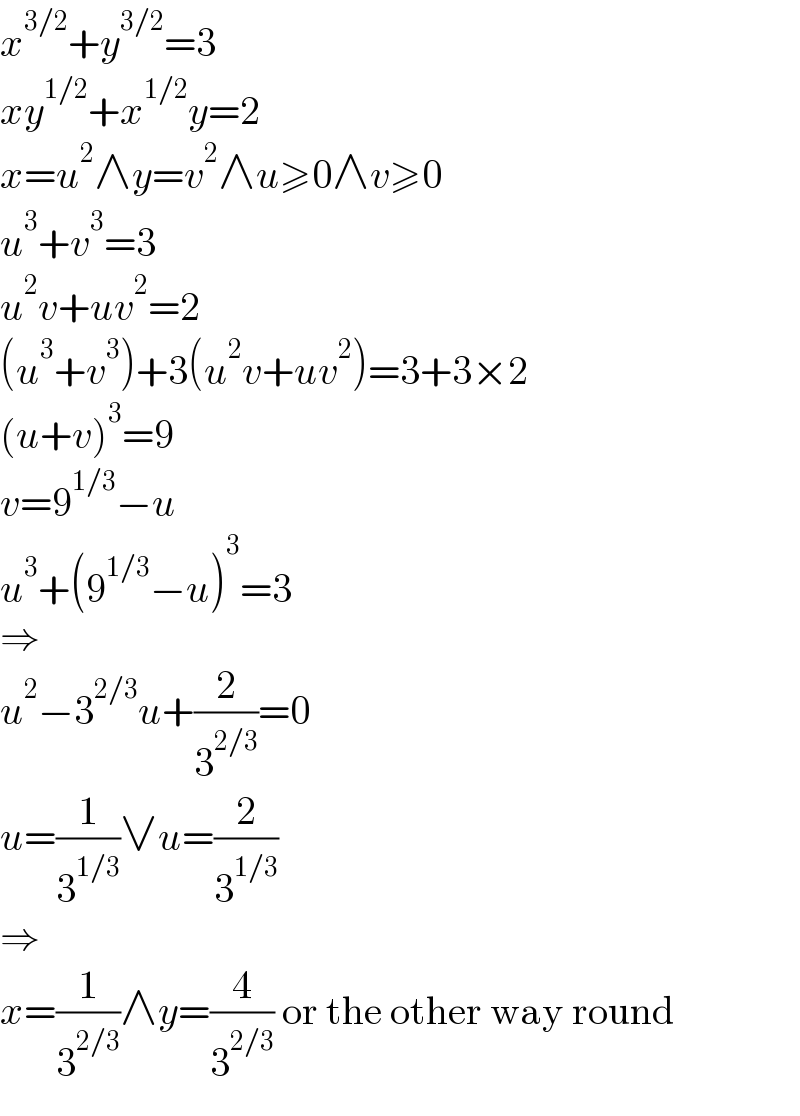
x^(3/2) +y^(3/2) =3 xy^(1/2) +x^(1/2) y=2 x=u^2 ∧y=v^2 ∧u≥0∧v≥0 u^3 +v^3 =3 u^2 v+uv^2 =2 (u^3 +v^3 )+3(u^2 v+uv^2 )=3+3×2 (u+v)^3 =9 v=9^(1/3) −u u^3 +(9^(1/3) −u)^3 =3 ⇒ u^2 −3^(2/3) u+(2/3^(2/3) )=0 u=(1/3^(1/3) )∨u=(2/3^(1/3) ) ⇒ x=(1/3^(2/3) )∧y=(4/3^(2/3) ) or the other way round

$${x}^{\mathrm{3}/\mathrm{2}} +{y}^{\mathrm{3}/\mathrm{2}} =\mathrm{3} \\ $$$${xy}^{\mathrm{1}/\mathrm{2}} +{x}^{\mathrm{1}/\mathrm{2}} {y}=\mathrm{2} \\ $$$${x}={u}^{\mathrm{2}} \wedge{y}={v}^{\mathrm{2}} \wedge{u}\geqslant\mathrm{0}\wedge{v}\geqslant\mathrm{0} \\ $$$${u}^{\mathrm{3}} +{v}^{\mathrm{3}} =\mathrm{3} \\ $$$${u}^{\mathrm{2}} {v}+{uv}^{\mathrm{2}} =\mathrm{2} \\ $$$$\left({u}^{\mathrm{3}} +{v}^{\mathrm{3}} \right)+\mathrm{3}\left({u}^{\mathrm{2}} {v}+{uv}^{\mathrm{2}} \right)=\mathrm{3}+\mathrm{3}×\mathrm{2} \\ $$$$\left({u}+{v}\right)^{\mathrm{3}} =\mathrm{9} \\ $$$${v}=\mathrm{9}^{\mathrm{1}/\mathrm{3}} −{u} \\ $$$${u}^{\mathrm{3}} +\left(\mathrm{9}^{\mathrm{1}/\mathrm{3}} −{u}\right)^{\mathrm{3}} =\mathrm{3} \\ $$$$\Rightarrow \\ $$$${u}^{\mathrm{2}} −\mathrm{3}^{\mathrm{2}/\mathrm{3}} {u}+\frac{\mathrm{2}}{\mathrm{3}^{\mathrm{2}/\mathrm{3}} }=\mathrm{0} \\ $$$${u}=\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{1}/\mathrm{3}} }\vee{u}=\frac{\mathrm{2}}{\mathrm{3}^{\mathrm{1}/\mathrm{3}} } \\ $$$$\Rightarrow \\ $$$${x}=\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}/\mathrm{3}} }\wedge{y}=\frac{\mathrm{4}}{\mathrm{3}^{\mathrm{2}/\mathrm{3}} }\:\mathrm{or}\:\mathrm{the}\:\mathrm{other}\:\mathrm{way}\:\mathrm{round} \\ $$

Commented by Lekhraj last updated on 08/Sep/22

Commented by Lekhraj last updated on 08/Sep/22

Commented by Lekhraj last updated on 08/Sep/22

Thank you very much .

$$\boldsymbol{\mathrm{Thank}}\:\boldsymbol{\mathrm{you}}\:\boldsymbol{\mathrm{very}}\:\boldsymbol{\mathrm{much}}\:. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com