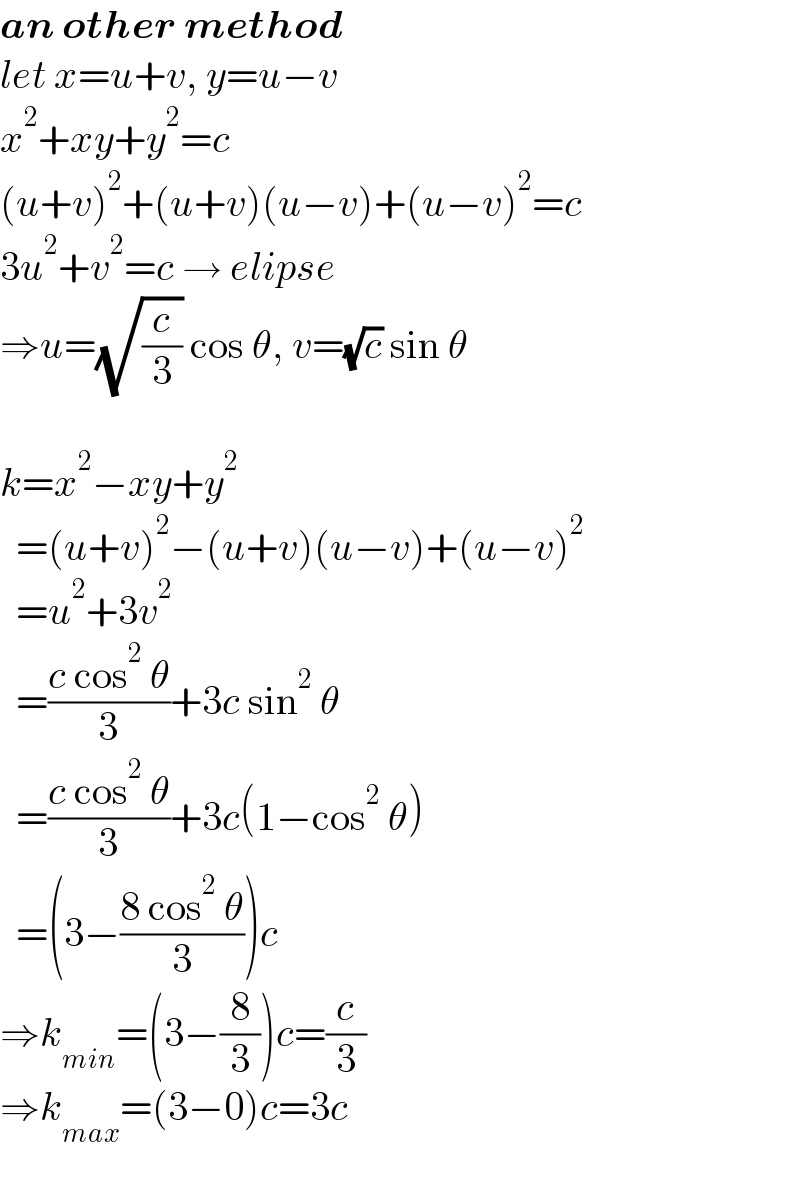Question Number 177130 by mr W last updated on 01/Oct/22
$${if}\:{x}^{\mathrm{2}} +{xy}+{y}^{\mathrm{2}} =\mathrm{5},\:{find}\:{the}\:{minimum} \\ $$$${and}\:{maximum}\:{of}\:{x}^{\mathrm{2}} −{xy}+{y}^{\mathrm{2}} . \\ $$
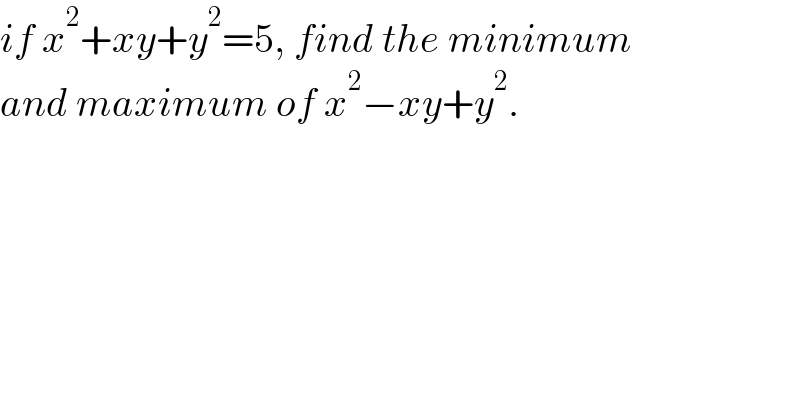
Commented by Frix last updated on 01/Oct/22
$$\mathrm{I}\:\mathrm{think} \\ $$$$\frac{\mathrm{5}}{\mathrm{3}}\leqslant{x}^{\mathrm{2}} −{xy}+{y}^{\mathrm{2}} \leqslant\mathrm{15} \\ $$
Commented by Frix last updated on 01/Oct/22
$${x}^{\mathrm{2}} \pm{xy}+{y}^{\mathrm{2}} ={c} \\ $$$$\Rightarrow \\ $$$$\frac{{c}}{\mathrm{3}}\leqslant{x}^{\mathrm{2}} \mp{xy}+{y}^{\mathrm{2}} \leqslant\mathrm{3}{c} \\ $$
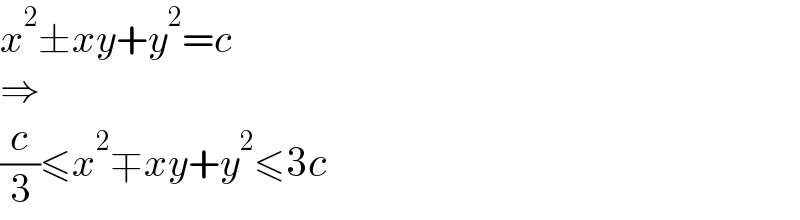
Commented by mr W last updated on 01/Oct/22
$${thanks}\:{sir}! \\ $$
Commented by Frix last updated on 01/Oct/22
$$\mathrm{you}\:\mathrm{are}\:\mathrm{right},\:\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{what}\:\mathrm{I}\:\mathrm{did}\:\mathrm{before}... \\ $$
Answered by mr W last updated on 01/Oct/22
![x^2 +xy+y^2 =c=5 ...(i) let x^2 −xy+y^2 =k ...(ii) [(i)−(ii)]/2: ⇒xy=((c−k)/2) ⇒y=((c−k)/(2x)) x^2 +((c−k)/2)+(((c−k)/(2x)))^2 =c x^4 −(((c+k)/2))x^2 +(((c−k)/2))^2 =0 Δ=(((c+k)/2))^2 −4(((c−k)/2))^2 ≥0 (k−3c)(3k−c)≤0 ⇒(c/3)≤k≤3c i.e. (c/3)≤ x^2 −xy+y^2 ≤3c with c=5: minimum=(5/3) maximum=3×5=15](Q177168.png)
$${x}^{\mathrm{2}} +{xy}+{y}^{\mathrm{2}} ={c}=\mathrm{5}\:\:\:...\left({i}\right) \\ $$$${let}\:{x}^{\mathrm{2}} −{xy}+{y}^{\mathrm{2}} ={k}\:\:\:...\left({ii}\right) \\ $$$$\left[\left({i}\right)−\left({ii}\right)\right]/\mathrm{2}: \\ $$$$\Rightarrow{xy}=\frac{{c}−{k}}{\mathrm{2}}\:\Rightarrow{y}=\frac{{c}−{k}}{\mathrm{2}{x}} \\ $$$${x}^{\mathrm{2}} +\frac{{c}−{k}}{\mathrm{2}}+\left(\frac{{c}−{k}}{\mathrm{2}{x}}\right)^{\mathrm{2}} ={c} \\ $$$${x}^{\mathrm{4}} −\left(\frac{{c}+{k}}{\mathrm{2}}\right){x}^{\mathrm{2}} +\left(\frac{{c}−{k}}{\mathrm{2}}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\Delta=\left(\frac{{c}+{k}}{\mathrm{2}}\right)^{\mathrm{2}} −\mathrm{4}\left(\frac{{c}−{k}}{\mathrm{2}}\right)^{\mathrm{2}} \geqslant\mathrm{0} \\ $$$$\left({k}−\mathrm{3}{c}\right)\left(\mathrm{3}{k}−{c}\right)\leqslant\mathrm{0} \\ $$$$\Rightarrow\frac{{c}}{\mathrm{3}}\leqslant{k}\leqslant\mathrm{3}{c} \\ $$$${i}.{e}.\:\frac{{c}}{\mathrm{3}}\leqslant\:{x}^{\mathrm{2}} −{xy}+{y}^{\mathrm{2}} \leqslant\mathrm{3}{c} \\ $$$${with}\:{c}=\mathrm{5}: \\ $$$${minimum}=\frac{\mathrm{5}}{\mathrm{3}} \\ $$$${maximum}=\mathrm{3}×\mathrm{5}=\mathrm{15} \\ $$
Commented by Strengthenchen last updated on 06/Oct/22
$${how}\:{can}\:{i}\:{to}\:{know}\:{use}\:{this}\:\:{way}\:{to}\:{get}\:\:{correcting}\:{answer}\:{if}\:{i}\:{never}\:{seem}\:{this}\:{form}\:{question}\:{before}? \\ $$
Answered by mr W last updated on 02/Oct/22
$$\boldsymbol{{an}}\:\boldsymbol{{other}}\:\boldsymbol{{method}} \\ $$$${let}\:{x}={u}+{v},\:{y}={u}−{v} \\ $$$${x}^{\mathrm{2}} +{xy}+{y}^{\mathrm{2}} ={c} \\ $$$$\left({u}+{v}\right)^{\mathrm{2}} +\left({u}+{v}\right)\left({u}−{v}\right)+\left({u}−{v}\right)^{\mathrm{2}} ={c} \\ $$$$\mathrm{3}{u}^{\mathrm{2}} +{v}^{\mathrm{2}} ={c}\:\rightarrow\:{elipse} \\ $$$$\Rightarrow{u}=\sqrt{\frac{{c}}{\mathrm{3}}}\:\mathrm{cos}\:\theta,\:{v}=\sqrt{{c}}\:\mathrm{sin}\:\theta \\ $$$$ \\ $$$${k}={x}^{\mathrm{2}} −{xy}+{y}^{\mathrm{2}} \\ $$$$\:\:=\left({u}+{v}\right)^{\mathrm{2}} −\left({u}+{v}\right)\left({u}−{v}\right)+\left({u}−{v}\right)^{\mathrm{2}} \\ $$$$\:\:={u}^{\mathrm{2}} +\mathrm{3}{v}^{\mathrm{2}} \\ $$$$\:\:=\frac{{c}\:\mathrm{cos}^{\mathrm{2}} \:\theta}{\mathrm{3}}+\mathrm{3}{c}\:\mathrm{sin}^{\mathrm{2}} \:\theta \\ $$$$\:\:=\frac{{c}\:\mathrm{cos}^{\mathrm{2}} \:\theta}{\mathrm{3}}+\mathrm{3}{c}\left(\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \:\theta\right) \\ $$$$\:\:=\left(\mathrm{3}−\frac{\mathrm{8}\:\mathrm{cos}^{\mathrm{2}} \:\theta}{\mathrm{3}}\right){c} \\ $$$$\Rightarrow{k}_{{min}} =\left(\mathrm{3}−\frac{\mathrm{8}}{\mathrm{3}}\right){c}=\frac{{c}}{\mathrm{3}} \\ $$$$\Rightarrow{k}_{{max}} =\left(\mathrm{3}−\mathrm{0}\right){c}=\mathrm{3}{c} \\ $$