
Question and Answers Forum
Question Number 17867 by Mr easymsn last updated on 11/Jul/17
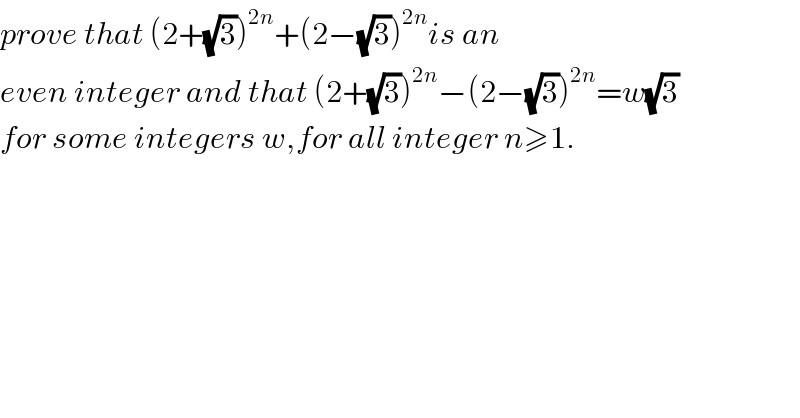
Answered by alex041103 last updated on 11/Jul/17
![We expand using (a+b)^n =Σ_(k=0) ^n ((n),(k) ) a^(n−k) b^k We get (2+(√3))^(2n) + (2−(√3))^(2n) = =Σ_(k=0) ^(2n) (((2n)),(( k)) ) 2^(2n−k) ((√3))^k [1+(−1)^k ] When k≡1(mod 2) (((2n)),(( k)) ) 2^(2n−k) ((√3))^k [1+(−1)^k ]=0 That′s why k≡0(mod 2) ⇒(2+(√3))^(2n) + (2−(√3))^(2n) = =Σ_(k=0) ^n (((2n)),(( 2k)) ) 2^(2n−2k) (3^(1/2) )^(2k) ×2 =2Σ_(k=0) ^n (((2n)),(( 2k)) ) 2^(2n−2k) ×3^k It′s easy to show that Σ_(k=0) ^n (((2n)),(( 2k)) ) 2^(2n−2k) ×3^k ∈ N ⇒(((2+(√3))^(2n) +(2−(√3))^(2n) )/2)∈N The same procedure works for the next statement (2+(√3))^(2n) − (2−(√3))^(2n) = =Σ_(k=0) ^(2n) (((2n)),(( k)) ) 2^(2n−k) ((√3))^k [1+(−1)^(k+1) ] Then we substitute k=2t+1 and (2+(√3))^(2n) − (2−(√3))^(2n) = =Σ_(k=0) ^(n−1) ((( 2n)),(( 2k+1)) ) 2^(2n−2k) ((√3))3^k =(√3)Σ_(k=0) ^(n−1) ((( 2n)),(( 2k+1)) ) 2^(2n−2k) 3^k ⇒(2+(√3))^(2n) − (2−(√3))^(2n) =w(√3), w∈N](Q17868.png)
Commented by alex041103 last updated on 12/Jul/17
| ||
Question and Answers Forum | ||
Question Number 17867 by Mr easymsn last updated on 11/Jul/17 | ||
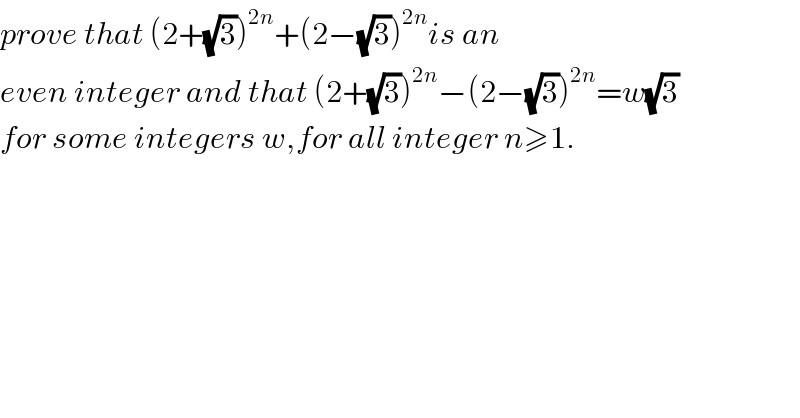 | ||
Answered by alex041103 last updated on 11/Jul/17 | ||
![We expand using (a+b)^n =Σ_(k=0) ^n ((n),(k) ) a^(n−k) b^k We get (2+(√3))^(2n) + (2−(√3))^(2n) = =Σ_(k=0) ^(2n) (((2n)),(( k)) ) 2^(2n−k) ((√3))^k [1+(−1)^k ] When k≡1(mod 2) (((2n)),(( k)) ) 2^(2n−k) ((√3))^k [1+(−1)^k ]=0 That′s why k≡0(mod 2) ⇒(2+(√3))^(2n) + (2−(√3))^(2n) = =Σ_(k=0) ^n (((2n)),(( 2k)) ) 2^(2n−2k) (3^(1/2) )^(2k) ×2 =2Σ_(k=0) ^n (((2n)),(( 2k)) ) 2^(2n−2k) ×3^k It′s easy to show that Σ_(k=0) ^n (((2n)),(( 2k)) ) 2^(2n−2k) ×3^k ∈ N ⇒(((2+(√3))^(2n) +(2−(√3))^(2n) )/2)∈N The same procedure works for the next statement (2+(√3))^(2n) − (2−(√3))^(2n) = =Σ_(k=0) ^(2n) (((2n)),(( k)) ) 2^(2n−k) ((√3))^k [1+(−1)^(k+1) ] Then we substitute k=2t+1 and (2+(√3))^(2n) − (2−(√3))^(2n) = =Σ_(k=0) ^(n−1) ((( 2n)),(( 2k+1)) ) 2^(2n−2k) ((√3))3^k =(√3)Σ_(k=0) ^(n−1) ((( 2n)),(( 2k+1)) ) 2^(2n−2k) 3^k ⇒(2+(√3))^(2n) − (2−(√3))^(2n) =w(√3), w∈N](Q17868.png) | ||
| ||
Commented by alex041103 last updated on 12/Jul/17 | ||
 | ||