Question Number 18015 by alex041103 last updated on 13/Jul/17
$${Just}\:{for}\:{fun} \\ $$$${Prove}\:{that}\:{there}\:{are}\:{no}\:{real}\:{numbers}\:{A}\:{and}\:{B}\:{that} \\ $$$${satisfy}\: \\ $$$${sinA}=\frac{\mathrm{2}}{{sinB}} \\ $$
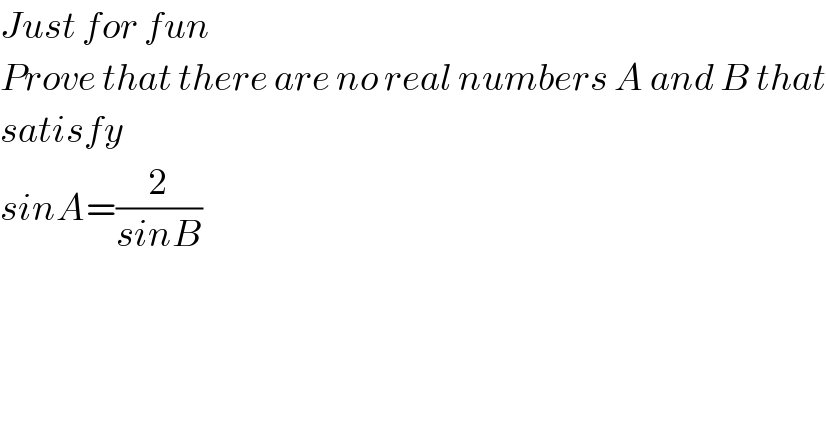
Commented by alex041103 last updated on 13/Jul/17
$${if}\:{you}\:{want}\:{you}\:{can}\:{generalize}\:{it}\: \\ $$$${for}\:{complex}\:{numbers}\:{and}\:{show}\:{that} \\ $$$${if}\:{A},{B}\in\mathbb{C}\:{there}\:{are}\:{such}\:{numbers} \\ $$$${which}\:{sitisfy}\:{this} \\ $$
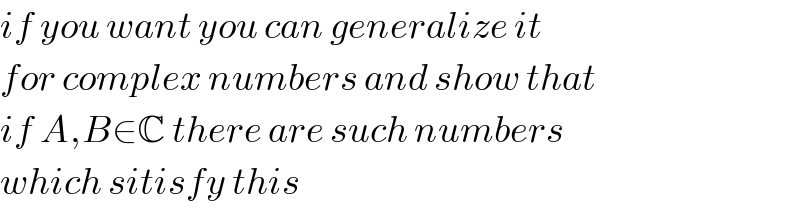
Answered by Tinkutara last updated on 13/Jul/17
![LHS ∈ [−1, 1] but RHS ∈ (−∞, −2] ∪ [2, ∞) hence there is no solution in real numbers.](Q18017.png)
$$\mathrm{LHS}\:\in\:\left[−\mathrm{1},\:\mathrm{1}\right]\:\mathrm{but} \\ $$$$\mathrm{RHS}\:\in\:\left(−\infty,\:−\mathrm{2}\right]\:\cup\:\left[\mathrm{2},\:\infty\right)\:\mathrm{hence}\:\mathrm{there} \\ $$$$\mathrm{is}\:\mathrm{no}\:\mathrm{solution}\:\mathrm{in}\:\mathrm{real}\:\mathrm{numbers}. \\ $$
Commented by alex041103 last updated on 14/Jul/17
$${What}\:{about}\:{the}\:{complex}\:{numbers}? \\ $$
Commented by alex041103 last updated on 14/Jul/17
$${yess} \\ $$
Commented by alex041103 last updated on 14/Jul/17
$${for}\:{complex}\:{numbers}\:{i}'{ll}\:{post}\:{a}\:{solution} \\ $$$${after}\:\mathrm{1}.\mathrm{5}\:{hours} \\ $$
Answered by alex041103 last updated on 14/Jul/17
![Tinkutara did a nice proof for the case where A,B∈R But what about the case where A,B∈C (complex numbers) We know that for z∈C, sin z ∈(−∞,∞) So clearly there are solutions. In fact there are infinate number of solutions. We know that for z∈C (1) e^(iz) =cos z + isin z (Euler′s formula) ⇒e^(i(−z)) =cos z−isin z or (2) −e^(−iz) =−cos z + isin z Then we add (1) and (2) 2isin z = e^(iz) − e^(−iz) ⇒sin z = ((e^(iz) − e^(−iz) )/(2i)) Now let′s define sin^(−1) (z) using the complex definition for sin z. Let s = sin z ⇒2is=e^(iz) −e^(−iz) 2ise^(iz) =(e^(iz) )^2 −1 let t=e^(iz) t^2 −2ist−1=0 ⇒t_(1;2) =((2is±(√(−4s^2 +4)))/2)=is±(√(1−s^2 )) ⇒ln(e^(iz) )=ln(is±(√(1−s^2 )))=iz ⇒z=sin^(−1) (s)=−i ln(is±(√(1−s^2 ))) And you can see if you substitute back s=sin z, after you simplify you′ll get sin^(−1) (sin z)=z. So now sinA=(2/(sinB)) ⇒A=sin^(−1) ((2/(sin B))) we insert that into the formula and we get A=−i ln(((2i)/(sin B))±(√(1−(4/(sin^2 B))))) And we add 2kπ (k∈Z) because sin(A)=sin(A+2π) A=−i ln(((2i)/(sin B))±(√(1−(4/(sin^2 B))))) +2kπ Example: sin A=(2/(sin 45°))=2(√2) A=−i ln(((2i)/((((√2)/2))))±(√(1−(4/(((2/4))))))) + 2kπ= =−i ln(2(√2)i±(√(−7)))+2kπ= =−i ln(2(√2)i±i(√7))+2kπ= =−i ln[i((√8)±(√7))]+2kπ= =−i[ln(i)+ln((√8)±(√7))]+2kπ We now that z=a+bi=r(cosθ + isinθ)=re^(iθ) ⇒z=e^(ln(r) + iθ) ⇒ln(z)=ln(r) + iθ where r=(√(a^2 +b^2 )) and θ=tan^(−1) ((b/a)). In our case ln(i)=ln(1)+i(π/2)=((πi)/2) ⇒A=−i×((πi)/2) − iln((√8)±(√7))+2kπ =(π/2)−iln((√8)±(√7)) + 2kπ ⇒A=(π/2)−i ln((√8)±(√7))+2kπ, k∈Z](Q18060.png)
$$ \\ $$$$ \\ $$$${Tinkutara}\:{did}\:{a}\:{nice}\:{proof}\:{for}\:{the} \\ $$$${case}\:{where}\:{A},{B}\in\mathbb{R} \\ $$$${But}\:{what}\:{about}\:{the}\:{case}\:{where} \\ $$$${A},{B}\in\mathbb{C}\:\left({complex}\:{numbers}\right) \\ $$$${We}\:{know}\:{that}\:{for}\:{z}\in\mathbb{C},\:{sin}\:{z}\:\in\left(−\infty,\infty\right) \\ $$$${So}\:{clearly}\:{there}\:{are}\:{solutions}. \\ $$$${In}\:{fact}\:{there}\:{are}\:{infinate}\:{number}\:{of}\:{solutions}.\: \\ $$$${We}\:{know}\:{that}\:{for}\:{z}\in\mathbb{C} \\ $$$$\left(\mathrm{1}\right)\:{e}^{{iz}} ={cos}\:{z}\:+\:{isin}\:{z}\:\left({Euler}'{s}\:{formula}\right) \\ $$$$\Rightarrow{e}^{{i}\left(−{z}\right)} ={cos}\:{z}−{isin}\:{z} \\ $$$${or} \\ $$$$\left(\mathrm{2}\right)\:\:−{e}^{−{iz}} =−{cos}\:{z}\:+\:{isin}\:{z} \\ $$$${Then}\:{we}\:{add}\:\left(\mathrm{1}\right)\:{and}\:\left(\mathrm{2}\right) \\ $$$$\mathrm{2}{isin}\:{z}\:=\:{e}^{{iz}} \:−\:{e}^{−{iz}} \\ $$$$\Rightarrow{sin}\:{z}\:=\:\frac{{e}^{{iz}} \:−\:{e}^{−{iz}} }{\mathrm{2}{i}} \\ $$$${Now}\:{let}'{s}\:{define}\:{sin}^{−\mathrm{1}} \left({z}\right)\:{using}\:{the} \\ $$$${complex}\:{definition}\:{for}\:{sin}\:{z}. \\ $$$${Let}\:{s}\:=\:{sin}\:{z} \\ $$$$\Rightarrow\mathrm{2}{is}={e}^{{iz}} −{e}^{−{iz}} \\ $$$$\mathrm{2}{ise}^{{iz}} =\left({e}^{{iz}} \right)^{\mathrm{2}} −\mathrm{1} \\ $$$${let}\:{t}={e}^{{iz}} \\ $$$${t}^{\mathrm{2}} −\mathrm{2}{ist}−\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow{t}_{\mathrm{1};\mathrm{2}} =\frac{\mathrm{2}{is}\pm\sqrt{−\mathrm{4}{s}^{\mathrm{2}} +\mathrm{4}}}{\mathrm{2}}={is}\pm\sqrt{\mathrm{1}−{s}^{\mathrm{2}} } \\ $$$$\Rightarrow{ln}\left({e}^{{iz}} \right)={ln}\left({is}\pm\sqrt{\mathrm{1}−{s}^{\mathrm{2}} }\right)={iz} \\ $$$$\Rightarrow{z}=\mathrm{sin}^{−\mathrm{1}} \left({s}\right)=−{i}\:{ln}\left({is}\pm\sqrt{\mathrm{1}−{s}^{\mathrm{2}} }\right) \\ $$$${And}\:{you}\:{can}\:{see}\:{if}\:{you}\:{substitute} \\ $$$${back}\:{s}={sin}\:{z},\:{after}\:{you}\:{simplify} \\ $$$${you}'{ll}\:{get}\:{s}\mathrm{in}^{−\mathrm{1}} \left(\mathrm{sin}\:{z}\right)={z}. \\ $$$${So}\:{now}\: \\ $$$${sinA}=\frac{\mathrm{2}}{{sinB}}\:\Rightarrow{A}={sin}^{−\mathrm{1}} \left(\frac{\mathrm{2}}{{sin}\:{B}}\right) \\ $$$${we}\:{insert}\:{that}\:{into}\:{the}\:{formula}\:{and}\:{we}\:{get} \\ $$$${A}=−{i}\:{ln}\left(\frac{\mathrm{2}{i}}{{sin}\:{B}}\pm\sqrt{\mathrm{1}−\frac{\mathrm{4}}{{sin}^{\mathrm{2}} {B}}}\right) \\ $$$${And}\:{we}\:{add}\:\mathrm{2}{k}\pi\:\left({k}\in\mathbb{Z}\right)\:{because} \\ $$$${sin}\left({A}\right)={sin}\left({A}+\mathrm{2}\pi\right) \\ $$$${A}=−{i}\:{ln}\left(\frac{\mathrm{2}{i}}{{sin}\:{B}}\pm\sqrt{\mathrm{1}−\frac{\mathrm{4}}{{sin}^{\mathrm{2}} {B}}}\right)\:+\mathrm{2}{k}\pi \\ $$$${Example}: \\ $$$${sin}\:{A}=\frac{\mathrm{2}}{{sin}\:\mathrm{45}°}=\mathrm{2}\sqrt{\mathrm{2}} \\ $$$${A}=−{i}\:{ln}\left(\frac{\mathrm{2}{i}}{\left(\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)}\pm\sqrt{\mathrm{1}−\frac{\mathrm{4}}{\left(\frac{\mathrm{2}}{\mathrm{4}}\right)}}\right)\:+\:\mathrm{2}{k}\pi= \\ $$$$=−{i}\:{ln}\left(\mathrm{2}\sqrt{\mathrm{2}}{i}\pm\sqrt{−\mathrm{7}}\right)+\mathrm{2}{k}\pi= \\ $$$$=−{i}\:{ln}\left(\mathrm{2}\sqrt{\mathrm{2}}{i}\pm{i}\sqrt{\mathrm{7}}\right)+\mathrm{2}{k}\pi= \\ $$$$=−{i}\:{ln}\left[{i}\left(\sqrt{\mathrm{8}}\pm\sqrt{\mathrm{7}}\right)\right]+\mathrm{2}{k}\pi= \\ $$$$=−{i}\left[{ln}\left({i}\right)+{ln}\left(\sqrt{\mathrm{8}}\pm\sqrt{\mathrm{7}}\right)\right]+\mathrm{2}{k}\pi \\ $$$${We}\:{now}\:{that}\: \\ $$$${z}={a}+{bi}={r}\left({cos}\theta\:+\:{isin}\theta\right)={re}^{{i}\theta} \\ $$$$\Rightarrow{z}={e}^{{ln}\left({r}\right)\:+\:{i}\theta} \\ $$$$\Rightarrow{ln}\left({z}\right)={ln}\left({r}\right)\:+\:{i}\theta \\ $$$${where}\:{r}=\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:{and}\:\theta=\mathrm{tan}^{−\mathrm{1}} \left(\frac{{b}}{{a}}\right). \\ $$$${In}\:{our}\:{case} \\ $$$${ln}\left({i}\right)={ln}\left(\mathrm{1}\right)+{i}\frac{\pi}{\mathrm{2}}=\frac{\pi{i}}{\mathrm{2}} \\ $$$$\Rightarrow{A}=−{i}×\frac{\pi{i}}{\mathrm{2}}\:−\:{iln}\left(\sqrt{\mathrm{8}}\pm\sqrt{\mathrm{7}}\right)+\mathrm{2}{k}\pi \\ $$$$=\frac{\pi}{\mathrm{2}}−{iln}\left(\sqrt{\mathrm{8}}\pm\sqrt{\mathrm{7}}\right)\:+\:\mathrm{2}{k}\pi \\ $$$$\Rightarrow{A}=\frac{\pi}{\mathrm{2}}−{i}\:{ln}\left(\sqrt{\mathrm{8}}\pm\sqrt{\mathrm{7}}\right)+\mathrm{2}{k}\pi,\:{k}\in\mathbb{Z} \\ $$