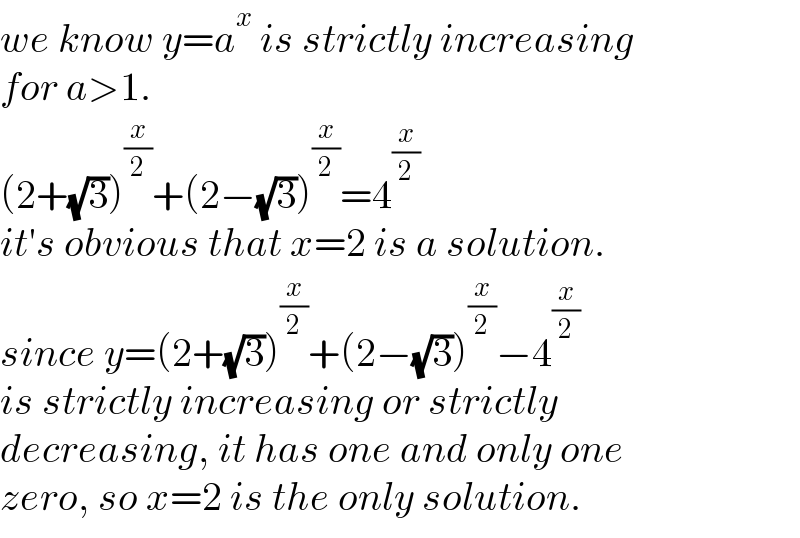Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 180301 by Noorzai last updated on 10/Nov/22

Commented by MJS_new last updated on 10/Nov/22

this has the shape a^x +(1/a^x )=b^x which we can solve for b: b=((a^x +(1/a^x )))^(1/x) and for a: a^x +(1/a^x )=2cosh (xln a) =b^x ⇒ a=e^((cosh^(−1) (b^x /2))/x) but we cannot solve for x. we can { ((always approximate)),((sometimes see an obvious solution)) :}

$$\mathrm{this}\:\mathrm{has}\:\mathrm{the}\:\mathrm{shape} \\ $$$${a}^{{x}} +\frac{\mathrm{1}}{{a}^{{x}} }={b}^{{x}} \\ $$$$\mathrm{which}\:\mathrm{we}\:\mathrm{can}\:\mathrm{solve}\:\mathrm{for}\:{b}: \\ $$$${b}=\sqrt[{{x}}]{{a}^{{x}} +\frac{\mathrm{1}}{{a}^{{x}} }} \\ $$$$\mathrm{and}\:\mathrm{for}\:{a}: \\ $$$${a}^{{x}} +\frac{\mathrm{1}}{{a}^{{x}} }=\mathrm{2cosh}\:\left({x}\mathrm{ln}\:{a}\right)\:={b}^{{x}} \\ $$$$\Rightarrow\:{a}=\mathrm{e}^{\frac{\mathrm{cosh}^{−\mathrm{1}} \:\frac{{b}^{{x}} }{\mathrm{2}}}{{x}}} \\ $$$$\mathrm{but}\:\mathrm{we}\:\mathrm{cannot}\:\mathrm{solve}\:\mathrm{for}\:{x}. \\ $$$$\mathrm{we}\:\mathrm{can}\:\begin{cases}{\mathrm{always}\:\mathrm{approximate}}\\{\mathrm{sometimes}\:\mathrm{see}\:\mathrm{an}\:\mathrm{obvious}\:\mathrm{solution}}\end{cases} \\ $$

Answered by MJS_new last updated on 10/Nov/22

obviously x=2: ((√(2+(√3))))^2 +((√(2−(√3))))^2 =2+(√3)+2−(√3)=4

$$\mathrm{obviously}\:{x}=\mathrm{2}: \\ $$$$\left(\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} +\left(\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} =\mathrm{2}+\sqrt{\mathrm{3}}+\mathrm{2}−\sqrt{\mathrm{3}}=\mathrm{4} \\ $$

Commented by peter frank last updated on 10/Nov/22

how?

$$\mathrm{how}? \\ $$

Commented by MJS_new last updated on 10/Nov/22

((√t))^2 =t what else do you need to know?

$$\left(\sqrt{{t}}\right)^{\mathrm{2}} ={t} \\ $$$$\mathrm{what}\:\mathrm{else}\:\mathrm{do}\:\mathrm{you}\:\mathrm{need}\:\mathrm{to}\:\mathrm{know}? \\ $$

Answered by Rasheed.Sindhi last updated on 10/Nov/22

((√((2+(√3) )/(2−(√3)))) )^x +1=((2/( (√(2−(√3))) )))^x ((√(((2+(√3) )/(2−(√3)))×((2+(√3))/(2+(√3) )))) )^x +1=((2/( (√(2−(√3))) ))×(((√(2+(√3))) )/( (√(2+(√3))))))^x (2+(√3) )^x +1=(2(√(2+(√3))) )^x (2+(√3) )^x −(2(√(2+(√3))) )^x +1=0 (2+(√3) )^x −(4(2+(√3) ))^(x/2) +1=0 (2+(√3) )^x −4^(x/2) (2+(√3) )^(x/2) +1=0 (2+(√3) )^(x/2) =y y^2 −4^(x/2) y+1=0 y=((4±(√(4^x −4)))/2)=(2+(√3) )^(x/2) 2±(√(4^(x−1) −1)) =(2+(√3) )^(x/2) LHS consist of two terms: non-radical & radical and non-radical=2 This is possible when (x/2)=1⇒x=2 and this satisfied also radical terms 2±(√(4^(2−1) −1)) =(2+(√3) )^(2/2) 2+(√3) =(2+(√3) )^(2/2) [ ∵2−(√3) =(2+(√3) )^(2/2) doesn′t satisy] ∴ x=2

$$\left(\sqrt{\frac{\mathrm{2}+\sqrt{\mathrm{3}}\:}{\mathrm{2}−\sqrt{\mathrm{3}}}}\:\right)^{{x}} +\mathrm{1}=\left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}\:}\right)^{{x}} \\ $$$$\left(\sqrt{\frac{\mathrm{2}+\sqrt{\mathrm{3}}\:}{\mathrm{2}−\sqrt{\mathrm{3}}}×\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\mathrm{2}+\sqrt{\mathrm{3}}\:}}\:\:\right)^{{x}} +\mathrm{1}=\left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}\:}×\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}\:}{\:\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}\right)^{{x}} \\ $$$$\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}} +\mathrm{1}=\left(\mathrm{2}\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}\:\right)^{{x}} \\ $$$$\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}} −\left(\mathrm{2}\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}\:\right)^{{x}} +\mathrm{1}=\mathrm{0} \\ $$$$\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}} −\left(\mathrm{4}\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)\right)^{{x}/\mathrm{2}} +\mathrm{1}=\mathrm{0} \\ $$$$\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}} −\mathrm{4}^{{x}/\mathrm{2}} \left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}/\mathrm{2}} +\mathrm{1}=\mathrm{0} \\ $$$$\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}/\mathrm{2}} ={y} \\ $$$${y}^{\mathrm{2}} −\mathrm{4}^{{x}/\mathrm{2}} {y}+\mathrm{1}=\mathrm{0} \\ $$$${y}=\frac{\mathrm{4}\pm\sqrt{\mathrm{4}^{{x}} −\mathrm{4}}}{\mathrm{2}}=\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}/\mathrm{2}} \\ $$$$\:\:\:\:\:\:\mathrm{2}\pm\sqrt{\mathrm{4}^{{x}−\mathrm{1}} −\mathrm{1}}\:=\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{{x}/\mathrm{2}} \\ $$$${LHS}\:{consist}\:{of}\:{two}\:{terms}:\: \\ $$$${non}-{radical}\:\&\:{radical}\: \\ $$$${and}\:{non}-{radical}=\mathrm{2} \\ $$$${This}\:{is}\:{possible}\:{when}\: \\ $$$$\:\:\:\frac{{x}}{\mathrm{2}}=\mathrm{1}\Rightarrow{x}=\mathrm{2}\: \\ $$$${and}\:{this}\:{satisfied}\:{also}\:{radical}\:{terms} \\ $$$$\:\:\:\:\:\:\mathrm{2}\pm\sqrt{\mathrm{4}^{\mathrm{2}−\mathrm{1}} −\mathrm{1}}\:=\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{\mathrm{2}/\mathrm{2}} \\ $$$$\:\:\:\:\:\:\mathrm{2}+\sqrt{\mathrm{3}}\:=\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{\mathrm{2}/\mathrm{2}} \\ $$$$\left[\:\because\mathrm{2}−\sqrt{\mathrm{3}}\:=\left(\mathrm{2}+\sqrt{\mathrm{3}}\:\right)^{\mathrm{2}/\mathrm{2}} \:{doesn}'{t}\:{satisy}\right] \\ $$$$\therefore\:\:\:{x}=\mathrm{2} \\ $$

Answered by mr W last updated on 10/Nov/22

we know y=a^x is strictly increasing for a>1. (2+(√3))^(x/2) +(2−(√3))^(x/2) =4^(x/2) it′s obvious that x=2 is a solution. since y=(2+(√3))^(x/2) +(2−(√3))^(x/2) −4^(x/2) is strictly increasing or strictly decreasing, it has one and only one zero, so x=2 is the only solution.

$${we}\:{know}\:{y}={a}^{{x}} \:{is}\:{strictly}\:{increasing} \\ $$$${for}\:{a}>\mathrm{1}. \\ $$$$\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)^{\frac{{x}}{\mathrm{2}}} +\left(\mathrm{2}−\sqrt{\mathrm{3}}\right)^{\frac{{x}}{\mathrm{2}}} =\mathrm{4}^{\frac{{x}}{\mathrm{2}}} \\ $$$${it}'{s}\:{obvious}\:{that}\:{x}=\mathrm{2}\:{is}\:{a}\:{solution}. \\ $$$${since}\:{y}=\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)^{\frac{{x}}{\mathrm{2}}} +\left(\mathrm{2}−\sqrt{\mathrm{3}}\right)^{\frac{{x}}{\mathrm{2}}} −\mathrm{4}^{\frac{{x}}{\mathrm{2}}} \\ $$$${is}\:{strictly}\:{increasing}\:{or}\:{strictly} \\ $$$${decreasing},\:{it}\:{has}\:{one}\:{and}\:{only}\:{one} \\ $$$${zero},\:{so}\:{x}=\mathrm{2}\:{is}\:{the}\:{only}\:{solution}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com