
Question Number 181318 by mr W last updated on 23/Nov/22

$${if}\:{x}+{y}+{z}=\mathrm{0},\:{find}\:{the}\:{maximum}\:{of} \\ $$$$\frac{\mid{x}+\mathrm{2}{y}+\mathrm{3}{z}\mid}{\:\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} }}. \\ $$
Commented by mr W last updated on 02/Dec/22
$${see}\:{Q}\mathrm{181875}\:{for}\:{other}\:{solutions} \\ $$
Answered by Frix last updated on 24/Nov/22
![((∣x+2y+3z∣)/( (√(x^2 +y^2 +z^2 )))) z=−(x+y) (((√2)∣2x+y∣)/( 2(√(x^2 +xy+y^2 )))) y=cx (((√2)∣c+2∣)/(2(√(c^2 +c+1)))) ⇒ min at c=−2 ((√2)/2)×((d[((∣c+2∣)/( (√(c^2 +c+1))))])/dc)=−((3(√2)c(c+2))/( 4∣c+2∣(√((c^2 +c+1)^3 ))))=0 ⇒ max at c=0 ⇒ 0≤(((√2)∣c+2∣)/(2(√(c^2 +c+1)))) ≤(√2) ∀r∈R\{0}: the minimum is at (x, y, z)=(r, −2r, r) the maximum is at (x, y, z)=(r, 0, −r)](Q181341.png)
$$\frac{\mid{x}+\mathrm{2}{y}+\mathrm{3}{z}\mid}{\:\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} }} \\ $$$${z}=−\left({x}+{y}\right) \\ $$$$\frac{\sqrt{\mathrm{2}}\mid\mathrm{2}{x}+{y}\mid}{\:\mathrm{2}\sqrt{{x}^{\mathrm{2}} +{xy}+{y}^{\mathrm{2}} }} \\ $$$${y}={cx} \\ $$$$\frac{\sqrt{\mathrm{2}}\mid{c}+\mathrm{2}\mid}{\mathrm{2}\sqrt{{c}^{\mathrm{2}} +{c}+\mathrm{1}}} \\ $$$$\Rightarrow\:\mathrm{min}\:\mathrm{at}\:\mathrm{c}=−\mathrm{2} \\ $$$$\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}×\frac{{d}\left[\frac{\mid{c}+\mathrm{2}\mid}{\:\sqrt{{c}^{\mathrm{2}} +{c}+\mathrm{1}}}\right]}{{dc}}=−\frac{\mathrm{3}\sqrt{\mathrm{2}}{c}\left({c}+\mathrm{2}\right)}{\:\mathrm{4}\mid{c}+\mathrm{2}\mid\sqrt{\left({c}^{\mathrm{2}} +{c}+\mathrm{1}\right)^{\mathrm{3}} }}=\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{max}\:\mathrm{at}\:{c}=\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{0}\leqslant\frac{\sqrt{\mathrm{2}}\mid{c}+\mathrm{2}\mid}{\mathrm{2}\sqrt{{c}^{\mathrm{2}} +{c}+\mathrm{1}}}\:\leqslant\sqrt{\mathrm{2}} \\ $$$$\forall{r}\in\mathbb{R}\backslash\left\{\mathrm{0}\right\}: \\ $$$$\mathrm{the}\:\mathrm{minimum}\:\mathrm{is}\:\mathrm{at}\:\left({x},\:{y},\:{z}\right)=\left({r},\:−\mathrm{2}{r},\:{r}\right) \\ $$$$\mathrm{the}\:\mathrm{maximum}\:\mathrm{is}\:\mathrm{at}\:\left({x},\:{y},\:{z}\right)=\left({r},\:\mathrm{0},\:−{r}\right) \\ $$
Answered by a.lgnaoui last updated on 24/Nov/22
![x+2y+3z=2(x+y+z)+z−x=z−x ∣x+2y+3z∣<∣x∣+∣z∣ x^2 +y^2 +z^2 =(x+y+z)^2 −2[y(x+z)+xz] =2[(x+z)^2 −xz] ((∣x+2y+3z∣)/( (√(x^2 +y^2 +z^2 ))))<((∣x+z∣)/(∣x+z∣(√(2−((2xz)/((x+z)^2 )))))) <((∣x+z∣)/( (√((2(x+z)^2 )/1))))<(1/( (√2))) the maximum is ((√2)/2)](Q181325.png)
$${x}+\mathrm{2}{y}+\mathrm{3}{z}=\mathrm{2}\left({x}+{y}+{z}\right)+{z}−{x}={z}−{x} \\ $$$$\mid{x}+\mathrm{2}{y}+\mathrm{3}{z}\mid<\mid{x}\mid+\mid{z}\mid \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} =\left({x}+{y}+{z}\right)^{\mathrm{2}} −\mathrm{2}\left[{y}\left({x}+{z}\right)+{xz}\right] \\ $$$$=\mathrm{2}\left[\left({x}+{z}\right)^{\mathrm{2}} −{xz}\right] \\ $$$$\frac{\mid{x}+\mathrm{2}{y}+\mathrm{3}{z}\mid}{\:\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} }}<\frac{\mid{x}+{z}\mid}{\mid{x}+{z}\mid\sqrt{\mathrm{2}−\frac{\mathrm{2}{xz}}{\left({x}+{z}\right)^{\mathrm{2}} }}} \\ $$$$<\frac{\mid{x}+{z}\mid}{\:\sqrt{\frac{\mathrm{2}\left({x}+{z}\right)^{\mathrm{2}} }{\mathrm{1}}}}<\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$$${the}\:{maximum}\:{is}\:\:\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$
Commented by Frix last updated on 24/Nov/22
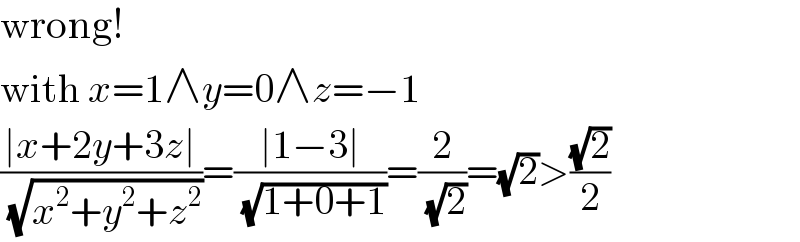
$$\mathrm{wrong}! \\ $$$$\mathrm{with}\:{x}=\mathrm{1}\wedge{y}=\mathrm{0}\wedge{z}=−\mathrm{1} \\ $$$$\frac{\mid{x}+\mathrm{2}{y}+\mathrm{3}{z}\mid}{\:\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} }}=\frac{\mid\mathrm{1}−\mathrm{3}\mid}{\:\sqrt{\mathrm{1}+\mathrm{0}+\mathrm{1}}}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}}=\sqrt{\mathrm{2}}>\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$
