
Question and Answers Forum
Question Number 181360 by lapache last updated on 24/Nov/22
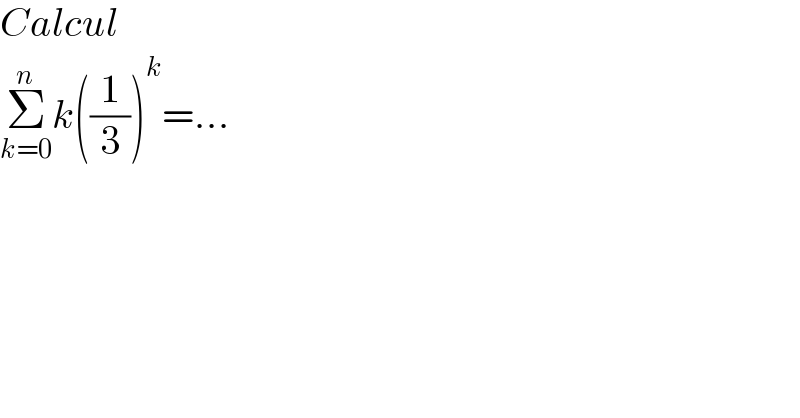
Answered by mahdipoor last updated on 24/Nov/22
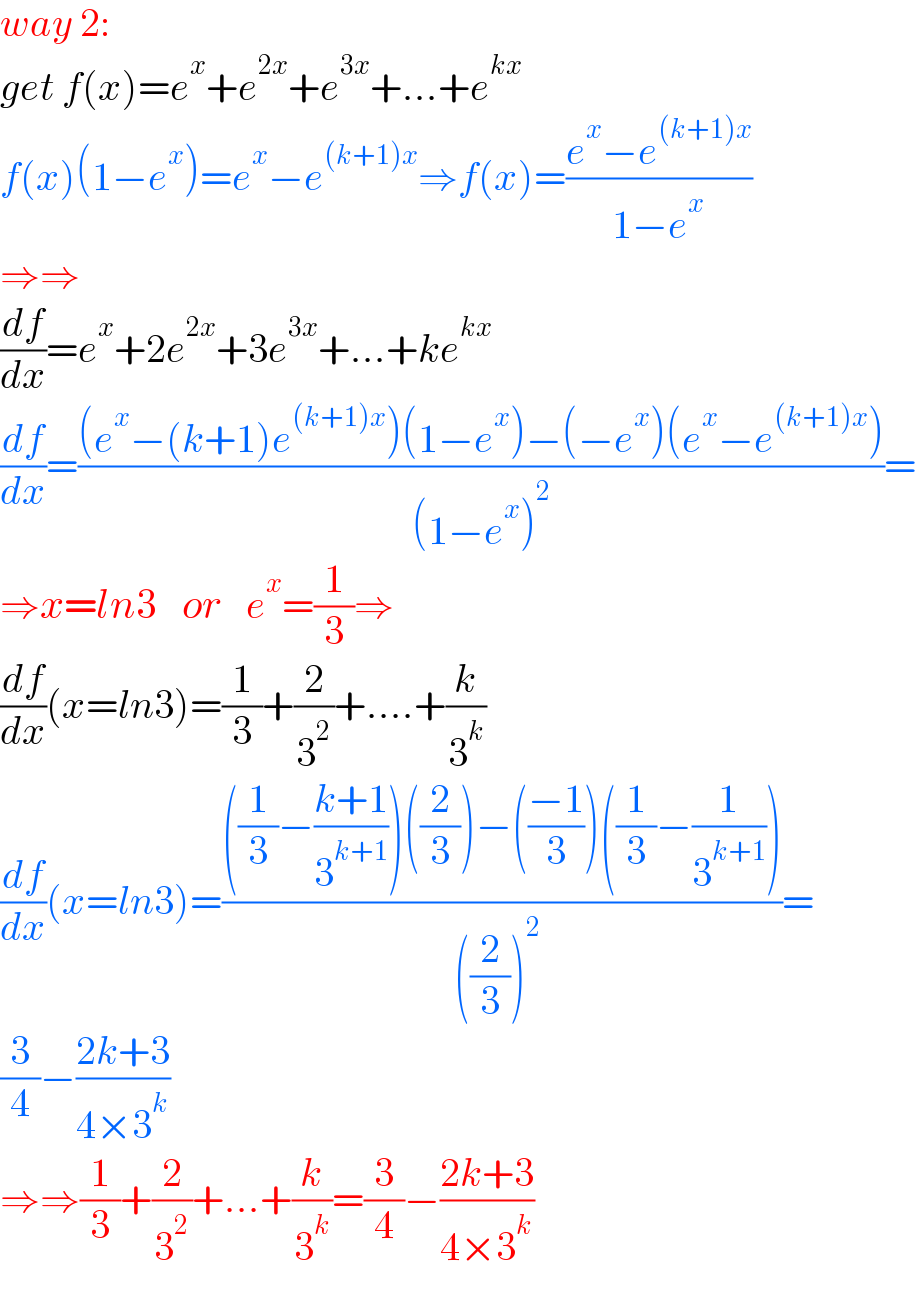
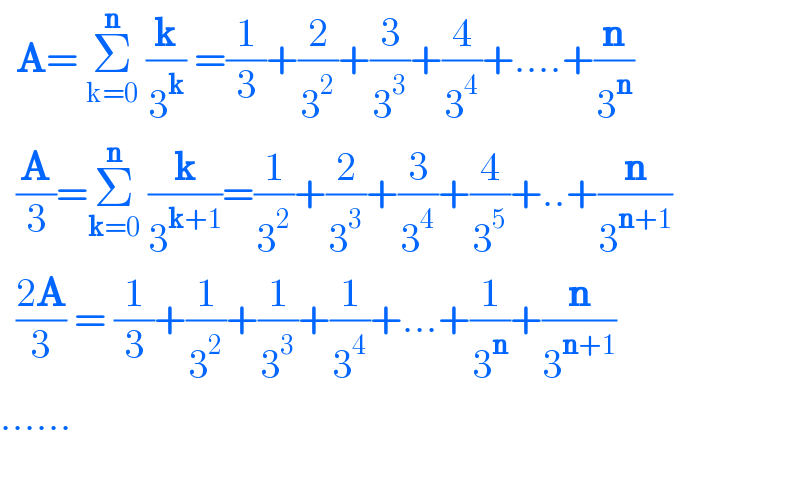
Answered by SEKRET last updated on 24/Nov/22
Answered by mahdipoor last updated on 24/Nov/22

| ||
Question and Answers Forum | ||
Question Number 181360 by lapache last updated on 24/Nov/22 | ||
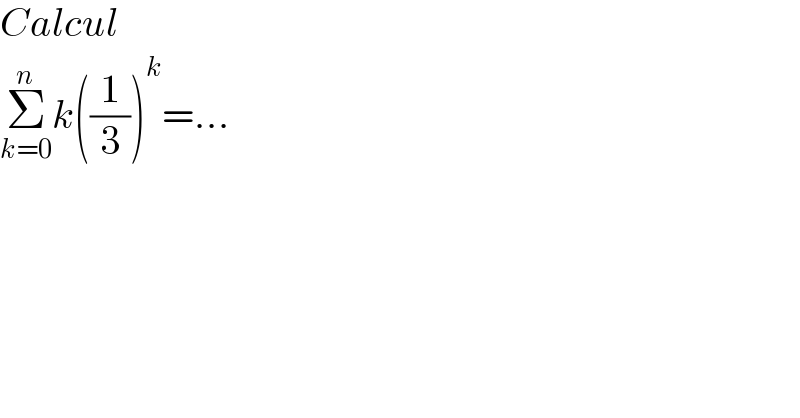 | ||
Answered by mahdipoor last updated on 24/Nov/22 | ||
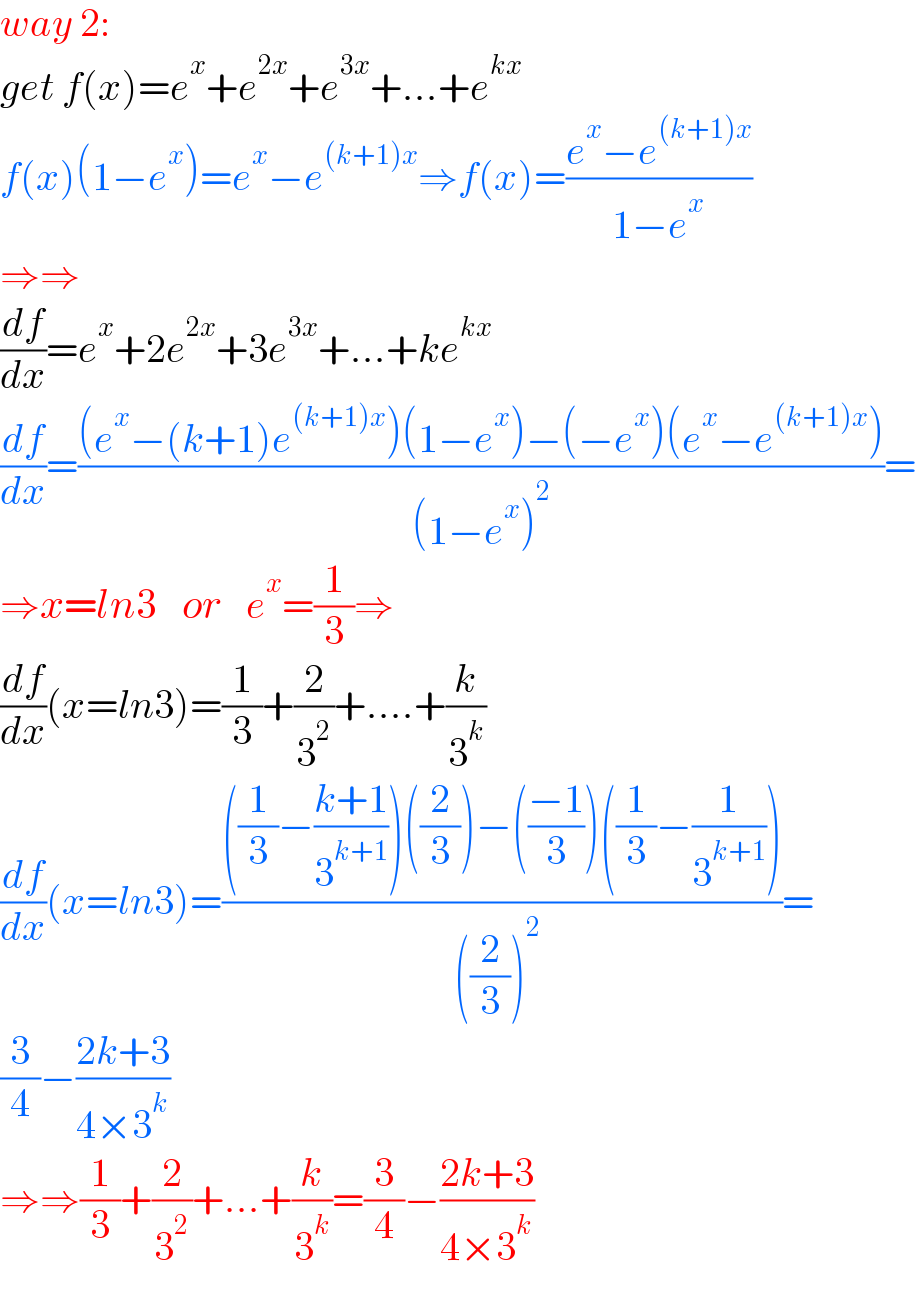 | ||
| ||
Answered by SEKRET last updated on 24/Nov/22 | ||
 | ||
| ||
Answered by mahdipoor last updated on 24/Nov/22 | ||
 | ||
| ||