Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 181382 by liuxinnan last updated on 24/Nov/22

Commented by liuxinnan last updated on 24/Nov/22

n >?

$${n}\:>? \\ $$

Answered by Frix last updated on 24/Nov/22

(n/(log_2 n))=(n/((ln n)/(ln 2)))=((nln 2)/(ln n)) first solve the equation (n/(ln n))=(3/(ln 2)) −((ln n)/n)=−((ln 2)/3) let n=e^(−t) te^t =((ln 2)/3) LambertW−function leads to t≈−2.29652∨t≈−.317338 ⇔ n≈9.93954∨n≈1.37347 now (n/(ln n))>(3/(ln 2)) and obviously this leads to 1<n<≈1.37347∨n>≈9.93954

$$\frac{{n}}{\mathrm{log}_{\mathrm{2}} \:{n}}=\frac{{n}}{\frac{\mathrm{ln}\:{n}}{\mathrm{ln}\:\mathrm{2}}}=\frac{{n}\mathrm{ln}\:\mathrm{2}}{\mathrm{ln}\:{n}} \\ $$$$\mathrm{first}\:\mathrm{solve}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\frac{{n}}{\mathrm{ln}\:{n}}=\frac{\mathrm{3}}{\mathrm{ln}\:\mathrm{2}} \\ $$$$−\frac{\mathrm{ln}\:{n}}{{n}}=−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}} \\ $$$$\mathrm{let}\:{n}=\mathrm{e}^{−{t}} \\ $$$${t}\mathrm{e}^{{t}} =\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}} \\ $$$$\mathrm{LambertW}−\mathrm{function}\:\mathrm{leads}\:\mathrm{to} \\ $$$${t}\approx−\mathrm{2}.\mathrm{29652}\vee{t}\approx−.\mathrm{317338} \\ $$$$\Leftrightarrow \\ $$$${n}\approx\mathrm{9}.\mathrm{93954}\vee{n}\approx\mathrm{1}.\mathrm{37347} \\ $$$$\mathrm{now} \\ $$$$\frac{{n}}{\mathrm{ln}\:{n}}>\frac{\mathrm{3}}{\mathrm{ln}\:\mathrm{2}}\:\mathrm{and}\:\mathrm{obviously}\:\mathrm{this}\:\mathrm{leads}\:\mathrm{to} \\ $$$$\mathrm{1}<{n}<\approx\mathrm{1}.\mathrm{37347}\vee{n}>\approx\mathrm{9}.\mathrm{93954} \\ $$

Commented by Socracious last updated on 25/Nov/22

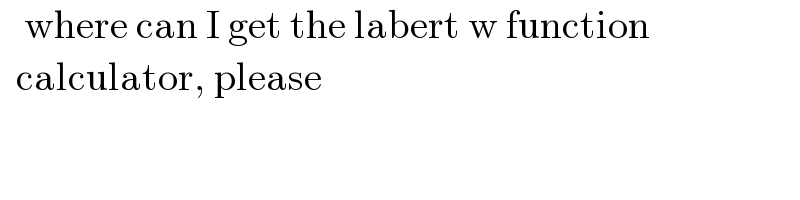
where can I get the labert w function calculator, please

$$\:\:\:\mathrm{where}\:\mathrm{can}\:\mathrm{I}\:\mathrm{get}\:\mathrm{the}\:\mathrm{labert}\:\mathrm{w}\:\mathrm{function} \\ $$$$\:\:\mathrm{calculator},\:\mathrm{please} \\ $$

Answered by mr W last updated on 24/Nov/22

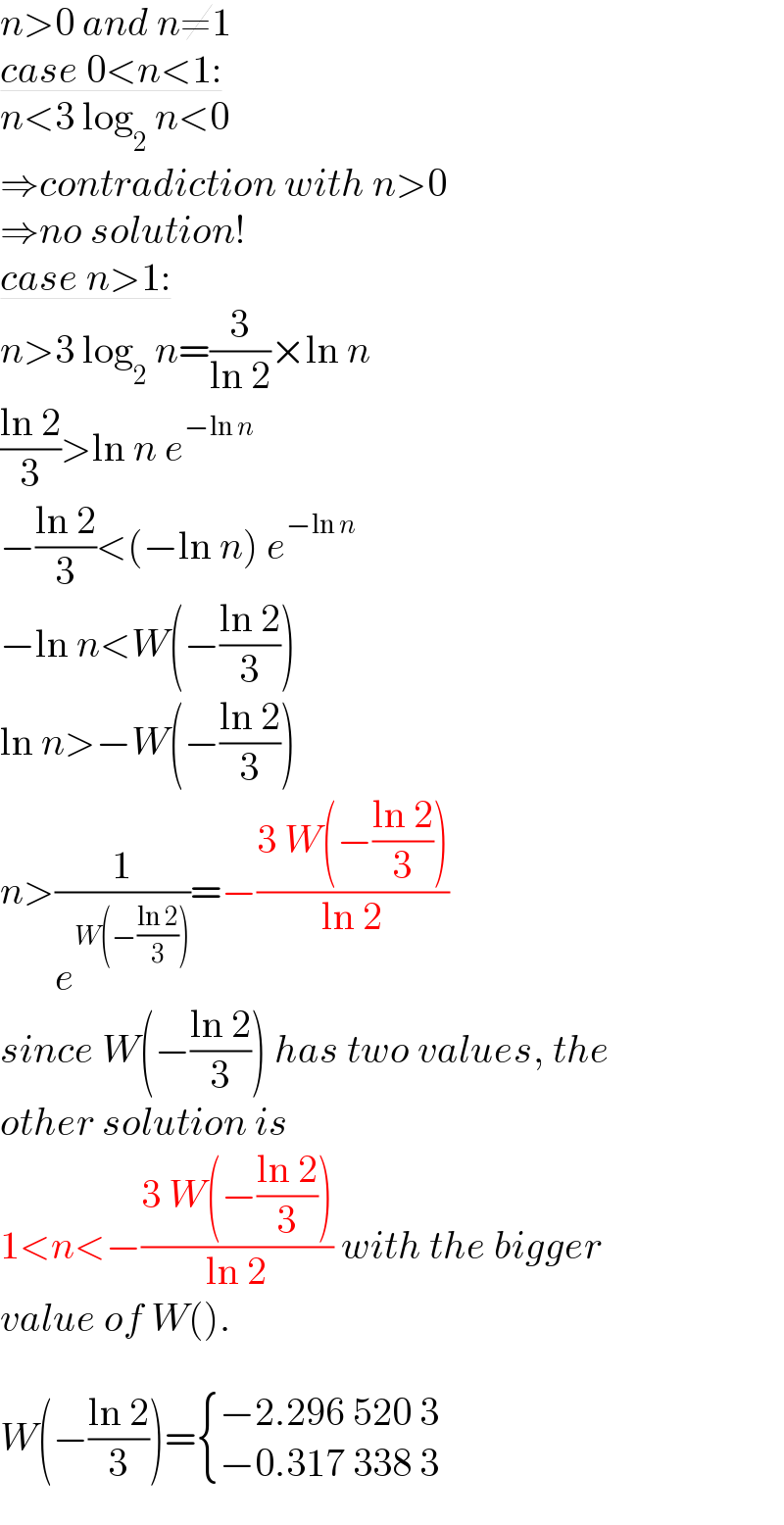
n>0 and n≠1 case 0<n<1: n<3 log_2 n<0 ⇒contradiction with n>0 ⇒no solution! case n>1: n>3 log_2 n=(3/(ln 2))×ln n ((ln 2)/3)>ln n e^(−ln n) −((ln 2)/3)<(−ln n) e^(−ln n) −ln n<W(−((ln 2)/3)) ln n>−W(−((ln 2)/3)) n>(1/e^(W(−((ln 2)/3))) )=−((3 W(−((ln 2)/3)))/(ln 2)) since W(−((ln 2)/3)) has two values, the other solution is 1<n<−((3 W(−((ln 2)/3)))/(ln 2)) with the bigger value of W(). W(−((ln 2)/3))= { ((−2.296 520 3)),((−0.317 338 3)) :}

$${n}>\mathrm{0}\:{and}\:{n}\neq\mathrm{1} \\ $$$$\underline{{case}\:\mathrm{0}<{n}<\mathrm{1}:} \\ $$$${n}<\mathrm{3}\:\mathrm{log}_{\mathrm{2}} \:{n}<\mathrm{0}\: \\ $$$$\Rightarrow{contradiction}\:{with}\:{n}>\mathrm{0} \\ $$$$\Rightarrow{no}\:{solution}! \\ $$$$\underline{{case}\:{n}>\mathrm{1}:} \\ $$$${n}>\mathrm{3}\:\mathrm{log}_{\mathrm{2}} \:{n}=\frac{\mathrm{3}}{\mathrm{ln}\:\mathrm{2}}×\mathrm{ln}\:{n} \\ $$$$\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}>\mathrm{ln}\:{n}\:{e}^{−\mathrm{ln}\:{n}} \\ $$$$−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}<\left(−\mathrm{ln}\:{n}\right)\:{e}^{−\mathrm{ln}\:{n}} \\ $$$$−\mathrm{ln}\:{n}<{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}\right) \\ $$$$\mathrm{ln}\:{n}>−{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}\right) \\ $$$${n}>\frac{\mathrm{1}}{{e}^{{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}\right)} }=−\frac{\mathrm{3}\:{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}\right)}{\mathrm{ln}\:\mathrm{2}} \\ $$$${since}\:{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}\right)\:{has}\:{two}\:{values},\:{the} \\ $$$${other}\:{solution}\:{is} \\ $$$$\mathrm{1}<{n}<−\frac{\mathrm{3}\:{W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}\right)}{\mathrm{ln}\:\mathrm{2}}\:{with}\:{the}\:{bigger}\: \\ $$$${value}\:{of}\:{W}\left(\right). \\ $$$$ \\ $$$${W}\left(−\frac{\mathrm{ln}\:\mathrm{2}}{\mathrm{3}}\right)=\begin{cases}{−\mathrm{2}.\mathrm{296}\:\mathrm{520}\:\mathrm{3}}\\{−\mathrm{0}.\mathrm{317}\:\mathrm{338}\:\mathrm{3}}\end{cases} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com