
Question and Answers Forum
Question Number 181840 by srikanth2684 last updated on 01/Dec/22

Commented by mr W last updated on 01/Dec/22
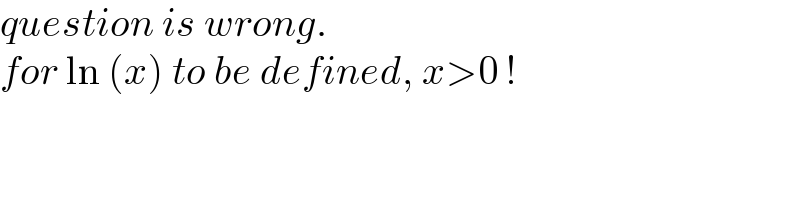
Commented by CElcedricjunior last updated on 01/Dec/22
![∫_(−1) ^4 lnxdx=[xln∣x∣−x]_(−1) ^4 =+∞](Q181844.png)
Commented by Frix last updated on 01/Dec/22
![+∞ is wrong! ∫(dx/x)=ln ∣x∣ +C In this case the absolute value makes sense because obviously the area between the graph of y=(1/x) and the x−axis is the same in both intervals [a, b] and [−b, −a] ⇒ ∫_(−r) ^(+r) (dx/x)=0∀r∈R despite of the singularity. ∫ln x dx=−x(1−ln x)+C In this case y=ln x is not defined for x≤0 and x, y∈R If we let x, y∈C: x<0∧r=∣x∣ ⇔ x=re^(iπ) and ln x =ln r +πi ⇒ ∫_(−1) ^4 ln x dx=−5+8ln 2 +πi [real (ln x) =ln ∣x∣; imag (ln x) =((π(1−sign x))/2)]](Q181870.png)
| ||
Question and Answers Forum | ||
Question Number 181840 by srikanth2684 last updated on 01/Dec/22 | ||
 | ||
Commented by mr W last updated on 01/Dec/22 | ||
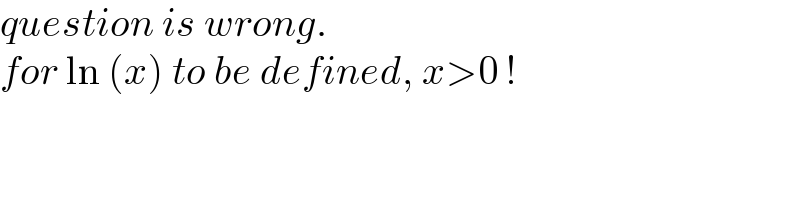 | ||
Commented by CElcedricjunior last updated on 01/Dec/22 | ||
![∫_(−1) ^4 lnxdx=[xln∣x∣−x]_(−1) ^4 =+∞](Q181844.png) | ||
Commented by Frix last updated on 01/Dec/22 | ||
![+∞ is wrong! ∫(dx/x)=ln ∣x∣ +C In this case the absolute value makes sense because obviously the area between the graph of y=(1/x) and the x−axis is the same in both intervals [a, b] and [−b, −a] ⇒ ∫_(−r) ^(+r) (dx/x)=0∀r∈R despite of the singularity. ∫ln x dx=−x(1−ln x)+C In this case y=ln x is not defined for x≤0 and x, y∈R If we let x, y∈C: x<0∧r=∣x∣ ⇔ x=re^(iπ) and ln x =ln r +πi ⇒ ∫_(−1) ^4 ln x dx=−5+8ln 2 +πi [real (ln x) =ln ∣x∣; imag (ln x) =((π(1−sign x))/2)]](Q181870.png) | ||