
Question and Answers Forum
Question Number 182681 by Acem last updated on 12/Dec/22

Commented by mr W last updated on 12/Dec/22

Commented by Acem last updated on 12/Dec/22
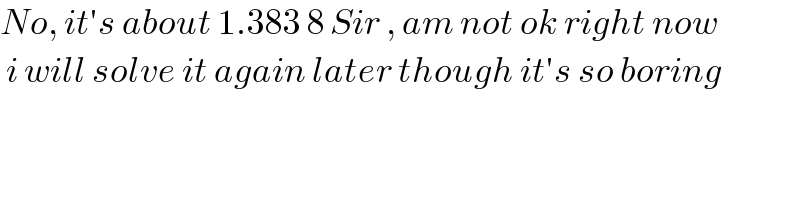
Commented by mr W last updated on 13/Dec/22

Commented by manxsol last updated on 13/Dec/22

Commented by manxsol last updated on 14/Dec/22
![combination metod friz+metod rasheed solution (√x)+(√y)=(√u) x(a),y(a), u=k 4ux=[u+(x−y)]^2 I 4uy=[u−(x−y)]^2 II sea x−y=qa+b lineal solve I ⇒ma^2 +na+p solve II⇒ ma^2 +na+p solve ma^2 +na+p=0 a_1 a_2 DEVELOPING (√x)+(√y)=(√u) ((√x)−(√y))((√x)+(√y))=(√u)((√x)−(√y)) x−y=(√u) ((√x)−(√y)) .................. (√x)−(√y)=(((x−y))/( (√u))) (√x)+(√y)=(√u) .................. add 2(√x)=(((u+(x−y)))/( (√u))) 4xu=(u+(x−y)^2 ) 4yu=(u−(x−y)^2 ) CONCLUSION (√x)+(√y)=(√u) is equivalent a solve {4xu=(u+(x−y))^2 } ∪ {4yu=(u−(x−y))^(2 ) }](Q182764.png)
Commented by manxsol last updated on 14/Dec/22
![aplication (√(39−10a−a^2 ))+(√(9−a^2 ))=2(√(14)) x=39−10a−a^2 y=9−a^2 u=56 the magic : x−y=30−10a solve with 4yu=(u−(x−y))^2 (it easy) 4(56)(9−a^2 )=[56−(30−10a)]^2 4(56)(9−a^2 )=[26+10a]^2 504−56a^2 =(13+5a)^2 504−56a^2 =169+25a^2 +130a 81a^2 +130a−335=0 a_1 = 1.3837 a_2 =−2.9887 root strange](Q182765.png)
Answered by Frix last updated on 12/Dec/22

Commented by Acem last updated on 12/Dec/22

Commented by Acem last updated on 12/Dec/22
Commented by Frix last updated on 12/Dec/22

Commented by Acem last updated on 13/Dec/22
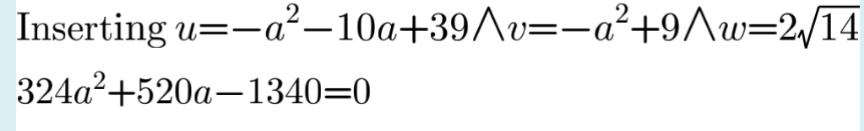
Commented by Acem last updated on 13/Dec/22
Answered by Acem last updated on 12/Dec/22
![(√(39− 10 a −a^2 )) + (√(9−a^2 )) = 2 (√(14)) (√(10(3 −a) +(9−a^2 ))) + (√(9−a^2 )) = 2(√(14)) (√(9−a^2 )) ((√((10(3−a))/(9−a^2 ))) +1)= 2(√(14)) ((10(3−a))/(9−a^2 )) +1 + 2 (√((10(3−a))/(9−a^2 ))) = ((56)/(9−a^2 )) (√((10(3−a))/(9−a^2 ))) = (1/2)[ ((56)/(9−a^2 )) − ((10(3−a))/(9−a^2 )) −1]_(making it as one term) ^(No usefull with (3−a)(3+a)) 10 = (3+a)^ × S_2 ^( 2) ^↗ etc... etc until we get rid of the root To face another complicated problems 40= (((a^2 + 10a +17)^2 )/((3−a)^2 (3+a))) ∼ Boriiiing 40= (([(a+5)^2 −8]^2 )/((3−a)^2 (3+a))) Hfff](Q182687.png)
Answered by Rasheed.Sindhi last updated on 13/Dec/22

Commented by Acem last updated on 13/Dec/22
Commented by Rasheed.Sindhi last updated on 13/Dec/22

Answered by Rasheed.Sindhi last updated on 13/Dec/22
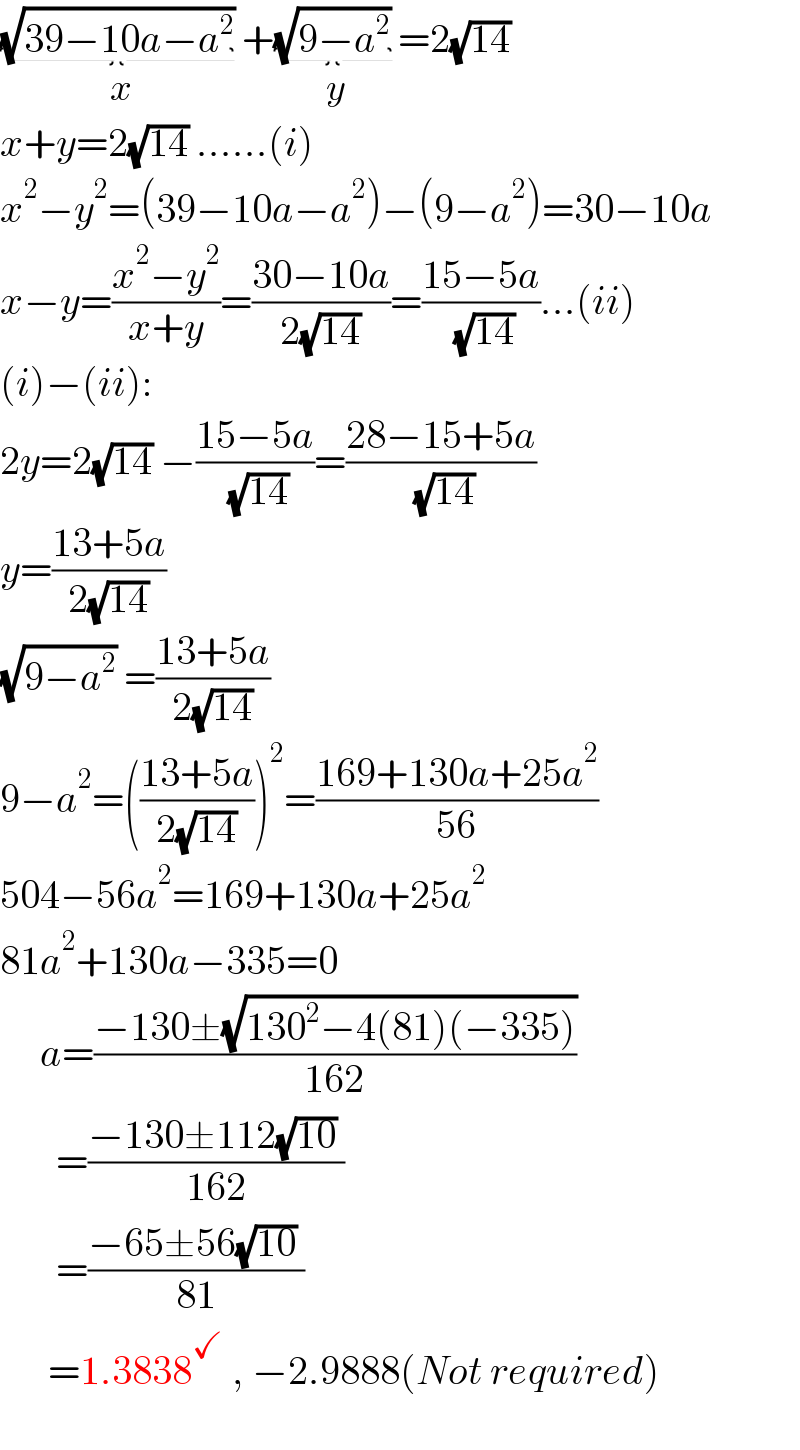
Commented by Acem last updated on 13/Dec/22
Commented by manxsol last updated on 13/Dec/22

Commented by manxsol last updated on 13/Dec/22
Commented by manxsol last updated on 13/Dec/22
Answered by mr W last updated on 14/Dec/22
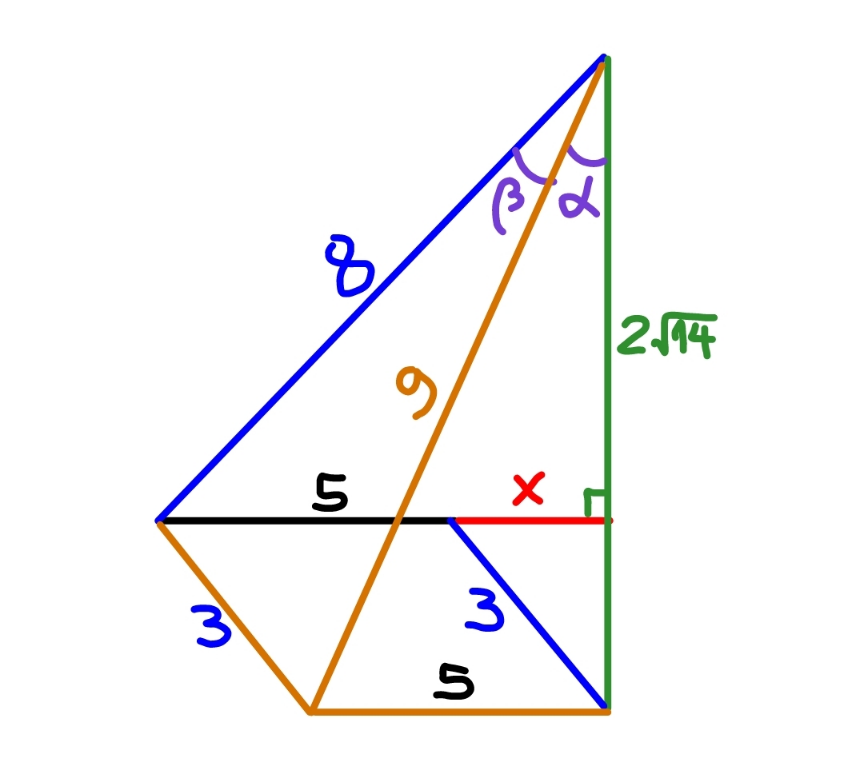
Commented by mr W last updated on 13/Dec/22
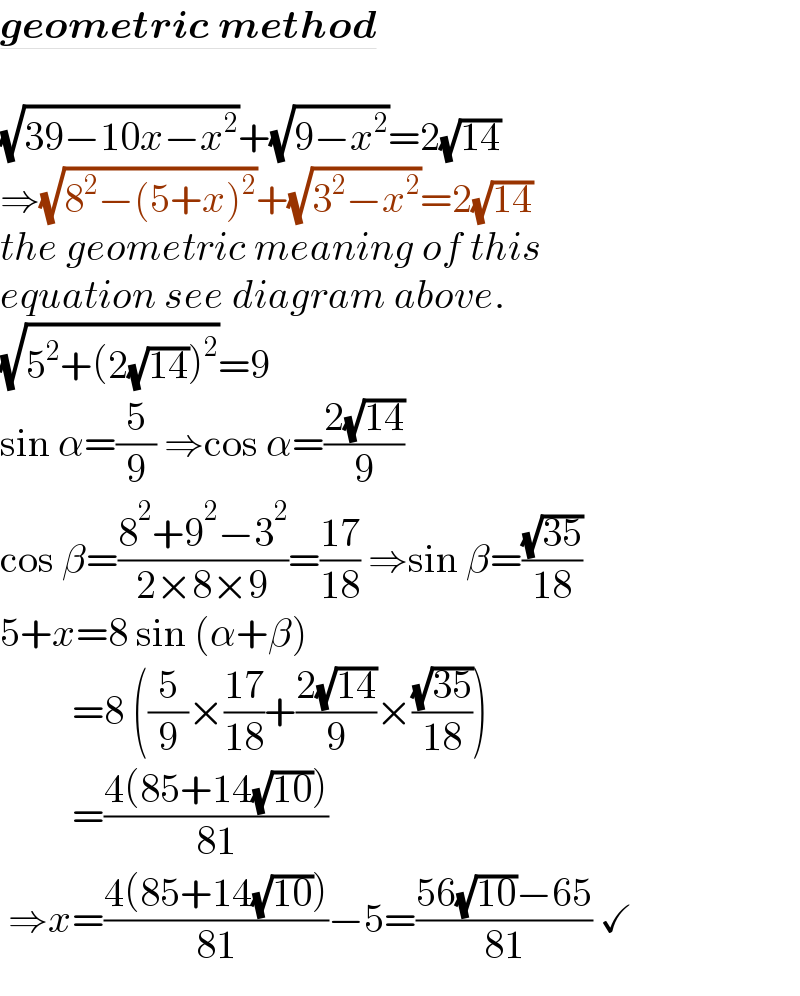
Commented by manxsol last updated on 14/Dec/22

