Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 1828 by Filup last updated on 07/Oct/15

Solve for a and explain your working a=e^a

$$\mathrm{Solve}\:\mathrm{for}\:{a}\:\mathrm{and}\:\mathrm{explain}\:\mathrm{your}\:\mathrm{working} \\ $$$${a}={e}^{{a}} \\ $$

Commented by Alejandro Prieto last updated on 14/Oct/15

Gra^� ficamente podri^� a verse que las funciones f(x) = e^x y g(x) = x no intersectan en ningu^� n punto. Por tanto, puede pensarse que no existen soluciones en R.

$$\mathrm{Gr}\acute {\mathrm{a}ficamente}\:\mathrm{podr}\acute {\mathrm{i}a}\:\mathrm{verse}\:\mathrm{que}\:\mathrm{las}\:\mathrm{funciones} \\ $$$${f}\left({x}\right)\:=\:{e}^{{x}} \:\mathrm{y}\:{g}\left({x}\right)\:=\:{x} \\ $$$$\mathrm{no}\:\mathrm{intersectan}\:\mathrm{en}\:\mathrm{ning}\acute {\mathrm{u}n}\:\mathrm{punto}. \\ $$$$\mathrm{Por}\:\mathrm{tanto},\:\mathrm{puede}\:\mathrm{pensarse}\:\mathrm{que}\:\mathrm{no}\:\mathrm{existen}\:\mathrm{soluciones}\:\mathrm{en}\:\mathbb{R}. \\ $$

Commented by 123456 last updated on 16/Oct/15

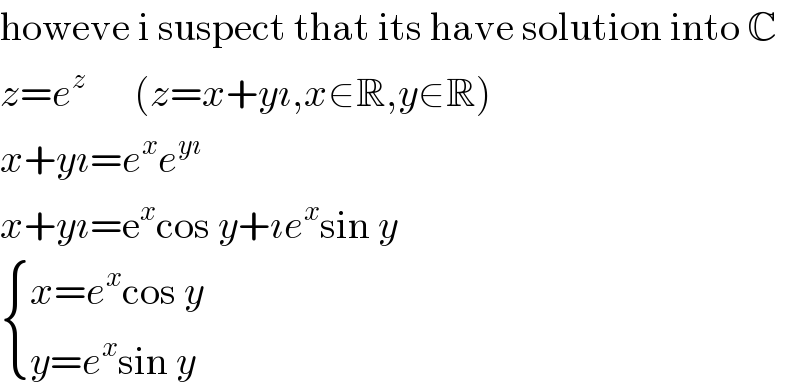
howeve i suspect that its have solution into C z=e^z (z=x+yı,x∈R,y∈R) x+yı=e^x e^(yı) x+yı=e^x cos y+ıe^x sin y { ((x=e^x cos y)),((y=e^x sin y)) :}

$$\mathrm{howeve}\:\mathrm{i}\:\mathrm{suspect}\:\mathrm{that}\:\mathrm{its}\:\mathrm{have}\:\mathrm{solution}\:\mathrm{into}\:\mathbb{C} \\ $$$${z}={e}^{{z}} \:\:\:\:\:\:\left({z}={x}+{y}\imath,{x}\in\mathbb{R},{y}\in\mathbb{R}\right) \\ $$$${x}+{y}\imath={e}^{{x}} {e}^{{y}\imath} \\ $$$${x}+{y}\imath=\mathrm{e}^{{x}} \mathrm{cos}\:{y}+\imath{e}^{{x}} \mathrm{sin}\:{y} \\ $$$$\begin{cases}{{x}={e}^{{x}} \mathrm{cos}\:{y}}\\{{y}={e}^{{x}} \mathrm{sin}\:{y}}\end{cases} \\ $$

Answered by 123456 last updated on 08/Oct/15

f(x)=e^x −x f(x)=0⇔e^x −x=0⇔e^x =x x∈R e^x ≥0 x≤0≤e^x so if it as a real solution it would be at [0,+∞) f(0)=e^0 −0=1≠0 f′(x)=e^x −1 x≥0,e^x ≥1⇒f′(x)≥0 so f is increasing into [0,+∞) lets x>0, by mean value theorem into [0,x],∀ξ∈[0,x] with g(x)=e^x g′(ξ)=((e^x −1)/(x−0)) g′(ξ)=e^ξ ≥1,∀ξ≥0 ((e^x −1)/x)≥1 e^x −1≥x e^x −x−1≥0 f(x)−1≥0 f(x)≥1

$${f}\left({x}\right)={e}^{{x}} −{x} \\ $$$${f}\left({x}\right)=\mathrm{0}\Leftrightarrow{e}^{{x}} −{x}=\mathrm{0}\Leftrightarrow{e}^{{x}} ={x} \\ $$$${x}\in\mathbb{R} \\ $$$${e}^{{x}} \geqslant\mathrm{0} \\ $$$${x}\leqslant\mathrm{0}\leqslant{e}^{{x}} \\ $$$$\mathrm{so}\:\mathrm{if}\:\mathrm{it}\:\mathrm{as}\:\mathrm{a}\:\mathrm{real}\:\mathrm{solution}\:\mathrm{it}\:\mathrm{would}\:\mathrm{be}\:\mathrm{at} \\ $$$$\left[\mathrm{0},+\infty\right) \\ $$$${f}\left(\mathrm{0}\right)={e}^{\mathrm{0}} −\mathrm{0}=\mathrm{1}\neq\mathrm{0} \\ $$$${f}'\left({x}\right)={e}^{{x}} −\mathrm{1} \\ $$$${x}\geqslant\mathrm{0},{e}^{{x}} \geqslant\mathrm{1}\Rightarrow{f}'\left({x}\right)\geqslant\mathrm{0} \\ $$$$\mathrm{so}\:{f}\:\mathrm{is}\:\mathrm{increasing}\:\mathrm{into}\:\left[\mathrm{0},+\infty\right) \\ $$$$\mathrm{lets}\:{x}>\mathrm{0},\:\mathrm{by}\:\mathrm{mean}\:\mathrm{value}\:\mathrm{theorem}\:\mathrm{into} \\ $$$$\left[\mathrm{0},{x}\right],\forall\xi\in\left[\mathrm{0},{x}\right]\:\mathrm{with}\:{g}\left({x}\right)={e}^{{x}} \\ $$$${g}'\left(\xi\right)=\frac{{e}^{{x}} −\mathrm{1}}{{x}−\mathrm{0}} \\ $$$${g}'\left(\xi\right)={e}^{\xi} \geqslant\mathrm{1},\forall\xi\geqslant\mathrm{0} \\ $$$$\frac{{e}^{{x}} −\mathrm{1}}{{x}}\geqslant\mathrm{1} \\ $$$${e}^{{x}} −\mathrm{1}\geqslant{x} \\ $$$${e}^{{x}} −{x}−\mathrm{1}\geqslant\mathrm{0} \\ $$$${f}\left({x}\right)−\mathrm{1}\geqslant\mathrm{0} \\ $$$${f}\left({x}\right)\geqslant\mathrm{1} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com