Question and Answers Forum
Question Number 184240 by ajfour last updated on 04/Jan/23

Commented by ajfour last updated on 04/Jan/23
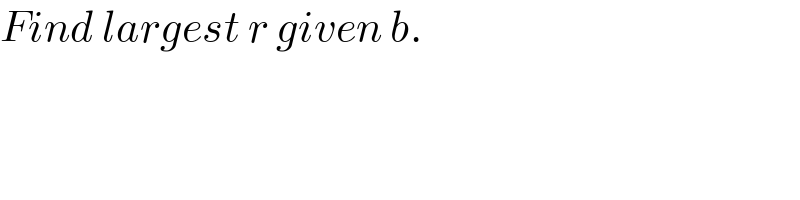
Answered by mr W last updated on 04/Jan/23

Commented by mr W last updated on 04/Jan/23
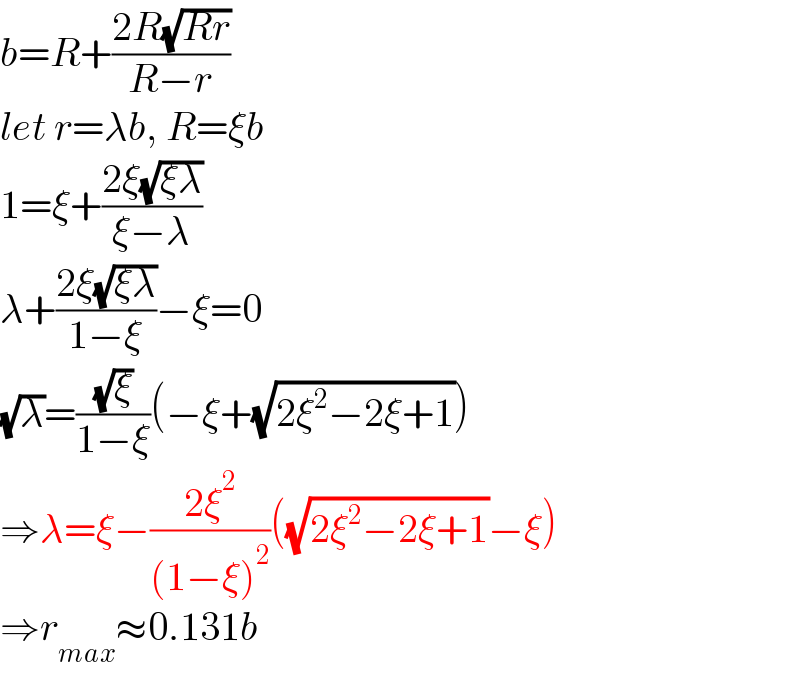
Commented by mr W last updated on 04/Jan/23

Answered by ajfour last updated on 04/Jan/23
−2) =m^2 (m−(1/m))(1+(1/m^2 )) ⇒ (3m^2 −1)(m−(1/m)−2) =(m^2 +1)(m−(1/m)) or 1−(2/((m−(1/m))))=((m^2 +1)/(3(m^2 −(1/3)))) ⇒ (m^2 −1)(m−(1/m))=(3m^2 −1) ⇒ (m^2 −1)^2 =m(3m^2 −1) ⇒ m≈0.6855](Q184254.png)
Commented by mr W last updated on 04/Jan/23