Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 184270 by mathlove last updated on 04/Jan/23

((−64))^(1/6) ∙((−128))^(1/7) =?

$$\sqrt[{\mathrm{6}}]{−\mathrm{64}}\centerdot\sqrt[{\mathrm{7}}]{−\mathrm{128}}=? \\ $$

Answered by HeferH last updated on 04/Jan/23

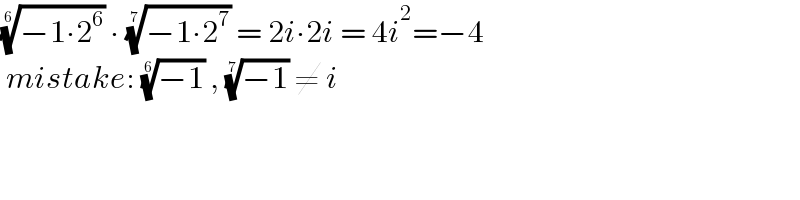
((−1∙2^6 ))^(1/6) ∙ ((−1∙2^7 ))^(1/7) = 2i∙2i = 4i^2 =−4 mistake: ((−1))^(1/6) , ((−1))^(1/7) ≠ i

$$\sqrt[{\mathrm{6}}]{−\mathrm{1}\centerdot\mathrm{2}^{\mathrm{6}} }\:\centerdot\:\sqrt[{\mathrm{7}}]{−\mathrm{1}\centerdot\mathrm{2}^{\mathrm{7}} }\:=\:\mathrm{2}{i}\centerdot\mathrm{2}{i}\:=\:\mathrm{4}{i}^{\mathrm{2}} =−\mathrm{4} \\ $$$$\:{mistake}:\:\sqrt[{\mathrm{6}}]{−\mathrm{1}}\:,\:\sqrt[{\mathrm{7}}]{−\mathrm{1}}\:\neq\:{i} \\ $$

Commented by TheSupreme last updated on 04/Jan/23

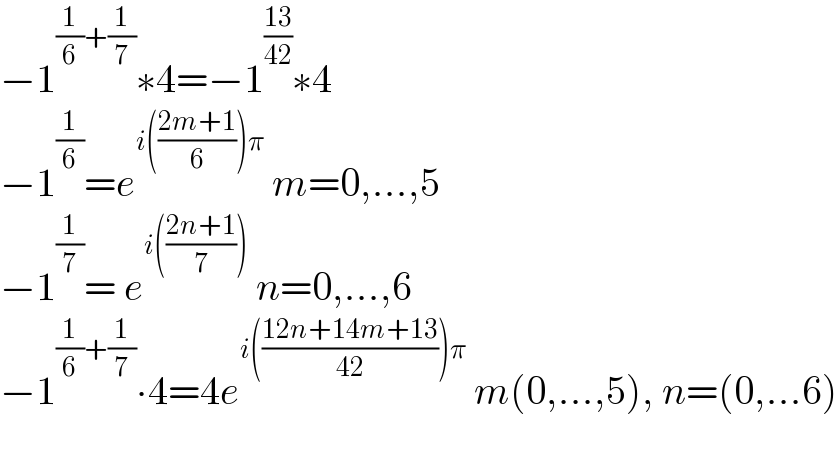
−1^((1/6)+(1/7)) ∗4=−1^((13)/(42)) ∗4 −1^(1/6) =e^(i(((2m+1)/6))π) m=0,...,5 −1^(1/7) = e^(i(((2n+1)/7))) n=0,...,6 −1^((1/6)+(1/7)) ∙4=4e^(i(((12n+14m+13)/(42)))π) m(0,...,5), n=(0,...6)

$$−\mathrm{1}^{\frac{\mathrm{1}}{\mathrm{6}}+\frac{\mathrm{1}}{\mathrm{7}}} \ast\mathrm{4}=−\mathrm{1}^{\frac{\mathrm{13}}{\mathrm{42}}} \ast\mathrm{4} \\ $$$$−\mathrm{1}^{\frac{\mathrm{1}}{\mathrm{6}}} ={e}^{{i}\left(\frac{\mathrm{2}{m}+\mathrm{1}}{\mathrm{6}}\right)\pi} \:{m}=\mathrm{0},...,\mathrm{5} \\ $$$$−\mathrm{1}^{\frac{\mathrm{1}}{\mathrm{7}}} =\:{e}^{{i}\left(\frac{\mathrm{2}{n}+\mathrm{1}}{\mathrm{7}}\right)} \:{n}=\mathrm{0},...,\mathrm{6} \\ $$$$−\mathrm{1}^{\frac{\mathrm{1}}{\mathrm{6}}+\frac{\mathrm{1}}{\mathrm{7}}} \centerdot\mathrm{4}=\mathrm{4}{e}^{{i}\left(\frac{\mathrm{12}{n}+\mathrm{14}{m}+\mathrm{13}}{\mathrm{42}}\right)\pi} \:{m}\left(\mathrm{0},...,\mathrm{5}\right),\:{n}=\left(\mathrm{0},...\mathrm{6}\right) \\ $$$$ \\ $$

Commented by Frix last updated on 04/Jan/23

((−1))^(1/6) =e^(i(π/6)) =((√3)/2)+(1/2)i ((−1))^(1/7) =e^(i(π/7)) =cos (π/7) +i sin (π/7)

$$\sqrt[{\mathrm{6}}]{−\mathrm{1}}=\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{6}}} =\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{i} \\ $$$$\sqrt[{\mathrm{7}}]{−\mathrm{1}}=\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{7}}} =\mathrm{cos}\:\frac{\pi}{\mathrm{7}}\:+\mathrm{i}\:\mathrm{sin}\:\frac{\pi}{\mathrm{7}} \\ $$

Answered by Frix last updated on 04/Jan/23

Complex roots are unique: ((re^(iθ) ))^(1/n) =(r)^(1/n) e^(i(θ/n)) −64=64e^(iπ) x= ((64e^(iπ) ))^(1/6) =((64))^(1/6) e^(i(π/6)) =2e^(i(π/6)) −128=128e^(iπ) y= ((128e^(iπ) ))^(1/7) =((128))^(1/7) e^(i(π/7)) =2e^(i(π/7)) xy=4e^(iπ((1/6)+(1/7))) =4e^(i((13π)/(42))) = =4sin ((4π)/(21)) +4i cos ((4π)/(21)) ≈2.25328+3.30496i

$$\mathrm{Complex}\:\mathrm{roots}\:\mathrm{are}\:\mathrm{unique}:\:\sqrt[{{n}}]{{r}\mathrm{e}^{\mathrm{i}\theta} }=\sqrt[{{n}}]{{r}}\mathrm{e}^{\mathrm{i}\frac{\theta}{{n}}} \\ $$$$−\mathrm{64}=\mathrm{64e}^{\mathrm{i}\pi} \:\:\:\:{x}=\:\sqrt[{\mathrm{6}}]{\mathrm{64e}^{\mathrm{i}\pi} }=\sqrt[{\mathrm{6}}]{\mathrm{64}}\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{6}}} =\mathrm{2e}^{\mathrm{i}\frac{\pi}{\mathrm{6}}} \\ $$$$−\mathrm{128}=\mathrm{128e}^{\mathrm{i}\pi} \:\:\:\:{y}=\:\sqrt[{\mathrm{7}}]{\mathrm{128e}^{\mathrm{i}\pi} }=\sqrt[{\mathrm{7}}]{\mathrm{128}}\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{7}}} =\mathrm{2e}^{\mathrm{i}\frac{\pi}{\mathrm{7}}} \\ $$$${xy}=\mathrm{4e}^{\mathrm{i}\pi\left(\frac{\mathrm{1}}{\mathrm{6}}+\frac{\mathrm{1}}{\mathrm{7}}\right)} =\mathrm{4e}^{\mathrm{i}\frac{\mathrm{13}\pi}{\mathrm{42}}} = \\ $$$$=\mathrm{4sin}\:\frac{\mathrm{4}\pi}{\mathrm{21}}\:+\mathrm{4i}\:\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{21}}\:\approx\mathrm{2}.\mathrm{25328}+\mathrm{3}.\mathrm{30496i} \\ $$

Commented by TheSupreme last updated on 05/Jan/23

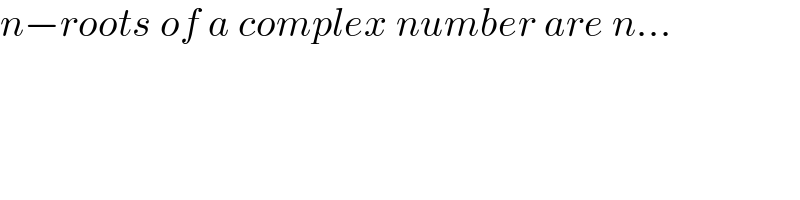
n−roots of a complex number are n...

$${n}−{roots}\:{of}\:{a}\:{complex}\:{number}\:{are}\:{n}... \\ $$

Commented by Frix last updated on 05/Jan/23

No. This problem had been explained by Sir MJS before. ∣z∣≥0∨z∈C ∣a+bi∣=(√(a^2 +b^2 ))≥0 Or what have you learned? A simple root is not an equation. An equation might have several roots but each root has exactly one value. (√4)=2 (unique) but x^2 =4 ⇒ x=±(√4) The ± comes from the law of transforming the equation not from“within” the root. Try to solve this and test the solution: (√x)=−2 If you were right we could solve this: (√(x−6))−(√x)=6 x=((49)/4) proof: (√(((49)/4)−6))−(√((49)/4))=(√((25)/4))−(√((49)/4))=±(5/2)∓(7/2)= ={−6, −1, 1, 6} You would need an extra rule saying “(√x) is always positive except when not used in any calculation” which I bet you have never learned before.

$$\mathrm{No}.\:\mathrm{This}\:\mathrm{problem}\:\mathrm{had}\:\mathrm{been}\:\mathrm{explained}\:\mathrm{by} \\ $$$$\mathrm{Sir}\:\mathrm{MJS}\:\mathrm{before}. \\ $$$$\mid{z}\mid\geqslant\mathrm{0}\vee{z}\in\mathbb{C} \\ $$$$\mid{a}+{b}\mathrm{i}\mid=\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }\geqslant\mathrm{0} \\ $$$$\mathrm{Or}\:\mathrm{what}\:\mathrm{have}\:\mathrm{you}\:\mathrm{learned}? \\ $$$$\mathrm{A}\:\mathrm{simple}\:\mathrm{root}\:\mathrm{is}\:\mathrm{not}\:\mathrm{an}\:\mathrm{equation}.\:\mathrm{An}\:\mathrm{equation} \\ $$$$\mathrm{might}\:\mathrm{have}\:\mathrm{several}\:\mathrm{roots}\:\mathrm{but}\:\mathrm{each}\:\mathrm{root} \\ $$$$\mathrm{has}\:\mathrm{exactly}\:\mathrm{one}\:\mathrm{value}. \\ $$$$\sqrt{\mathrm{4}}=\mathrm{2}\:\left(\mathrm{unique}\right)\:\mathrm{but}\:{x}^{\mathrm{2}} =\mathrm{4}\:\Rightarrow\:{x}=\pm\sqrt{\mathrm{4}} \\ $$$$\mathrm{The}\:\pm\:\mathrm{comes}\:\mathrm{from}\:\mathrm{the}\:\mathrm{law}\:\mathrm{of}\:\mathrm{transforming} \\ $$$$\mathrm{the}\:\mathrm{equation}\:\mathrm{not}\:\mathrm{from}``\mathrm{within}''\:\mathrm{the}\:\mathrm{root}. \\ $$$$\mathrm{Try}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{and}\:\mathrm{test}\:\mathrm{the}\:\mathrm{solution}: \\ $$$$\sqrt{{x}}=−\mathrm{2} \\ $$$$\mathrm{If}\:\mathrm{you}\:\mathrm{were}\:\mathrm{right}\:\mathrm{we}\:\mathrm{could}\:\mathrm{solve}\:\mathrm{this}: \\ $$$$\sqrt{{x}−\mathrm{6}}−\sqrt{{x}}=\mathrm{6} \\ $$$${x}=\frac{\mathrm{49}}{\mathrm{4}} \\ $$$$\mathrm{proof}: \\ $$$$\sqrt{\frac{\mathrm{49}}{\mathrm{4}}−\mathrm{6}}−\sqrt{\frac{\mathrm{49}}{\mathrm{4}}}=\sqrt{\frac{\mathrm{25}}{\mathrm{4}}}−\sqrt{\frac{\mathrm{49}}{\mathrm{4}}}=\pm\frac{\mathrm{5}}{\mathrm{2}}\mp\frac{\mathrm{7}}{\mathrm{2}}= \\ $$$$=\left\{−\mathrm{6},\:−\mathrm{1},\:\mathrm{1},\:\mathrm{6}\right\} \\ $$$$\mathrm{You}\:\mathrm{would}\:\mathrm{need}\:\mathrm{an}\:\mathrm{extra}\:\mathrm{rule}\:\mathrm{saying} \\ $$$$``\sqrt{{x}}\:\mathrm{is}\:\mathrm{always}\:\mathrm{positive}\:\mathrm{except}\:\mathrm{when}\:\mathrm{not} \\ $$$$\mathrm{used}\:\mathrm{in}\:\mathrm{any}\:\mathrm{calculation}''\:\mathrm{which}\:\mathrm{I}\:\mathrm{bet}\:\mathrm{you} \\ $$$$\mathrm{have}\:\mathrm{never}\:\mathrm{learned}\:\mathrm{before}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com