
Question and Answers Forum
Question Number 184349 by alcohol last updated on 05/Jan/23
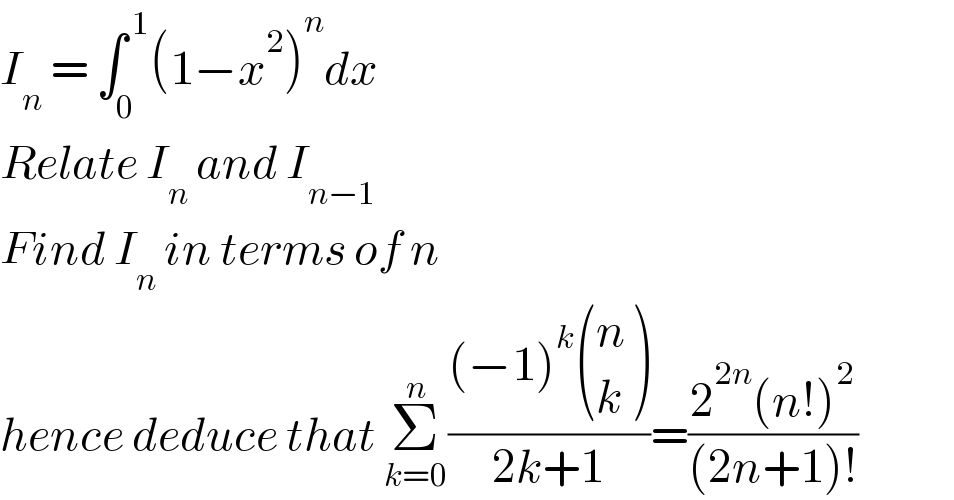
Answered by mr W last updated on 05/Jan/23
![I_n =∫_0 ^1 (1−x^2 )^n dx =[x(1−x^2 )^n ]_0 ^1 +2n∫_0 ^1 x^2 (1−x^2 )^(n−1) dx =2n∫_0 ^1 (1+x^2 −1)(1−x^2 )^(n−1) dx =2n∫_0 ^1 (1−x^2 )^(n−1) dx−2n∫_0 ^1 (1−x^2 )^n dx =2nI_(n−1) −2nI_n ⇒I_n =((2n)/(2n+1))I_(n−1) I_n =((2n)/(2n+1))×((2n−2)/(2n−1))×...×(2/3)×I_0 I_0 =∫_0 ^1 dx=1 ⇒I_n =(((2n)!!)/((2n+1)!!))=(([(2n)!!]^2 )/((2n+1)!))=(([2^n n!]^2 )/((2n+1)!))=((2^(2n) (n!)^2 )/((2n+1)!)) on the other side: I_n =∫_0 ^1 (1−x^2 )^n dx =∫_0 ^1 [Σ_(k=0) ^n ((n),(k) )(−x^2 )^k ]dx =Σ_(k=0) ^n (−1)^k ((n),(k) )[∫_0 ^1 x^(2k) dx] =Σ_(k=0) ^n (−1)^k ((n),(k) )[(x^(2k+1) /(2k+1))]_0 ^1 =Σ_(k=0) ^n (((−1)^k )/(2k+1)) ((n),(k) ) ⇒Σ_(k=0) ^n (((−1)^k )/(2k+1)) ((n),(k) ) =((2^(2n) (n!)^2 )/((2n+1)!))](Q184353.png)
| ||
Question and Answers Forum | ||
Question Number 184349 by alcohol last updated on 05/Jan/23 | ||
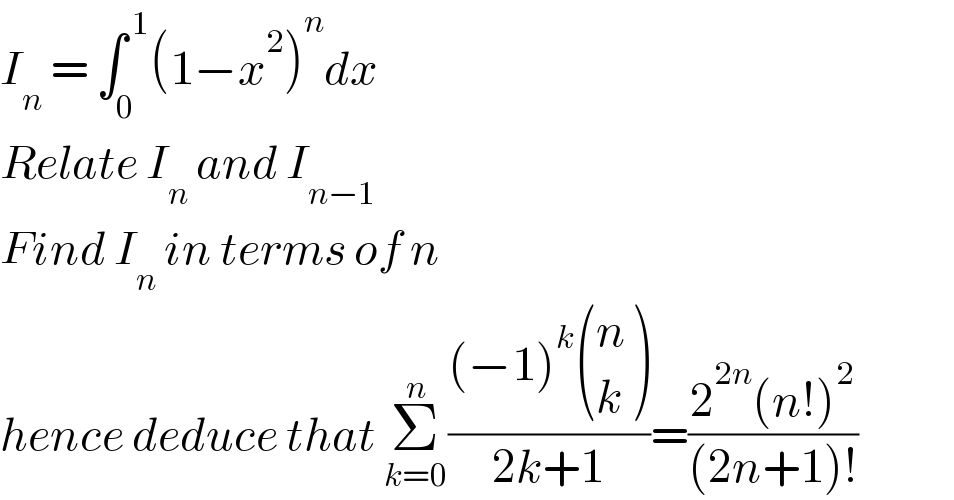 | ||
Answered by mr W last updated on 05/Jan/23 | ||
![I_n =∫_0 ^1 (1−x^2 )^n dx =[x(1−x^2 )^n ]_0 ^1 +2n∫_0 ^1 x^2 (1−x^2 )^(n−1) dx =2n∫_0 ^1 (1+x^2 −1)(1−x^2 )^(n−1) dx =2n∫_0 ^1 (1−x^2 )^(n−1) dx−2n∫_0 ^1 (1−x^2 )^n dx =2nI_(n−1) −2nI_n ⇒I_n =((2n)/(2n+1))I_(n−1) I_n =((2n)/(2n+1))×((2n−2)/(2n−1))×...×(2/3)×I_0 I_0 =∫_0 ^1 dx=1 ⇒I_n =(((2n)!!)/((2n+1)!!))=(([(2n)!!]^2 )/((2n+1)!))=(([2^n n!]^2 )/((2n+1)!))=((2^(2n) (n!)^2 )/((2n+1)!)) on the other side: I_n =∫_0 ^1 (1−x^2 )^n dx =∫_0 ^1 [Σ_(k=0) ^n ((n),(k) )(−x^2 )^k ]dx =Σ_(k=0) ^n (−1)^k ((n),(k) )[∫_0 ^1 x^(2k) dx] =Σ_(k=0) ^n (−1)^k ((n),(k) )[(x^(2k+1) /(2k+1))]_0 ^1 =Σ_(k=0) ^n (((−1)^k )/(2k+1)) ((n),(k) ) ⇒Σ_(k=0) ^n (((−1)^k )/(2k+1)) ((n),(k) ) =((2^(2n) (n!)^2 )/((2n+1)!))](Q184353.png) | ||
| ||