
Question and Answers Forum
Question Number 184796 by Spillover last updated on 11/Jan/23
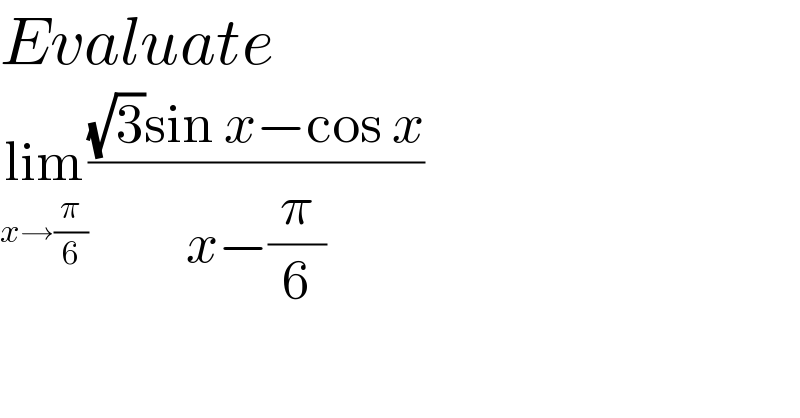
Commented by MJS_new last updated on 11/Jan/23

Commented by MJS_new last updated on 11/Jan/23

Answered by aba last updated on 12/Jan/23

| ||
Question and Answers Forum | ||
Question Number 184796 by Spillover last updated on 11/Jan/23 | ||
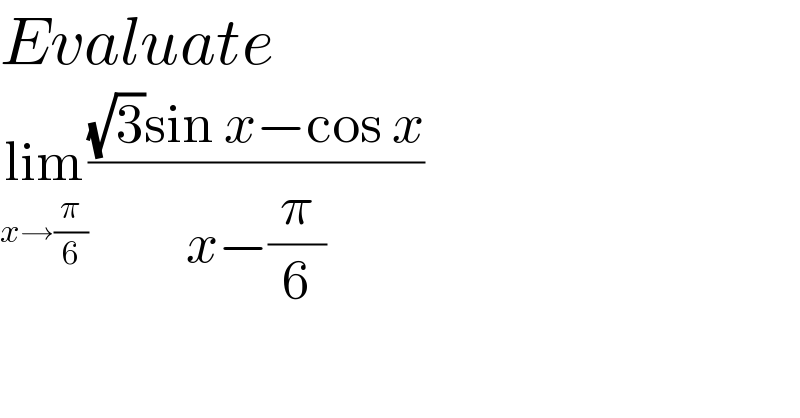 | ||
Commented by MJS_new last updated on 11/Jan/23 | ||
 | ||
Commented by MJS_new last updated on 11/Jan/23 | ||
 | ||
Answered by aba last updated on 12/Jan/23 | ||
 | ||
| ||