Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 185008 by paul2222 last updated on 15/Jan/23

Answered by witcher3 last updated on 26/Jan/23

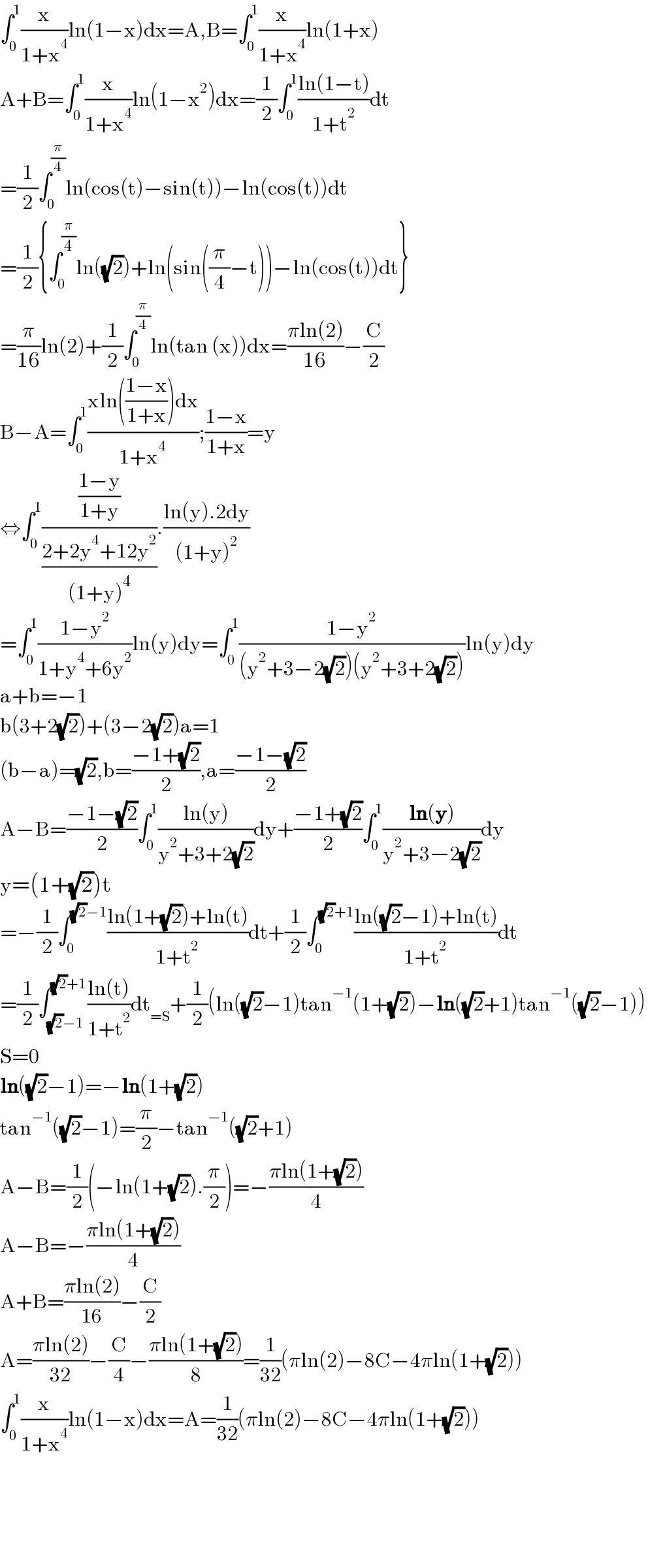
∫_0 ^1 (x/(1+x^4 ))ln(1−x)dx=A,B=∫_0 ^1 (x/(1+x^4 ))ln(1+x) A+B=∫_0 ^1 (x/(1+x^4 ))ln(1−x^2 )dx=(1/2)∫_0 ^1 ((ln(1−t))/(1+t^2 ))dt =(1/2)∫_0 ^(π/4) ln(cos(t)−sin(t))−ln(cos(t))dt =(1/2){∫_0 ^(π/4) ln((√2))+ln(sin((π/4)−t))−ln(cos(t))dt} =(π/(16))ln(2)+(1/2)∫_0 ^(π/4) ln(tan (x))dx=((πln(2))/(16))−(C/2) B−A=∫_0 ^1 ((xln(((1−x)/(1+x)))dx)/(1+x^4 ));((1−x)/(1+x))=y ⇔∫_0 ^1 (((1−y)/(1+y))/((2+2y^4 +12y^2 )/((1+y)^4 ))).((ln(y).2dy)/((1+y)^2 )) =∫_0 ^1 ((1−y^2 )/(1+y^4 +6y^2 ))ln(y)dy=∫_0 ^1 ((1−y^2 )/((y^2 +3−2(√2))(y^2 +3+2(√2))))ln(y)dy a+b=−1 b(3+2(√2))+(3−2(√2))a=1 (b−a)=(√2),b=((−1+(√2))/2),a=((−1−(√2))/2) A−B=((−1−(√2))/2)∫_0 ^1 ((ln(y))/(y^2 +3+2(√2)))dy+((−1+(√2))/2)∫_0 ^1 ((ln(y))/(y^2 +3−2(√2)))dy y=(1+(√2))t =−(1/2)∫_0 ^((√2)−1) ((ln(1+(√2))+ln(t))/(1+t^2 ))dt+(1/2)∫_0 ^((√2)+1) ((ln((√2)−1)+ln(t))/(1+t^2 ))dt =(1/2)∫_((√2)−1) ^((√2)+1) ((ln(t))/(1+t^2 ))dt_(=S) +(1/2)(ln((√2)−1)tan^(−1) (1+(√2))−ln((√2)+1)tan^(−1) ((√2)−1)) S=0 ln((√2)−1)=−ln(1+(√2)) tan^(−1) ((√2)−1)=(π/2)−tan^(−1) ((√2)+1) A−B=(1/2)(−ln(1+(√2)).(π/2))=−((πln(1+(√2)))/4) A−B=−((πln(1+(√2)))/4) A+B=((πln(2))/(16))−(C/2) A=((πln(2))/(32))−(C/4)−((πln(1+(√2)))/8)=(1/(32))(πln(2)−8C−4πln(1+(√2))) ∫_0 ^1 (x/(1+x^4 ))ln(1−x)dx=A=(1/(32))(πln(2)−8C−4πln(1+(√2)))

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{ln}\left(\mathrm{1}−\mathrm{x}\right)\mathrm{dx}=\mathrm{A},\mathrm{B}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{ln}\left(\mathrm{1}+\mathrm{x}\right) \\ $$$$\mathrm{A}+\mathrm{B}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{ln}\left(\mathrm{1}−\mathrm{x}^{\mathrm{2}} \right)\mathrm{dx}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{1}−\mathrm{t}\right)}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \mathrm{ln}\left(\mathrm{cos}\left(\mathrm{t}\right)−\mathrm{sin}\left(\mathrm{t}\right)\right)−\mathrm{ln}\left(\mathrm{cos}\left(\mathrm{t}\right)\right)\mathrm{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \mathrm{ln}\left(\sqrt{\mathrm{2}}\right)+\mathrm{ln}\left(\mathrm{sin}\left(\frac{\pi}{\mathrm{4}}−\mathrm{t}\right)\right)−\mathrm{ln}\left(\mathrm{cos}\left(\mathrm{t}\right)\right)\mathrm{dt}\right\} \\ $$$$=\frac{\pi}{\mathrm{16}}\mathrm{ln}\left(\mathrm{2}\right)+\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \mathrm{ln}\left(\mathrm{tan}\:\left(\mathrm{x}\right)\right)\mathrm{dx}=\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{16}}−\frac{\mathrm{C}}{\mathrm{2}} \\ $$$$\mathrm{B}−\mathrm{A}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{xln}\left(\frac{\mathrm{1}−\mathrm{x}}{\mathrm{1}+\mathrm{x}}\right)\mathrm{dx}}{\mathrm{1}+\mathrm{x}^{\mathrm{4}} };\frac{\mathrm{1}−\mathrm{x}}{\mathrm{1}+\mathrm{x}}=\mathrm{y} \\ $$$$\Leftrightarrow\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\frac{\mathrm{1}−\mathrm{y}}{\mathrm{1}+\mathrm{y}}}{\frac{\mathrm{2}+\mathrm{2y}^{\mathrm{4}} +\mathrm{12y}^{\mathrm{2}} }{\left(\mathrm{1}+\mathrm{y}\right)^{\mathrm{4}} }}.\frac{\mathrm{ln}\left(\mathrm{y}\right).\mathrm{2dy}}{\left(\mathrm{1}+\mathrm{y}\right)^{\mathrm{2}} } \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }{\mathrm{1}+\mathrm{y}^{\mathrm{4}} +\mathrm{6y}^{\mathrm{2}} }\mathrm{ln}\left(\mathrm{y}\right)\mathrm{dy}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−\mathrm{y}^{\mathrm{2}} }{\left(\mathrm{y}^{\mathrm{2}} +\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\right)\left(\mathrm{y}^{\mathrm{2}} +\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)}\mathrm{ln}\left(\mathrm{y}\right)\mathrm{dy} \\ $$$$\mathrm{a}+\mathrm{b}=−\mathrm{1} \\ $$$$\mathrm{b}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)+\left(\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\right)\mathrm{a}=\mathrm{1} \\ $$$$\left(\mathrm{b}−\mathrm{a}\right)=\sqrt{\mathrm{2}},\mathrm{b}=\frac{−\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{2}},\mathrm{a}=\frac{−\mathrm{1}−\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\mathrm{A}−\mathrm{B}=\frac{−\mathrm{1}−\sqrt{\mathrm{2}}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{y}\right)}{\mathrm{y}^{\mathrm{2}} +\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{dy}+\frac{−\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\boldsymbol{\mathrm{ln}}\left(\boldsymbol{\mathrm{y}}\right)}{\mathrm{y}^{\mathrm{2}} +\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{dy} \\ $$$$\mathrm{y}=\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)\mathrm{t} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)+\mathrm{ln}\left(\mathrm{t}\right)}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt}+\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}+\mathrm{1}} \frac{\mathrm{ln}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)+\mathrm{ln}\left(\mathrm{t}\right)}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\sqrt{\mathrm{2}}−\mathrm{1}} ^{\sqrt{\mathrm{2}}+\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{t}\right)}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt}_{=\mathrm{S}} +\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{ln}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{1}+\sqrt{\mathrm{2}}\right)−\boldsymbol{\mathrm{ln}}\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)\mathrm{tan}^{−\mathrm{1}} \left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\right) \\ $$$$\mathrm{S}=\mathrm{0} \\ $$$$\boldsymbol{\mathrm{ln}}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)=−\boldsymbol{\mathrm{ln}}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right) \\ $$$$\mathrm{tan}^{−\mathrm{1}} \left(\sqrt{\mathrm{2}}−\mathrm{1}\right)=\frac{\pi}{\mathrm{2}}−\mathrm{tan}^{−\mathrm{1}} \left(\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$$\mathrm{A}−\mathrm{B}=\frac{\mathrm{1}}{\mathrm{2}}\left(−\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right).\frac{\pi}{\mathrm{2}}\right)=−\frac{\pi\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)}{\mathrm{4}} \\ $$$$\mathrm{A}−\mathrm{B}=−\frac{\pi\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)}{\mathrm{4}} \\ $$$$\mathrm{A}+\mathrm{B}=\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{16}}−\frac{\mathrm{C}}{\mathrm{2}} \\ $$$$\mathrm{A}=\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{32}}−\frac{\mathrm{C}}{\mathrm{4}}−\frac{\pi\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)}{\mathrm{8}}=\frac{\mathrm{1}}{\mathrm{32}}\left(\pi\mathrm{ln}\left(\mathrm{2}\right)−\mathrm{8C}−\mathrm{4}\pi\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)\right) \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{ln}\left(\mathrm{1}−\mathrm{x}\right)\mathrm{dx}=\mathrm{A}=\frac{\mathrm{1}}{\mathrm{32}}\left(\pi\mathrm{ln}\left(\mathrm{2}\right)−\mathrm{8C}−\mathrm{4}\pi\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)\right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com