
Question and Answers Forum
Question Number 186253 by ajfour last updated on 02/Feb/23
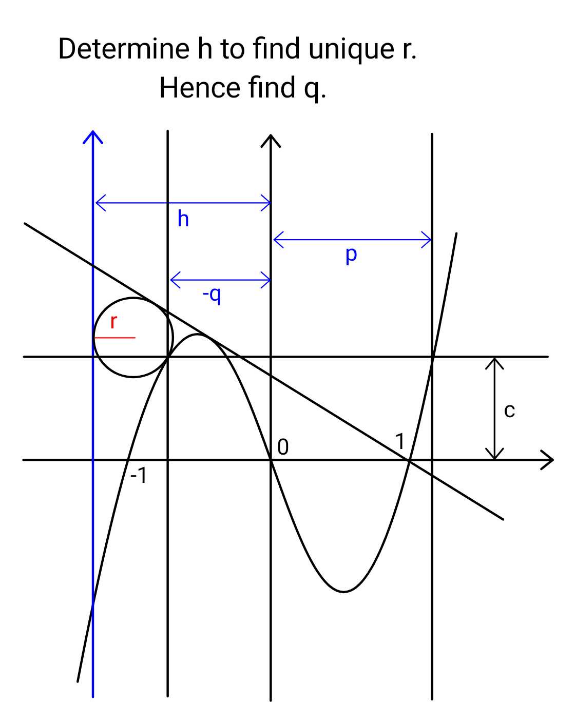
Commented by ajfour last updated on 02/Feb/23
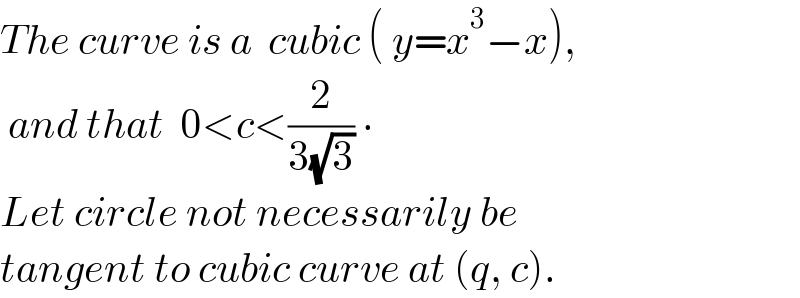
Answered by a.lgnaoui last updated on 04/Feb/23
![equation de droite (D) y=ax+b x=1 y=0 Extremums=(−((√3)/3),+((√3)/3)) conciderons B(−((√3)/3),((2(√3))/9))∈(D) y=((1−(√3))/3)x+(((√3) −1)/3) cercle (C)coupe la ciurbe enC C(−q,q−q^3 ) ,tangente enC est (dy/dx)(x=−q)=3q^2 −1=tan θ r+rcos θ=h−(−q)=h+q r=((h+q)/(1+(1/( (√(1+tan^2 θ))))))=(((h+q)(√(1+tan^2 θ )))/(1+(√(1+tan^2 θ)))) r=(((h+q)(√((q^2 −(1/3))^2 +(1/9))))/( (√((q^2 −(1/3))^2 +(1/9))) +(1/3))) la droite(D)tangente au cercle (C) en C tan ψ=((1−(√3))/3) (x−x_0 )^2 +(y−y_0 )^2 =r^2 y=(√(r^2 −(x−x_0 )^2 )) +y_0 (dy/dx)=((−2(x−x_0 ))/(2(√(r^2 −(x−x_0 )^2 )) +y_0 ))=((1−(√3))/3) (1) x−x_0 =z −6z=(((1−(√3))/3))(y_0 +2(√(r^2 −z^2 )) ) (√(r^2 −z^2 )) =((−9z)/(1−(√3)))−(y_0 /2) r^2 −z^2 =((81z^2 )/((1−(√3) )^2 ))−((9y_0 )/((85−2(√3) )(1−(√3) )))z+(y_0 ^2 /4) [((85−2(√3))/((1−(√(3)^2 ))))]z^2 −((9y_0 )/((1−(√(3)))(85−2(√(3)))))z+(y_0 ^2 /4)−r^2 =0 △=((81y_0 ^2 )/((1−(√3) )^2 (85−2(√3) )^2 ))−((y_0 ^2 −4r^2 )/((1−(√3) )^2 ))(85−2(√3) ) =81y_0 ^2 −(y_0 ^2 −4r^2 )(85−2(√3) )^3 =0 [81−(85−2(√3) )^3 ]y_0^ ^2 = 4r^2 (85−2(√3) )^3 y_0 =((2r(√((85−2(√3) )^3 )))/((85−2(√3) )^3 −81)) z=((9−9(√3))/(2(85−2(√3) )^2 ))y_0 en remplacant dans z en deduire x−x_0 dans l equation de cerxle y=y_0 +(√(r^2 −z^2 )) (1)⇒((1−(√3))/3)=((2r(√((85−2(√3) )^3 )))/((85−2(√3) )^3 −81))+ r(√(1−((81(1−(√3) )^2 )/(2(85−2(√3) )[(85−2(√3) )^3 −81])))) (A suivre)................ after calcul ((1−(√3))/3)≅r=(((h+q)(√((q^2 −(1/3))^2 +(1/9))))/( (√((q^2 −(1/3))^2 +(1/9))) +(1/3))) (h+q)(√((q^2 −(1/3))+(1/9)))=(((1−(√3))/3))[(√((q^2 −(1/3))^2 +(1/9))) +(1/3)] h+q=(((1−(√3))/3))(((√((q^2 −(1/3))^2 +(1/9))) +(1/3))/( (√((q^2 −(1/3))^2 +(1/9))))) h=(((1−(√3))/3))(((√((q^2 −(1/3))^2 +(1/9))) +(1/3))/( (√((q^2 −(1/3))^2 +(1/9)))))−q h=((1−(√3))/3)(1+(1/(3×(√((q^2 −(1/3))^2 +(1/9))))))−q h=((1−(√3))/3)+((1−(√3))/( (√((9q^2 −3)^2 +9)) ))−q ∣h+q∣=(((√3) −1)/3)+(((√3) −1)/( (√((9q^2 −3)^2 +9)) )) h+q=r+(r/( (√(1+tanθ^2 ))))=r(1+(1/( (√(1+(((1−(√3))/3))^2 ))))) h+q=r+((3r)/( (√(13−2(√3))))) (((√3) −1)/( (√(13−2(√3)))))=(((√3) −1)/( (√((9q^2 −3)^2 +9)))) (9q^2 −3)^2 =4−2(√3) q^2 =(((√(4−2(√3) )) +3)/9) q=((√((√(4−2(√3) )) +3))/3)=1,244 donc ∣h∣=1,18 r=(((√3) −1)/3)=0,244](Q186377.png)
Commented by ajfour last updated on 04/Feb/23
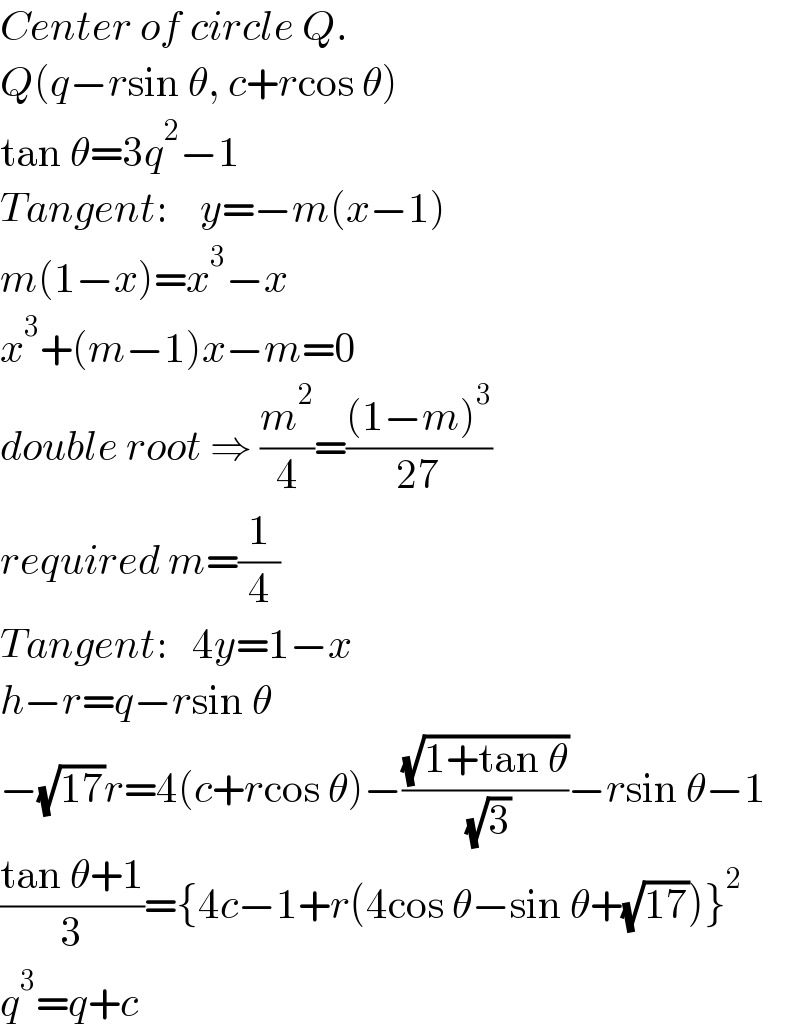
| ||
Question and Answers Forum | ||
Question Number 186253 by ajfour last updated on 02/Feb/23 | ||
 | ||
Commented by ajfour last updated on 02/Feb/23 | ||
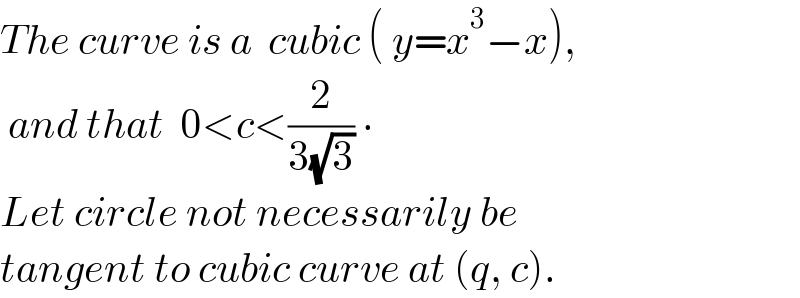 | ||
Answered by a.lgnaoui last updated on 04/Feb/23 | ||
![equation de droite (D) y=ax+b x=1 y=0 Extremums=(−((√3)/3),+((√3)/3)) conciderons B(−((√3)/3),((2(√3))/9))∈(D) y=((1−(√3))/3)x+(((√3) −1)/3) cercle (C)coupe la ciurbe enC C(−q,q−q^3 ) ,tangente enC est (dy/dx)(x=−q)=3q^2 −1=tan θ r+rcos θ=h−(−q)=h+q r=((h+q)/(1+(1/( (√(1+tan^2 θ))))))=(((h+q)(√(1+tan^2 θ )))/(1+(√(1+tan^2 θ)))) r=(((h+q)(√((q^2 −(1/3))^2 +(1/9))))/( (√((q^2 −(1/3))^2 +(1/9))) +(1/3))) la droite(D)tangente au cercle (C) en C tan ψ=((1−(√3))/3) (x−x_0 )^2 +(y−y_0 )^2 =r^2 y=(√(r^2 −(x−x_0 )^2 )) +y_0 (dy/dx)=((−2(x−x_0 ))/(2(√(r^2 −(x−x_0 )^2 )) +y_0 ))=((1−(√3))/3) (1) x−x_0 =z −6z=(((1−(√3))/3))(y_0 +2(√(r^2 −z^2 )) ) (√(r^2 −z^2 )) =((−9z)/(1−(√3)))−(y_0 /2) r^2 −z^2 =((81z^2 )/((1−(√3) )^2 ))−((9y_0 )/((85−2(√3) )(1−(√3) )))z+(y_0 ^2 /4) [((85−2(√3))/((1−(√(3)^2 ))))]z^2 −((9y_0 )/((1−(√(3)))(85−2(√(3)))))z+(y_0 ^2 /4)−r^2 =0 △=((81y_0 ^2 )/((1−(√3) )^2 (85−2(√3) )^2 ))−((y_0 ^2 −4r^2 )/((1−(√3) )^2 ))(85−2(√3) ) =81y_0 ^2 −(y_0 ^2 −4r^2 )(85−2(√3) )^3 =0 [81−(85−2(√3) )^3 ]y_0^ ^2 = 4r^2 (85−2(√3) )^3 y_0 =((2r(√((85−2(√3) )^3 )))/((85−2(√3) )^3 −81)) z=((9−9(√3))/(2(85−2(√3) )^2 ))y_0 en remplacant dans z en deduire x−x_0 dans l equation de cerxle y=y_0 +(√(r^2 −z^2 )) (1)⇒((1−(√3))/3)=((2r(√((85−2(√3) )^3 )))/((85−2(√3) )^3 −81))+ r(√(1−((81(1−(√3) )^2 )/(2(85−2(√3) )[(85−2(√3) )^3 −81])))) (A suivre)................ after calcul ((1−(√3))/3)≅r=(((h+q)(√((q^2 −(1/3))^2 +(1/9))))/( (√((q^2 −(1/3))^2 +(1/9))) +(1/3))) (h+q)(√((q^2 −(1/3))+(1/9)))=(((1−(√3))/3))[(√((q^2 −(1/3))^2 +(1/9))) +(1/3)] h+q=(((1−(√3))/3))(((√((q^2 −(1/3))^2 +(1/9))) +(1/3))/( (√((q^2 −(1/3))^2 +(1/9))))) h=(((1−(√3))/3))(((√((q^2 −(1/3))^2 +(1/9))) +(1/3))/( (√((q^2 −(1/3))^2 +(1/9)))))−q h=((1−(√3))/3)(1+(1/(3×(√((q^2 −(1/3))^2 +(1/9))))))−q h=((1−(√3))/3)+((1−(√3))/( (√((9q^2 −3)^2 +9)) ))−q ∣h+q∣=(((√3) −1)/3)+(((√3) −1)/( (√((9q^2 −3)^2 +9)) )) h+q=r+(r/( (√(1+tanθ^2 ))))=r(1+(1/( (√(1+(((1−(√3))/3))^2 ))))) h+q=r+((3r)/( (√(13−2(√3))))) (((√3) −1)/( (√(13−2(√3)))))=(((√3) −1)/( (√((9q^2 −3)^2 +9)))) (9q^2 −3)^2 =4−2(√3) q^2 =(((√(4−2(√3) )) +3)/9) q=((√((√(4−2(√3) )) +3))/3)=1,244 donc ∣h∣=1,18 r=(((√3) −1)/3)=0,244](Q186377.png) | ||
| ||
Commented by ajfour last updated on 04/Feb/23 | ||
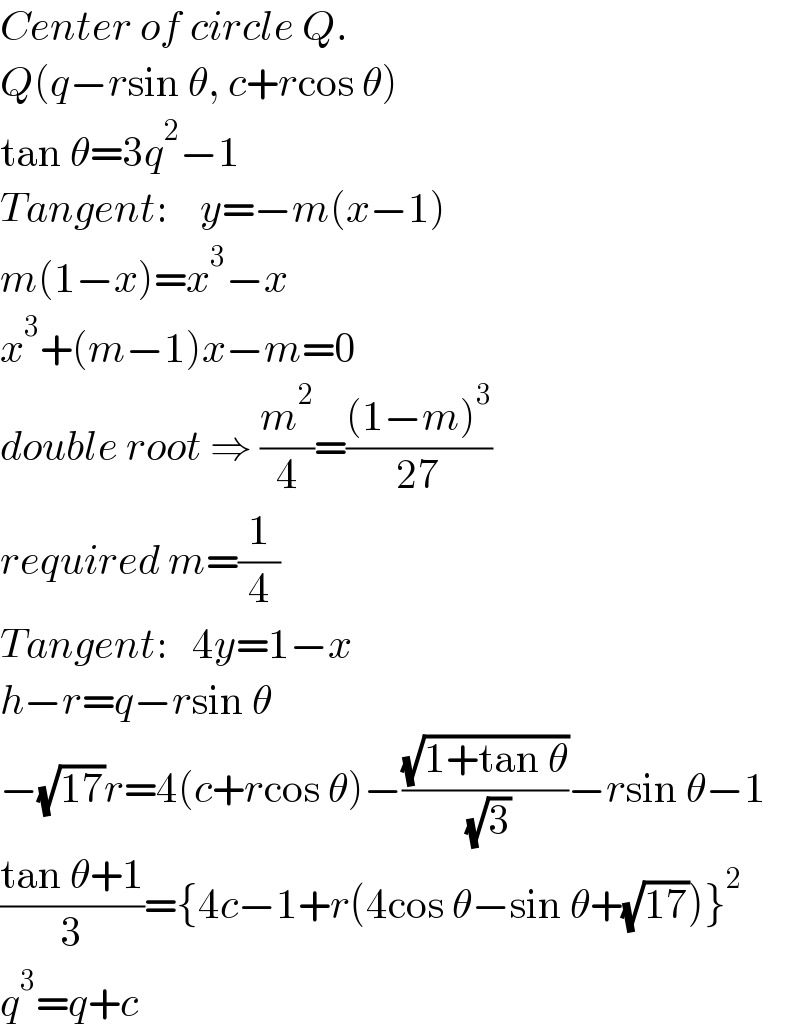 | ||