Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 19245 by Tinkutara last updated on 07/Aug/17

Let f(x) be a quadratic polynomial with integer coefficients such that f(0) and f(1) are odd integers. Prove that the equation f(x) = 0 does not have an integer solution.

$$\mathrm{Let}\:{f}\left({x}\right)\:\mathrm{be}\:\mathrm{a}\:\mathrm{quadratic}\:\mathrm{polynomial} \\ $$$$\mathrm{with}\:\mathrm{integer}\:\mathrm{coefficients}\:\mathrm{such}\:\mathrm{that}\:{f}\left(\mathrm{0}\right) \\ $$$$\mathrm{and}\:{f}\left(\mathrm{1}\right)\:\mathrm{are}\:\mathrm{odd}\:\mathrm{integers}.\:\mathrm{Prove}\:\mathrm{that} \\ $$$$\mathrm{the}\:\mathrm{equation}\:{f}\left({x}\right)\:=\:\mathrm{0}\:\mathrm{does}\:\mathrm{not}\:\mathrm{have}\:\mathrm{an} \\ $$$$\mathrm{integer}\:\mathrm{solution}. \\ $$

Commented by RasheedSindhi last updated on 08/Aug/17

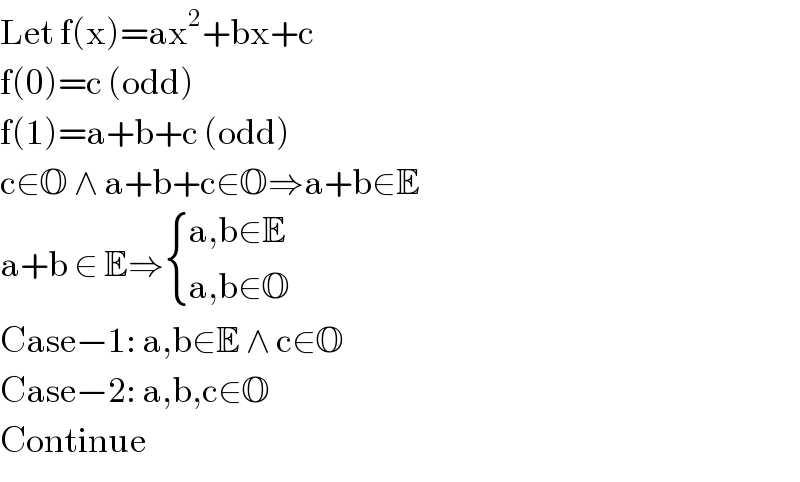
Let f(x)=ax^2 +bx+c f(0)=c (odd) f(1)=a+b+c (odd) c∈O ∧ a+b+c∈O⇒a+b∈E a+b ∈ E⇒ { ((a,b∈E)),((a,b∈O)) :} Case−1: a,b∈E ∧ c∈O Case−2: a,b,c∈O Continue

$$\mathrm{Let}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{ax}^{\mathrm{2}} +\mathrm{bx}+\mathrm{c} \\ $$$$\mathrm{f}\left(\mathrm{0}\right)=\mathrm{c}\:\left(\mathrm{odd}\right) \\ $$$$\mathrm{f}\left(\mathrm{1}\right)=\mathrm{a}+\mathrm{b}+\mathrm{c}\:\left(\mathrm{odd}\right) \\ $$$$\mathrm{c}\in\mathbb{O}\:\wedge\:\mathrm{a}+\mathrm{b}+\mathrm{c}\in\mathbb{O}\Rightarrow\mathrm{a}+\mathrm{b}\in\mathbb{E} \\ $$$$\mathrm{a}+\mathrm{b}\:\in\:\mathbb{E}\Rightarrow\begin{cases}{\mathrm{a},\mathrm{b}\in\mathbb{E}}\\{\mathrm{a},\mathrm{b}\in\mathbb{O}}\end{cases} \\ $$$$\mathrm{Case}−\mathrm{1}:\:\mathrm{a},\mathrm{b}\in\mathbb{E}\:\wedge\:\mathrm{c}\in\mathbb{O} \\ $$$$\mathrm{Case}−\mathrm{2}:\:\mathrm{a},\mathrm{b},\mathrm{c}\in\mathbb{O} \\ $$$$\mathrm{Continue} \\ $$

Answered by RasheedSindhi last updated on 09/Aug/17

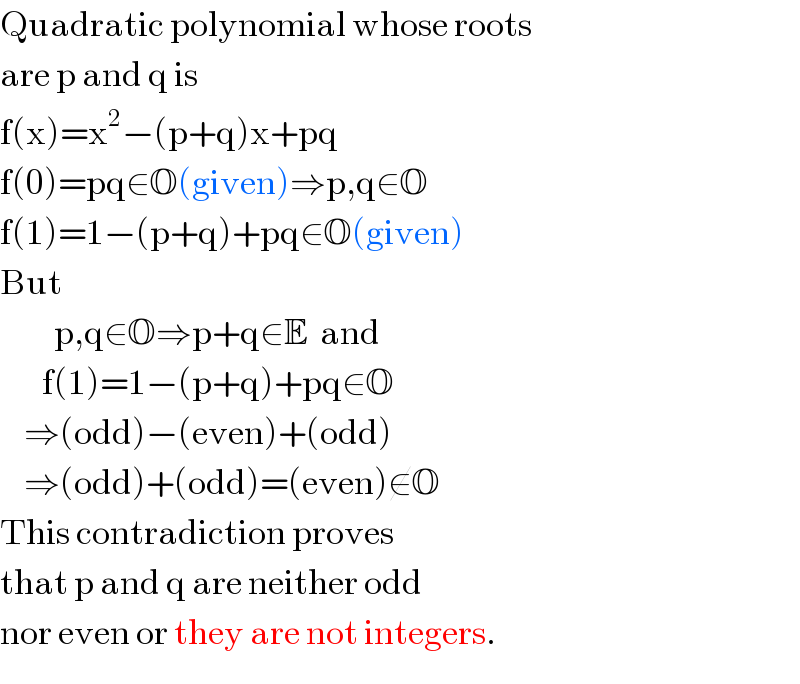
Quadratic polynomial whose roots are p and q is f(x)=x^2 −(p+q)x+pq f(0)=pq∈O(given)⇒p,q∈O f(1)=1−(p+q)+pq∈O(given) But p,q∈O⇒p+q∈E and f(1)=1−(p+q)+pq∈O ⇒(odd)−(even)+(odd) ⇒(odd)+(odd)=(even)∉O This contradiction proves that p and q are neither odd nor even or they are not integers.

$$\mathrm{Quadratic}\:\mathrm{polynomial}\:\mathrm{whose}\:\mathrm{roots} \\ $$$$\mathrm{are}\:\mathrm{p}\:\mathrm{and}\:\mathrm{q}\:\mathrm{is} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} −\left(\mathrm{p}+\mathrm{q}\right)\mathrm{x}+\mathrm{pq} \\ $$$$\mathrm{f}\left(\mathrm{0}\right)=\mathrm{pq}\in\mathbb{O}\left(\mathrm{given}\right)\Rightarrow\mathrm{p},\mathrm{q}\in\mathbb{O} \\ $$$$\mathrm{f}\left(\mathrm{1}\right)=\mathrm{1}−\left(\mathrm{p}+\mathrm{q}\right)+\mathrm{pq}\in\mathbb{O}\left(\mathrm{given}\right) \\ $$$$\mathrm{But} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{p},\mathrm{q}\in\mathbb{O}\Rightarrow\mathrm{p}+\mathrm{q}\in\mathbb{E}\:\:\mathrm{and} \\ $$$$\:\:\:\:\:\:\:\mathrm{f}\left(\mathrm{1}\right)=\mathrm{1}−\left(\mathrm{p}+\mathrm{q}\right)+\mathrm{pq}\in\mathbb{O} \\ $$$$\:\:\:\:\Rightarrow\left(\mathrm{odd}\right)−\left(\mathrm{even}\right)+\left(\mathrm{odd}\right) \\ $$$$\:\:\:\:\Rightarrow\left(\mathrm{odd}\right)+\left(\mathrm{odd}\right)=\left(\mathrm{even}\right)\notin\mathbb{O} \\ $$$$\mathrm{This}\:\mathrm{contradiction}\:\mathrm{proves} \\ $$$$\mathrm{that}\:\mathrm{p}\:\mathrm{and}\:\mathrm{q}\:\mathrm{are}\:\mathrm{neither}\:\mathrm{odd} \\ $$$$\mathrm{nor}\:\mathrm{even}\:\mathrm{or}\:\mathrm{they}\:\mathrm{are}\:\mathrm{not}\:\mathrm{integers}. \\ $$

Commented by Tinkutara last updated on 09/Aug/17

Thank you very much Sir!

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com