
Question and Answers Forum
Question Number 19903 by j.masanja06@gmail.com last updated on 17/Aug/17
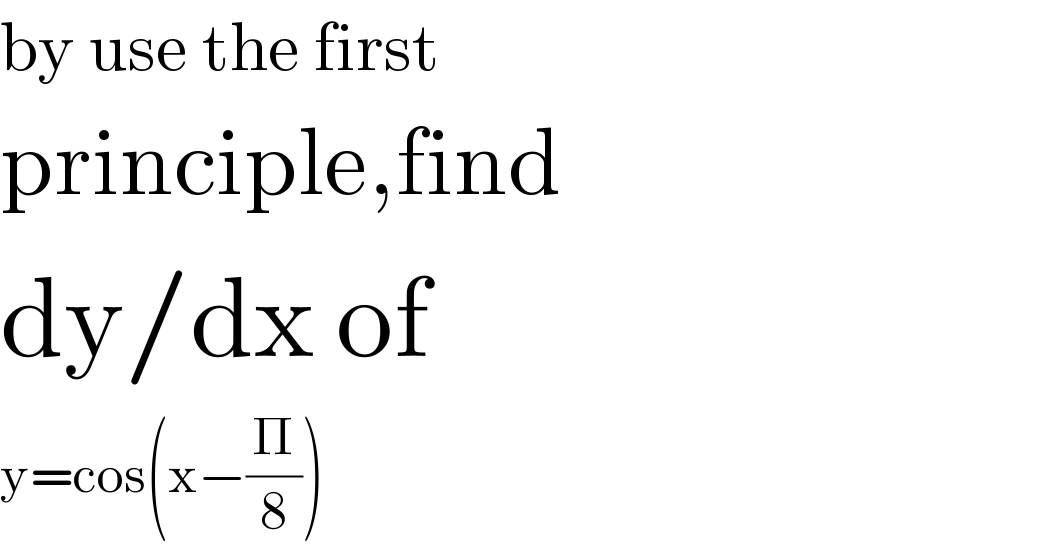
Answered by icyfalcon999 last updated on 18/Aug/17
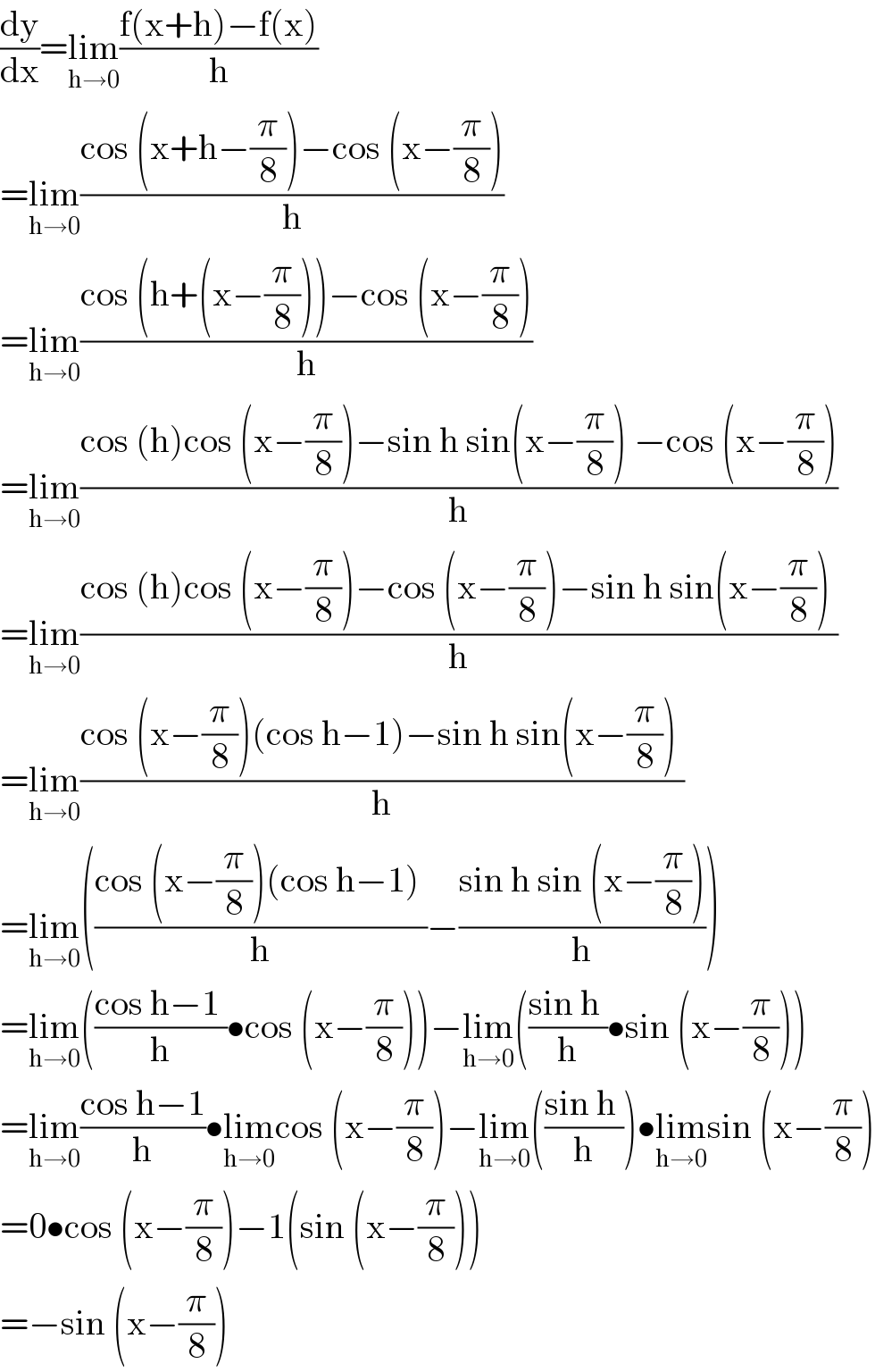
| ||
Question and Answers Forum | ||
Question Number 19903 by j.masanja06@gmail.com last updated on 17/Aug/17 | ||
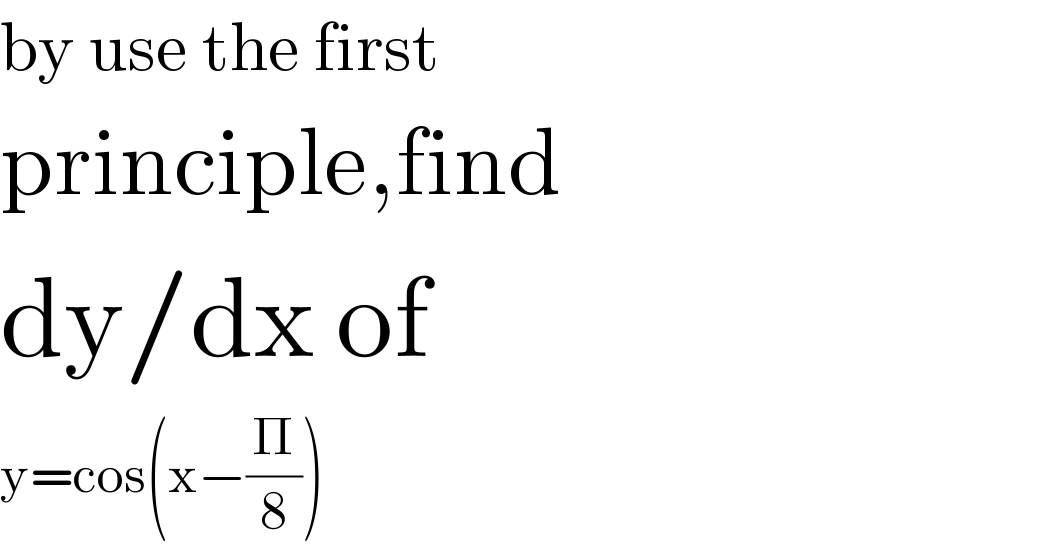 | ||
Answered by icyfalcon999 last updated on 18/Aug/17 | ||
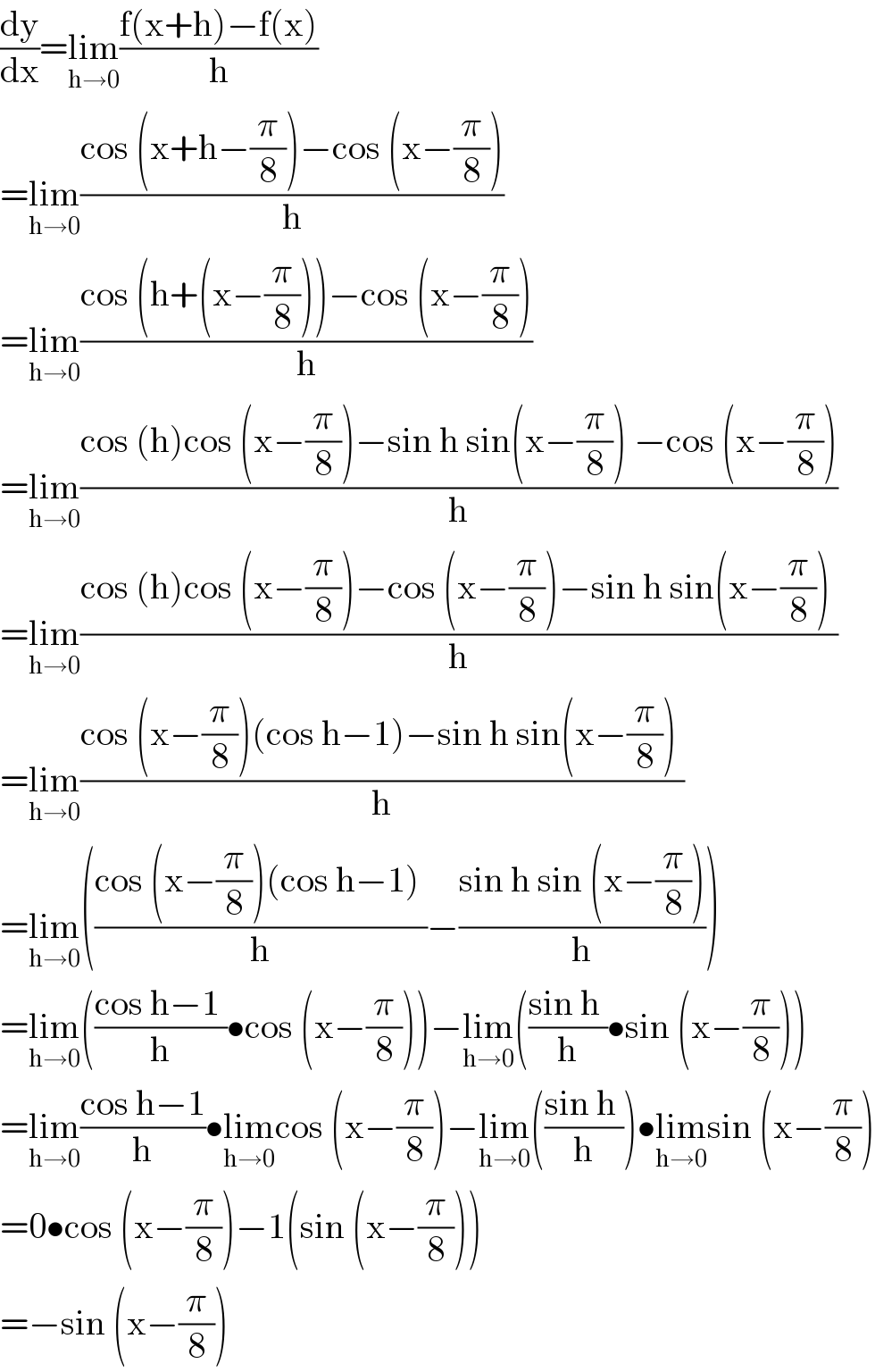 | ||
| ||