Question and Answers Forum

All Questions      Topic List

Mensuration Questions

Previous in All Question      Next in All Question      

Previous in Mensuration      Next in Mensuration      

Question Number 19912 by ajfour last updated on 17/Aug/17

Commented by ajfour last updated on 18/Aug/17

Find tangent of the acute angle between AB and SC and the shortest distance between them. The side of the equilateral triangle is even a. SA is perpendicular to the plane of △ABC.

$${Find}\:{tangent}\:{of}\:{the}\:{acute}\:{angle} \\ $$$${between}\:{AB}\:{and}\:{SC}\:{and}\:{the} \\ $$$${shortest}\:{distance}\:{between}\:{them}. \\ $$$${The}\:{side}\:{of}\:{the}\:{equilateral}\:{triangle} \\ $$$${is}\:{even}\:\boldsymbol{{a}}.\:{SA}\:{is}\:{perpendicular}\:{to} \\ $$$${the}\:{plane}\:{of}\:\bigtriangleup{ABC}. \\ $$

Answered by ajfour last updated on 19/Aug/17

Commented by ajfour last updated on 19/Aug/17

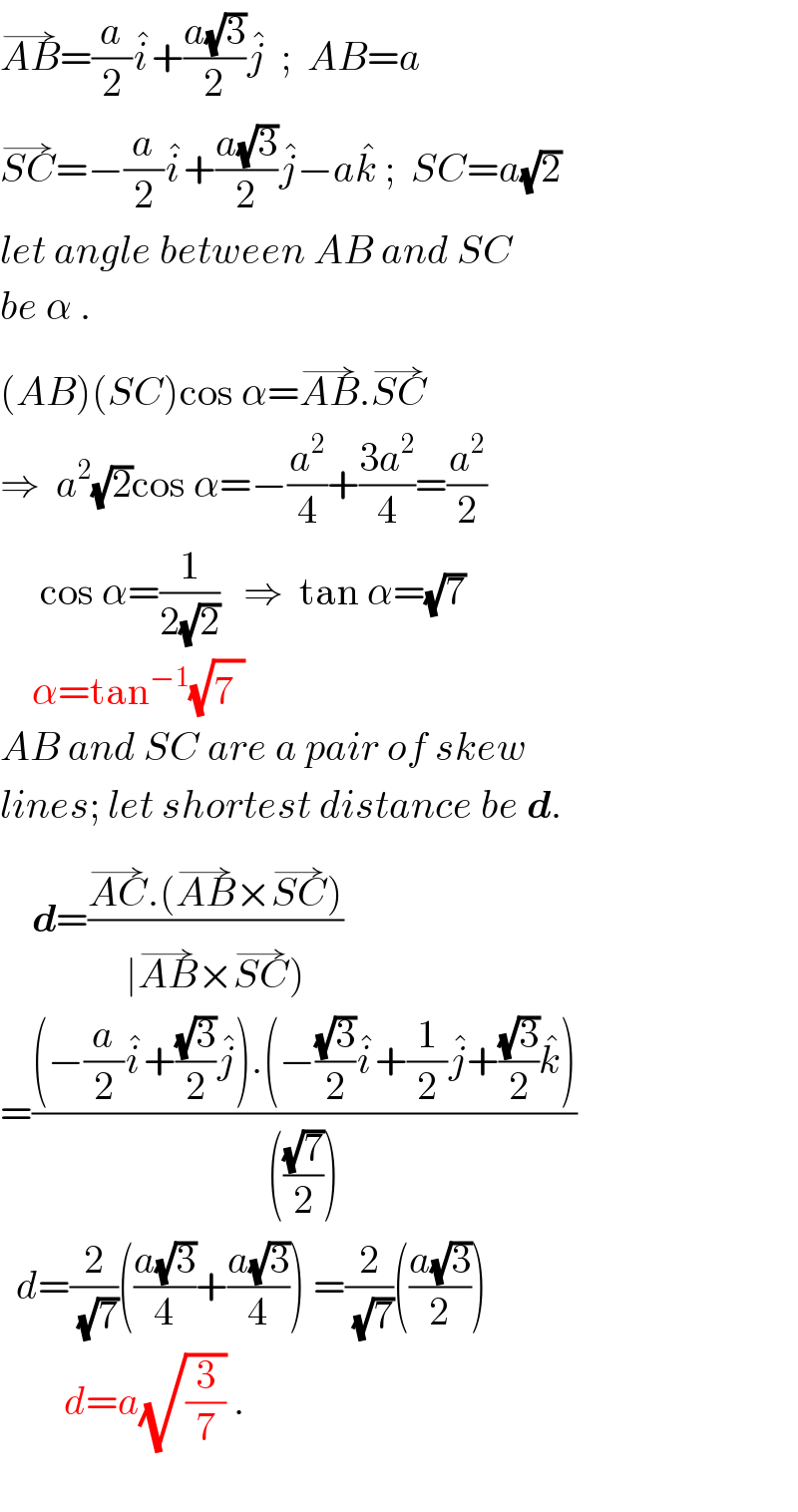
AB^(→) =(a/2)i^� +((a(√3))/2)j^� ; AB=a SC^(→) =−(a/2)i^� +((a(√3))/2)j^� −ak^� ; SC=a(√2) let angle between AB and SC be α . (AB)(SC)cos α=AB^(→) .SC^(→) ⇒ a^2 (√2)cos α=−(a^2 /4)+((3a^2 )/4)=(a^2 /2) cos α=(1/(2(√2))) ⇒ tan α=(√7) α=tan^(−1) (√7^ ) AB and SC are a pair of skew lines; let shortest distance be d. d=((AC^(→) .(AB^(→) ×SC^(→) ))/(∣AB^(→) ×SC^(→) ))) =(((−(a/2)i^� +((√3)/2)j^� ).(−((√3)/2)i^� +(1/2)j^� +((√3)/2)k^� ))/((((√7)/2)))) d=(2/(√7))(((a(√3))/4)+((a(√3))/4)) =(2/(√7))(((a(√3))/2)) d=a(√(3/7)) .

$$\overset{\rightarrow} {{AB}}=\frac{{a}}{\mathrm{2}}\hat {{i}}+\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{2}}\hat {{j}}\:\:;\:\:{AB}={a} \\ $$$$\overset{\rightarrow} {{SC}}=−\frac{{a}}{\mathrm{2}}\hat {{i}}+\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{2}}\hat {{j}}−{a}\hat {{k}}\:;\:\:{SC}={a}\sqrt{\mathrm{2}} \\ $$$${let}\:{angle}\:{between}\:{AB}\:{and}\:{SC}\: \\ $$$${be}\:\alpha\:. \\ $$$$\left({AB}\right)\left({SC}\right)\mathrm{cos}\:\alpha=\overset{\rightarrow} {{AB}}.\overset{\rightarrow} {{SC}} \\ $$$$\Rightarrow\:\:{a}^{\mathrm{2}} \sqrt{\mathrm{2}}\mathrm{cos}\:\alpha=−\frac{{a}^{\mathrm{2}} }{\mathrm{4}}+\frac{\mathrm{3}{a}^{\mathrm{2}} }{\mathrm{4}}=\frac{{a}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\:\:\:\:\:\mathrm{cos}\:\alpha=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\:\:\Rightarrow\:\:\mathrm{tan}\:\alpha=\sqrt{\mathrm{7}}\: \\ $$$$\:\:\:\:\alpha=\mathrm{tan}^{−\mathrm{1}} \sqrt{\mathrm{7}^{} }\: \\ $$$${AB}\:{and}\:{SC}\:{are}\:{a}\:{pair}\:{of}\:{skew} \\ $$$${lines};\:{let}\:{shortest}\:{distance}\:{be}\:\boldsymbol{{d}}. \\ $$$$\:\:\:\:\boldsymbol{{d}}=\frac{\overset{\rightarrow} {{AC}}.\left(\overset{\rightarrow} {{AB}}×\overset{\rightarrow} {{SC}}\right)}{\left.\mid\overset{\rightarrow} {{AB}}×\overset{\rightarrow} {{SC}}\right)} \\ $$$$=\frac{\left(−\frac{{a}}{\mathrm{2}}\hat {{i}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\hat {{j}}\right).\left(−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\hat {{i}}+\frac{\mathrm{1}}{\mathrm{2}}\hat {{j}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\hat {{k}}\right)}{\left(\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}\right)} \\ $$$$\:\:{d}=\frac{\mathrm{2}}{\sqrt{\mathrm{7}}}\left(\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{4}}+\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{4}}\right)\:=\frac{\mathrm{2}}{\sqrt{\mathrm{7}}}\left(\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:\:\:\:{d}={a}\sqrt{\frac{\mathrm{3}}{\mathrm{7}}}\:. \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com