Question Number 2000 by Yozzi last updated on 29/Oct/15
$${Find}\:{a}\:{non}−{constant}\:{function}\:{f}\: \\ $$$${satisfying}\:{f}\left(\mathrm{0}\right)=\mathrm{1},{f}\left(−\mathrm{2}\right)=\mathrm{0}\:{and} \\ $$$${f}\left({x}−{y}\right)={f}\left({x}\right){f}\left({y}\right)−{f}\left(−\mathrm{2}−{x}\right){f}\left({y}−\mathrm{2}\right). \\ $$
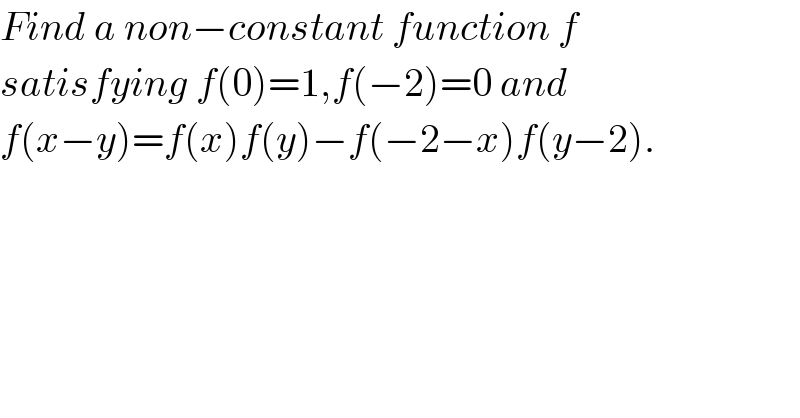
Commented by prakash jain last updated on 29/Oct/15
![x=0 f(−y)=f(0)f(y)−f(−2)f(y−2) ∵f(−2)=0 for the above relation to be true ∀y. f(−y)=f(y) ⇒even function y=x f(0)=[f(x)]^2 −f(−2−x)f(x−2) [f(x)]^2 =1+f(x−2)f(x+2)](Q2019.png)
$${x}=\mathrm{0} \\ $$$${f}\left(−{y}\right)={f}\left(\mathrm{0}\right){f}\left({y}\right)−{f}\left(−\mathrm{2}\right){f}\left({y}−\mathrm{2}\right) \\ $$$$\because{f}\left(−\mathrm{2}\right)=\mathrm{0}\:\mathrm{for}\:\mathrm{the}\:\mathrm{above}\:\mathrm{relation}\:\mathrm{to}\:\mathrm{be} \\ $$$$\mathrm{true}\:\forall{y}. \\ $$$${f}\left(−{y}\right)={f}\left({y}\right) \\ $$$$\Rightarrow{even}\:{function} \\ $$$${y}={x} \\ $$$${f}\left(\mathrm{0}\right)=\left[{f}\left({x}\right)\right]^{\mathrm{2}} −{f}\left(−\mathrm{2}−{x}\right){f}\left({x}−\mathrm{2}\right) \\ $$$$\left[{f}\left({x}\right)\right]^{\mathrm{2}} =\mathrm{1}+{f}\left({x}−\mathrm{2}\right){f}\left({x}+\mathrm{2}\right) \\ $$
Commented by prakash jain last updated on 30/Oct/15
![y=2 f(x−2)=f(x)f(2)−f(x+2)f(0) f(x−2)=−f(x+2) f(x)=−f(x+4) f(x+8)=[f(x+4+4)]=−f(x+4)=f(x) f is periodic with period 8. cos [(π/4)(x−y)]=cos (π/4)x∙cos (π/4)y+sin( (π/4)x)sin ((π/4)y) sin ((πy)/4)=cos (((π(y−2))/4)) sin ((πx)/4)=−cos ((π/2)+(π/4)x)=−cos (((π(−x−2))/4))](Q2024.png)
$${y}=\mathrm{2} \\ $$$${f}\left({x}−\mathrm{2}\right)={f}\left({x}\right){f}\left(\mathrm{2}\right)−{f}\left({x}+\mathrm{2}\right){f}\left(\mathrm{0}\right) \\ $$$${f}\left({x}−\mathrm{2}\right)=−{f}\left({x}+\mathrm{2}\right) \\ $$$${f}\left({x}\right)=−{f}\left({x}+\mathrm{4}\right) \\ $$$${f}\left({x}+\mathrm{8}\right)=\left[{f}\left({x}+\mathrm{4}+\mathrm{4}\right)\right]=−{f}\left({x}+\mathrm{4}\right)={f}\left({x}\right) \\ $$$${f}\:{is}\:{periodic}\:{with}\:{period}\:\mathrm{8}. \\ $$$$\mathrm{cos}\:\left[\frac{\pi}{\mathrm{4}}\left({x}−{y}\right)\right]=\mathrm{cos}\:\frac{\pi}{\mathrm{4}}{x}\centerdot\mathrm{cos}\:\frac{\pi}{\mathrm{4}}{y}+\mathrm{sin}\left(\:\frac{\pi}{\mathrm{4}}{x}\right)\mathrm{sin}\:\left(\frac{\pi}{\mathrm{4}}{y}\right) \\ $$$$\mathrm{sin}\:\frac{\pi{y}}{\mathrm{4}}=\mathrm{cos}\:\left(\frac{\pi\left({y}−\mathrm{2}\right)}{\mathrm{4}}\right) \\ $$$$\mathrm{sin}\:\frac{\pi{x}}{\mathrm{4}}=−\mathrm{cos}\:\left(\frac{\pi}{\mathrm{2}}+\frac{\pi}{\mathrm{4}}{x}\right)=−\mathrm{cos}\:\left(\frac{\pi\left(−{x}−\mathrm{2}\right)}{\mathrm{4}}\right) \\ $$
Answered by Rasheed Soomro last updated on 02/Nov/15
![Find a non−constant function f satisfying f(0)=1,f(−2)=0 and f(x−y)=f(x)f(y)−f(−2−x)f(y−2)...........(1) −−−−−−×××−−−−−−− x=−2,y=x f(−2−x)=f(−2)f(x)−f(−2−{−2})f(x−2) f(−2−x)=0.f(x)−f(0)f(x−2)=−f(x−2) f(−2−x)=−f(x−2)=−{f(x)f(2)−f(−2−x)f(2−2)} =−{f(x)f(2)−f(−2−x)f(0)}=f(−x−2)−f(2)f(x) f(−x−2)−f(−x−2)=−f(2)f(x) f(2)=0 ∣ f(x)=0 [But this not non−constant] ∗∗∗ Substituting y=0 f(x)=f(x)f(0)−f(−2−x)f(−2) =f(x) This means f(x) is arbitrary if y=0. x=−2−x f(−2−x−y)=f(−2−x)f(y)−f(−2−{}) continue](Q2010.png)
$${Find}\:{a}\:{non}−{constant}\:{function}\:{f}\: \\ $$$${satisfying}\:{f}\left(\mathrm{0}\right)=\mathrm{1},{f}\left(−\mathrm{2}\right)=\mathrm{0}\:{and} \\ $$$${f}\left({x}−{y}\right)={f}\left({x}\right){f}\left({y}\right)−{f}\left(−\mathrm{2}−{x}\right){f}\left({y}−\mathrm{2}\right)...........\left(\mathrm{1}\right) \\ $$$$−−−−−−×××−−−−−−− \\ $$$${x}=−\mathrm{2},{y}={x} \\ $$$${f}\left(−\mathrm{2}−{x}\right)={f}\left(−\mathrm{2}\right){f}\left({x}\right)−{f}\left(−\mathrm{2}−\left\{−\mathrm{2}\right\}\right){f}\left({x}−\mathrm{2}\right) \\ $$$${f}\left(−\mathrm{2}−{x}\right)=\mathrm{0}.{f}\left({x}\right)−{f}\left(\mathrm{0}\right){f}\left({x}−\mathrm{2}\right)=−{f}\left({x}−\mathrm{2}\right) \\ $$$${f}\left(−\mathrm{2}−{x}\right)=−{f}\left({x}−\mathrm{2}\right)=−\left\{{f}\left({x}\right){f}\left(\mathrm{2}\right)−{f}\left(−\mathrm{2}−{x}\right){f}\left(\mathrm{2}−\mathrm{2}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\left\{{f}\left({x}\right){f}\left(\mathrm{2}\right)−{f}\left(−\mathrm{2}−{x}\right){f}\left(\mathrm{0}\right)\right\}={f}\left(−{x}−\mathrm{2}\right)−{f}\left(\mathrm{2}\right){f}\left({x}\right) \\ $$$${f}\left(−{x}−\mathrm{2}\right)−{f}\left(−{x}−\mathrm{2}\right)=−{f}\left(\mathrm{2}\right){f}\left({x}\right) \\ $$$${f}\left(\mathrm{2}\right)=\mathrm{0}\:\mid\:{f}\left({x}\right)=\mathrm{0}\:\:\left[{But}\:{this}\:{not}\:{non}−{constant}\right] \\ $$$$\ast\ast\ast \\ $$$${Substituting}\:{y}=\mathrm{0} \\ $$$${f}\left({x}\right)={f}\left({x}\right){f}\left(\mathrm{0}\right)−{f}\left(−\mathrm{2}−{x}\right){f}\left(−\mathrm{2}\right) \\ $$$$\:\:\:\:\:\:\:\:\:={f}\left({x}\right) \\ $$$${This}\:{means}\:{f}\left({x}\right)\:\:{is}\:{arbitrary}\:{if}\:{y}=\mathrm{0}. \\ $$$${x}=−\mathrm{2}−{x} \\ $$$${f}\left(−\mathrm{2}−{x}−{y}\right)={f}\left(−\mathrm{2}−{x}\right){f}\left({y}\right)−{f}\left(−\mathrm{2}−\left\{\right\}\right) \\ $$$${continue} \\ $$
Answered by prakash jain last updated on 30/Oct/15
$${f}\left({x}\right)=\mathrm{cos}\:\frac{\pi{x}}{\mathrm{4}} \\ $$
Commented by prakash jain last updated on 30/Oct/15
$${see}\:{comments}\:{in}\:{question}\:{for}\:{explaination}. \\ $$