Question Number 20230 by ajfour last updated on 24/Aug/17
Commented by ajfour last updated on 27/Aug/17
$${Find}\:{the}\:{area}\:{of}\:{that}\:{part}\:{of}\:{the} \\ $$$${surface}\:{of}\:{the}\:{sphere}\:: \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} ={a}^{\mathrm{2}} \:{which}\:{is}\:{cut}\:{out}\:{by} \\ $$$${the}\:{surface}\:{of}\:{the}\:{cylinder}\:: \\ $$$$\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1}\:\:\left({a}>{b}\right). \\ $$
Commented by ajfour last updated on 26/Aug/17
$${I}\:{couldn}'{t}\:{solve}\:{this},\:{method}\:{is} \\ $$$${clear}\:{to}\:{me},\:{but}\:{the}\:{integral}\:{i} \\ $$$${cannot}\:{simplify}.\:{mrW}\mathrm{1}\:{Sir}, \\ $$$${please}\:{help}\:{after}\:{i}\:{attach}\:{a}\: \\ $$$${diagram}\:\:{for}\:{its}\:{solution}. \\ $$
Commented by ajfour last updated on 26/Aug/17
Commented by ajfour last updated on 26/Aug/17
$${Let}\:{Area}\:{of}\:{sphere}\:{outside} \\ $$$${cylinder}\:{be}\:{A}. \\ $$$${A}=\mathrm{2}\int_{−\theta_{\mathrm{0}} } ^{\:\:\theta_{\mathrm{0}} } {r}\left(\mathrm{2}\phi\right){ad}\theta \\ $$$${where}\:{a}\mathrm{cos}\:\theta_{\mathrm{0}} ={b} \\ $$$$\:\:\:{r}^{\mathrm{2}} ={x}^{\mathrm{2}} +{y}^{\mathrm{2}} \:{such}\:{that}\: \\ $$$$\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1}\:\:\:{and}\:{also}\:{r}={a}\mathrm{cos}\:\theta \\ $$$${and}\:\:\:\:\mathrm{tan}\:\phi=\frac{{x}}{{y}}\:. \\ $$$${Please}\:{help}\:{evaluating}\:{the}\:{integral}.. \\ $$
Commented by ajfour last updated on 26/Aug/17
![x=ytan φ x^2 +y^2 =y^2 (1+tan^2 φ)=r^2 ...(i( ((y^2 tan^2 φ)/a^2 )+(y^2 /b^2 )=1 y^2 (((tan^2 φ)/a^2 )+(1/b^2 ))=1 ....(ii) dividing (i) by (ii): ((1+tan^2 φ)/(((tan^2 φ)/a^2 )+(1/b^2 )))=r^2 ⇒ tan^2 φ=((((r^2 /b^2 )−1))/((1−(r^2 /a^2 )))) 1+tan^2 φ=((r^2 ((1/b^2 )−(1/a^2 )))/((1−(r^2 /a^2 )))) as r=acos θ sec^2 φ=((cos^2 θ(a^2 −b^2 ))/(b^2 sin^2 θ)) ⇒ cos φ=((btan θ)/(√(a^2 −b^2 ))) ⇒ φ=cos^(−1) (((btan θ)/(√(a^2 −b^2 )))) A=8a∫_0 ^( θ_0 ) r𝛗d𝛉 =8a(I) I=∫_0 ^( θ_0 ) acos θcos^(−1) (((btan θ)/(√(a^2 −b^2 ))))dθ =asin θcos^(−1) (((btan θ)/(√(a^2 −b^2 ))))∣_0 ^θ_0 −((−b)/(√(a^2 −b^2 )))∫_0 ^( θ_0 ) (((asin θsec^2 θ)dθ)/(√(1−((b^2 tan^2 θ)/((a^2 −b^2 )))))) and as acos θ_0 =b tan θ_0 =((√(a^2 −b^2 ))/b) , so I=0+ab∫_0 ^( θ_0 ) ((sec θtan θdθ)/(√(a^2 −b^2 −b^2 tan^2 θ))) =a∫_0 ^( θ_0 ) ((d(bsec θ))/(√(a^2 −b^2 sec^2 θ))) =asin^(−1) (((bsec θ)/a))∣_0 ^θ_0 =a[sin^(−1) ((b/(acos θ_0 )))−sin^(−1) ((b/a))] but acos θ_0 =b, so I=a[(π/2)−sin^(−1) (b/a)] and A=8a^2 [(π/2)−sin^(−1) (b/a)] A=4𝛑a^2 −8a^2 sin^(−1) ((b/a)). (still answer dont match) Help !](Q20416.png)
$${x}={y}\mathrm{tan}\:\phi \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={y}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \phi\right)={r}^{\mathrm{2}} \:\:\:\:\:...\left({i}\left(\right.\right. \\ $$$$\frac{{y}^{\mathrm{2}} \mathrm{tan}\:^{\mathrm{2}} \phi}{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$${y}^{\mathrm{2}} \left(\frac{\mathrm{tan}\:^{\mathrm{2}} \phi}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }\right)=\mathrm{1}\:\:\:\:\:\:....\left({ii}\right) \\ $$$${dividing}\:\left({i}\right)\:{by}\:\left({ii}\right): \\ $$$$\frac{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \phi}{\frac{\mathrm{tan}\:^{\mathrm{2}} \phi}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }}={r}^{\mathrm{2}} \:\Rightarrow\:\mathrm{tan}\:^{\mathrm{2}} \phi=\frac{\left(\frac{{r}^{\mathrm{2}} }{{b}^{\mathrm{2}} }−\mathrm{1}\right)}{\left(\mathrm{1}−\frac{{r}^{\mathrm{2}} }{{a}^{\mathrm{2}} }\right)} \\ $$$$\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \phi=\frac{{r}^{\mathrm{2}} \left(\frac{\mathrm{1}}{{b}^{\mathrm{2}} }−\frac{\mathrm{1}}{{a}^{\mathrm{2}} }\right)}{\left(\mathrm{1}−\frac{{r}^{\mathrm{2}} }{{a}^{\mathrm{2}} }\right)} \\ $$$${as}\:{r}={a}\mathrm{cos}\:\theta \\ $$$$\mathrm{sec}\:^{\mathrm{2}} \phi=\frac{\mathrm{cos}\:^{\mathrm{2}} \theta\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)}{{b}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta} \\ $$$$\Rightarrow\:\:\mathrm{cos}\:\phi=\frac{{b}\mathrm{tan}\:\theta}{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}\: \\ $$$$\Rightarrow\:\:\phi=\mathrm{cos}^{−\mathrm{1}} \left(\frac{{b}\mathrm{tan}\:\theta}{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}\right) \\ $$$$\boldsymbol{{A}}=\mathrm{8}{a}\int_{\mathrm{0}} ^{\:\:\theta_{\mathrm{0}} } \boldsymbol{{r}\phi{d}\theta}\:=\mathrm{8}{a}\left({I}\right) \\ $$$$\:\:{I}=\int_{\mathrm{0}} ^{\:\:\theta_{\mathrm{0}} } {a}\mathrm{cos}\:\theta\mathrm{cos}^{−\mathrm{1}} \left(\frac{{b}\mathrm{tan}\:\theta}{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}\right){d}\theta \\ $$$$\:={a}\mathrm{sin}\:\theta\mathrm{cos}^{−\mathrm{1}} \left(\frac{{b}\mathrm{tan}\:\theta}{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}\right)\mid_{\mathrm{0}} ^{\theta_{\mathrm{0}} } \:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:−\frac{−{b}}{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}\int_{\mathrm{0}} ^{\:\theta_{\mathrm{0}} } \frac{\left({a}\mathrm{sin}\:\theta\mathrm{sec}\:^{\mathrm{2}} \theta\right){d}\theta}{\sqrt{\mathrm{1}−\frac{{b}^{\mathrm{2}} \mathrm{tan}\:^{\mathrm{2}} \theta}{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)}}} \\ $$$$\:{and}\:{as}\:{a}\mathrm{cos}\:\theta_{\mathrm{0}} ={b} \\ $$$$\:\:\:\:\mathrm{tan}\:\theta_{\mathrm{0}} =\frac{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}{{b}}\:,\:{so} \\ $$$${I}=\mathrm{0}+{ab}\int_{\mathrm{0}} ^{\:\theta_{\mathrm{0}} } \frac{\mathrm{sec}\:\theta\mathrm{tan}\:\theta{d}\theta}{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} −{b}^{\mathrm{2}} \mathrm{tan}\:^{\mathrm{2}} \theta}} \\ $$$$\:\:={a}\int_{\mathrm{0}} ^{\:\theta_{\mathrm{0}} } \frac{{d}\left({b}\mathrm{sec}\:\theta\right)}{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} \mathrm{sec}\:^{\mathrm{2}} \theta}} \\ $$$$\:\:={a}\mathrm{sin}^{−\mathrm{1}} \left(\frac{{b}\mathrm{sec}\:\theta}{{a}}\right)\mid_{\mathrm{0}} ^{\theta_{\mathrm{0}} } \\ $$$$\:\:={a}\left[\mathrm{sin}^{−\mathrm{1}} \left(\frac{{b}}{{a}\mathrm{cos}\:\theta_{\mathrm{0}} }\right)−\mathrm{sin}^{−\mathrm{1}} \left(\frac{{b}}{{a}}\right)\right] \\ $$$${but}\:{a}\mathrm{cos}\:\theta_{\mathrm{0}} ={b},\:{so} \\ $$$${I}={a}\left[\frac{\pi}{\mathrm{2}}−\mathrm{sin}^{−\mathrm{1}} \frac{{b}}{{a}}\right] \\ $$$${and} \\ $$$$\:\:\:\:{A}=\mathrm{8}{a}^{\mathrm{2}} \left[\frac{\pi}{\mathrm{2}}−\mathrm{sin}^{−\mathrm{1}} \frac{{b}}{{a}}\right] \\ $$$$\:\:\:\:\:\:\boldsymbol{{A}}=\mathrm{4}\boldsymbol{\pi{a}}^{\mathrm{2}} −\mathrm{8}\boldsymbol{{a}}^{\mathrm{2}} \mathrm{sin}^{−\mathrm{1}} \left(\frac{\boldsymbol{{b}}}{\boldsymbol{{a}}}\right). \\ $$$$\left({still}\:{answer}\:{dont}\:{match}\right) \\ $$$$\:\boldsymbol{{Help}}\:! \\ $$
Answered by ajfour last updated on 25/Aug/17
$${Area}=\mathrm{4}\pi{a}^{\mathrm{2}} −\mathrm{8}{a}^{\mathrm{2}} \mathrm{sin}^{−\mathrm{1}} \left(\frac{\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}{{a}}\right)\:. \\ $$
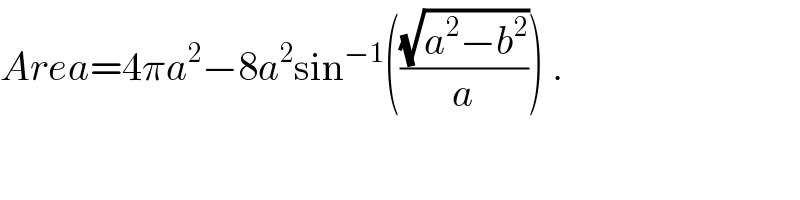
Commented by ajfour last updated on 26/Aug/17
$${This}\:{is}\:{tbe}\:{answer}\:{in}\:{book}. \\ $$$${if}\:{b}\rightarrow{a}\:{then}\:{Area}\:{should}\:{tend} \\ $$$${to}\:{zero}.\:{This}\:{is}\:{fulfilled}\:{by}\:{my} \\ $$$${answer}. \\ $$$${if}\:{b}\rightarrow\mathrm{0}\:{Area}\:{should}\:{tend}\:{to}\:\mathrm{4}\pi{r}^{\mathrm{2}} , \\ $$$${again}\:{this}\:{is}\:{fulfilled}\:{by}\:{my} \\ $$$${answer}\:.. \\ $$