
Question Number 21464 by mondodotto@gmail.com last updated on 24/Sep/17

Answered by myintkhaing last updated on 24/Sep/17
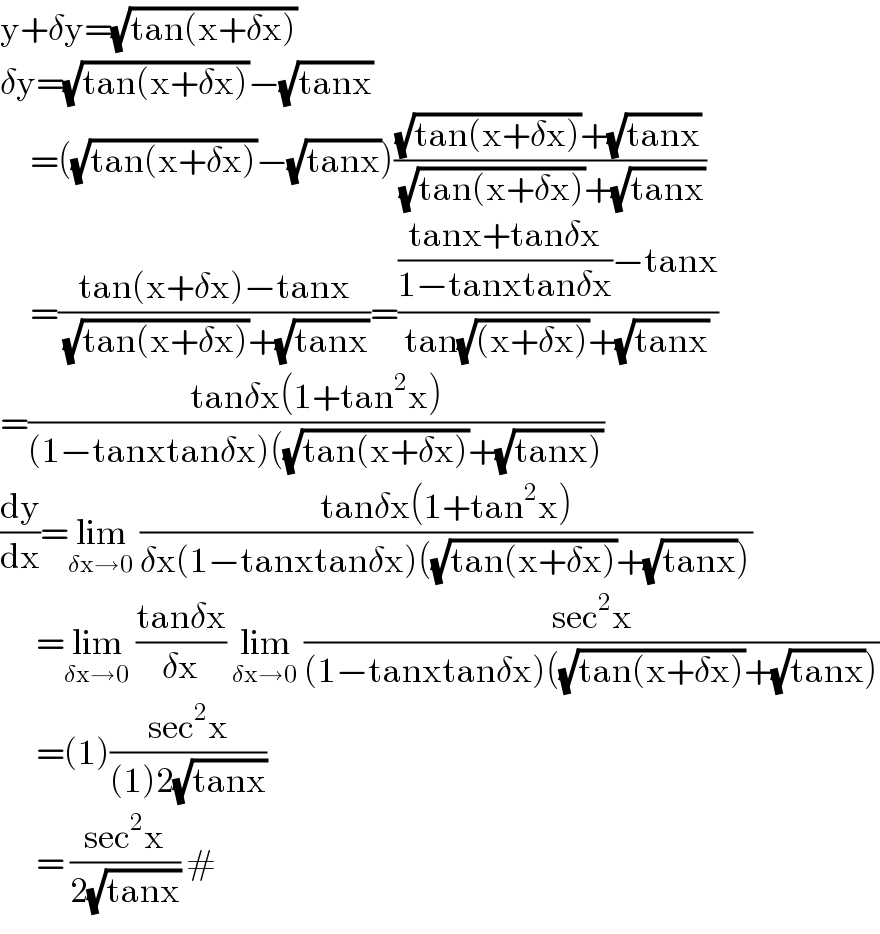
$$\mathrm{y}+\delta\mathrm{y}=\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)} \\ $$$$\delta\mathrm{y}=\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}−\sqrt{\mathrm{tanx}} \\ $$$$\:\:\:\:\:=\left(\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}−\sqrt{\mathrm{tanx}}\right)\frac{\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}+\sqrt{\mathrm{tanx}}}{\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}+\sqrt{\mathrm{tanx}}} \\ $$$$\:\:\:\:\:=\frac{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)−\mathrm{tanx}}{\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}+\sqrt{\mathrm{tanx}}}=\frac{\frac{\mathrm{tanx}+\mathrm{tan}\delta\mathrm{x}}{\mathrm{1}−\mathrm{tanxtan}\delta\mathrm{x}}−\mathrm{tanx}}{\mathrm{tan}\sqrt{\left(\mathrm{x}+\delta\mathrm{x}\right)}+\sqrt{\mathrm{tanx}}} \\ $$$$=\frac{\mathrm{tan}\delta\mathrm{x}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \mathrm{x}\right)}{\left(\mathrm{1}−\mathrm{tanxtan}\delta\mathrm{x}\right)\left(\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}+\sqrt{\left.\mathrm{tanx}\right)}\right.} \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}=\underset{\delta\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{tan}\delta\mathrm{x}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \mathrm{x}\right)}{\delta\mathrm{x}\left(\mathrm{1}−\mathrm{tanxtan}\delta\mathrm{x}\right)\left(\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}+\sqrt{\mathrm{tanx}}\right)} \\ $$$$\:\:\:\:\:\:=\underset{\delta\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{tan}\delta\mathrm{x}}{\delta\mathrm{x}}\:\underset{\delta\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sec}^{\mathrm{2}} \mathrm{x}}{\left(\mathrm{1}−\mathrm{tanxtan}\delta\mathrm{x}\right)\left(\sqrt{\mathrm{tan}\left(\mathrm{x}+\delta\mathrm{x}\right)}+\sqrt{\mathrm{tanx}}\right)} \\ $$$$\:\:\:\:\:\:=\left(\mathrm{1}\right)\frac{\mathrm{sec}^{\mathrm{2}} \mathrm{x}}{\left(\mathrm{1}\right)\mathrm{2}\sqrt{\mathrm{tanx}}}\: \\ $$$$\:\:\:\:\:\:=\:\frac{\mathrm{sec}^{\mathrm{2}} \mathrm{x}}{\mathrm{2}\sqrt{\mathrm{tanx}}}\:# \\ $$
Answered by $@ty@m last updated on 24/Sep/17
![(dy/dx)=lim_(δx→0) (((√(tan (x+δx)))−(√(tanx )))/(δx)) (dy/dx)=lim_(δx→0) (((√(tan (x+δx)))−(√(tanx )))/(tan(x+δx)−tan x))×((tan(x+δx)−tan x)/(δx)) =L_1 ×L_2 where L_1 =lim_(δx→0) (((√(tan (x+δx)))−(√(tanx )))/(tan(x+δx)−tan x)) =(1/(2(√(tan x)))) , using formula lim_(x→a) ((x^n −a^n )/(x−a))=na^(n−1p) and L_2 =lim_(δx→0) ((tan(x+δx)−tan x)/(δx)) =lim_(δx→0) (1/(δx))[((sin (x+δx))/(cos (x+δx)))−((sin x)/(cos x))] =.... =... =sec^2 x {Do it yourself} ∴(dy/dx)=((sec^2 x)/(2(√(tanx ))))](Q21479.png)
$$\frac{{dy}}{{dx}}=\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{tan}\:\left({x}+\delta{x}\right)}−\sqrt{\mathrm{tan}{x}\:}}{\delta{x}} \\ $$$$\frac{{dy}}{{dx}}=\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{tan}\:\left({x}+\delta{x}\right)}−\sqrt{\mathrm{tan}{x}\:}}{\mathrm{tan}\left({x}+\delta{x}\right)−\mathrm{tan}\:{x}}×\frac{\mathrm{tan}\left({x}+\delta{x}\right)−\mathrm{tan}\:{x}}{\delta{x}} \\ $$$$={L}_{\mathrm{1}} ×{L}_{\mathrm{2}} \\ $$$${where}\:{L}_{\mathrm{1}} =\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{tan}\:\left({x}+\delta{x}\right)}−\sqrt{\mathrm{tan}{x}\:}}{\mathrm{tan}\left({x}+\delta{x}\right)−\mathrm{tan}\:{x}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{tan}\:{x}}}\:,\:{using}\:\:{formula}\:\underset{{x}\rightarrow{a}} {\mathrm{lim}}\frac{{x}^{{n}} −{a}^{{n}} }{{x}−{a}}={na}^{{n}−\mathrm{1}{p}} \\ $$$${and}\:{L}_{\mathrm{2}} =\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{tan}\left({x}+\delta{x}\right)−\mathrm{tan}\:{x}}{\delta{x}} \\ $$$$=\underset{\delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{\delta{x}}\left[\frac{\mathrm{sin}\:\left({x}+\delta{x}\right)}{\mathrm{cos}\:\left({x}+\delta{x}\right)}−\frac{\mathrm{sin}\:{x}}{\mathrm{cos}\:{x}}\right] \\ $$$$=.... \\ $$$$=... \\ $$$$=\mathrm{sec}^{\mathrm{2}} {x}\:\left\{{Do}\:{it}\:{yourself}\right\}\: \\ $$$$\therefore\frac{{dy}}{{dx}}=\frac{{sec}^{\mathrm{2}} {x}}{\mathrm{2}\sqrt{\mathrm{tan}{x}\:}} \\ $$
