Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 2272 by Rasheed Soomro last updated on 12/Nov/15

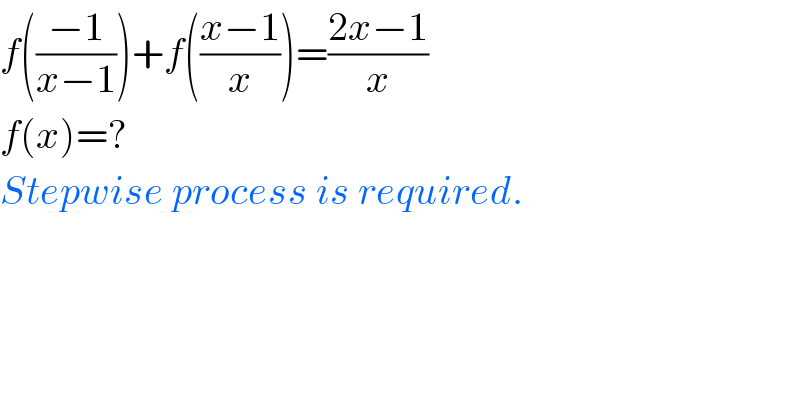
f(((−1)/(x−1)))+f(((x−1)/x))=((2x−1)/x) f(x)=? Stepwise process is required.

$${f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right)+{f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)=\frac{\mathrm{2}{x}−\mathrm{1}}{{x}} \\ $$$${f}\left({x}\right)=? \\ $$$${Stepwise}\:{process}\:{is}\:{required}. \\ $$

Answered by Rasheed Soomro last updated on 16/Nov/15

f(((−1)/(x−1)))+f(((x−1)/x))=((2x−1)/x) In order to get equation involving f(x), we have to replace ((−1)/(x−1)) (or ((x−1)/x) ) by x : Let ((−1)/(x−1))=y⇒x=((y−1)/y) f(((−1)/(x−1)))+f(((x−1)/x))=((2x−1)/x) ⇒f(y)+f(((((y−1)/y)−1)/((y−1)/y)))=((2(((y−1)/y))−1)/((y−1)/y)) ⇒f(y)+f(((−1)/(y−1))) =((y−2)/(y−1)) ⇒f(x)+f(((−1)/(x−1))) =((x−2)/(x−1)) [replacing y by x ] ⇒f(x)=((x−2)/(x−1))−f(((−1)/(x−1)))........................(1) f(((−1)/(x−1)))=((((−1)/(x−1))−2)/(((−1)/(x−1))−1))−f(((−1)/(((−1)/(x−1))−1))) [ replace x by ((−1)/(x−1)) in (1)] =((2x−1)/x)−f(((x−1)/x)) [ replace x by ((−1)/(x−1)) in (1)] f(((x−1)/x))=((((x−1)/x)−2)/(((x−1)/x)−1))−f(((−1)/(((x−1)/x)−1))) [replace x by ((x−1)/x) in (1) ] =x+1−f(x) [replace x by ((x−1)/x) in (1) ] −−−−−−−−−−−−−−−−−−−−− From (1) f(x)=((x−2)/(x−1))−f(((−1)/(x−1))) =((x−2)/(x−1))−f(((−1)/(x−1))) =((x−2)/(x−1))−(((2x−1)/x)−f(((x−1)/x))) =((x−2)/(x−1))−((2x−1)/x)+x+1−f(x) 2f(x)=((x−2)/(x−1))−((2x−1)/x)+x+1 f(x)=(1/2)(((x−2)/(x−1))−((2x−1)/x)+x+1)

$${f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right)+{f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)=\frac{\mathrm{2}{x}−\mathrm{1}}{{x}} \\ $$$${In}\:{order}\:{to}\:{get}\:{equation}\:{involving}\:{f}\left({x}\right), \\ $$$${we}\:{have}\:{to}\:{replace}\:\:\frac{−\mathrm{1}}{{x}−\mathrm{1}}\:\left({or}\:\frac{{x}−\mathrm{1}}{{x}}\:\right)\:{by}\:\:{x}\:\:: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{Let}\:\:\:\:\:\:\frac{−\mathrm{1}}{{x}−\mathrm{1}}={y}\Rightarrow{x}=\frac{{y}−\mathrm{1}}{{y}} \\ $$$$\:\:{f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right)+{f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)=\frac{\mathrm{2}{x}−\mathrm{1}}{{x}}\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow{f}\left({y}\right)+{f}\left(\frac{\frac{{y}−\mathrm{1}}{{y}}−\mathrm{1}}{\frac{{y}−\mathrm{1}}{{y}}}\right)=\frac{\mathrm{2}\left(\frac{{y}−\mathrm{1}}{{y}}\right)−\mathrm{1}}{\frac{{y}−\mathrm{1}}{{y}}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow{f}\left({y}\right)+{f}\left(\frac{−\mathrm{1}}{{y}−\mathrm{1}}\right)\:=\frac{{y}−\mathrm{2}}{{y}−\mathrm{1}}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow{f}\left({x}\right)+{f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right)\:=\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}\:\:\:\:\left[{replacing}\:{y}\:{by}\:{x}\:\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow{f}\left({x}\right)=\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}−{f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right)........................\left(\mathrm{1}\right) \\ $$$$\:\:\:{f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right)=\frac{\frac{−\mathrm{1}}{{x}−\mathrm{1}}−\mathrm{2}}{\frac{−\mathrm{1}}{{x}−\mathrm{1}}−\mathrm{1}}−{f}\left(\frac{−\mathrm{1}}{\frac{−\mathrm{1}}{{x}−\mathrm{1}}−\mathrm{1}}\right)\:\:\:\:\left[\:{replace}\:{x}\:{by}\:\frac{−\mathrm{1}}{{x}−\mathrm{1}}\:{in}\:\left(\mathrm{1}\right)\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{2}{x}−\mathrm{1}}{{x}}−{f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)\:\:\:\:\left[\:{replace}\:{x}\:{by}\:\frac{−\mathrm{1}}{{x}−\mathrm{1}}\:{in}\:\left(\mathrm{1}\right)\right] \\ $$$$\:\:{f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)=\frac{\frac{{x}−\mathrm{1}}{{x}}−\mathrm{2}}{\frac{{x}−\mathrm{1}}{{x}}−\mathrm{1}}−{f}\left(\frac{−\mathrm{1}}{\frac{{x}−\mathrm{1}}{{x}}−\mathrm{1}}\right)\:\:\:\left[{replace}\:{x}\:{by}\:\frac{{x}−\mathrm{1}}{{x}}\:{in}\:\left(\mathrm{1}\right)\:\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:={x}+\mathrm{1}−{f}\left({x}\right)\:\:\:\left[{replace}\:{x}\:{by}\:\frac{{x}−\mathrm{1}}{{x}}\:{in}\:\left(\mathrm{1}\right)\:\right] \\ $$$$−−−−−−−−−−−−−−−−−−−−− \\ $$$${From}\:\left(\mathrm{1}\right)\:\:{f}\left({x}\right)=\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}−{f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}−{f}\left(\frac{−\mathrm{1}}{{x}−\mathrm{1}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}−\left(\frac{\mathrm{2}{x}−\mathrm{1}}{{x}}−{f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}−\frac{\mathrm{2}{x}−\mathrm{1}}{{x}}+{x}+\mathrm{1}−{f}\left({x}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}{f}\left({x}\right)=\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}−\frac{\mathrm{2}{x}−\mathrm{1}}{{x}}+{x}+\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{x}−\mathrm{2}}{{x}−\mathrm{1}}−\frac{\mathrm{2}{x}−\mathrm{1}}{{x}}+{x}+\mathrm{1}\right) \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com