
Question Number 2273 by RasheedAhmad last updated on 12/Nov/15
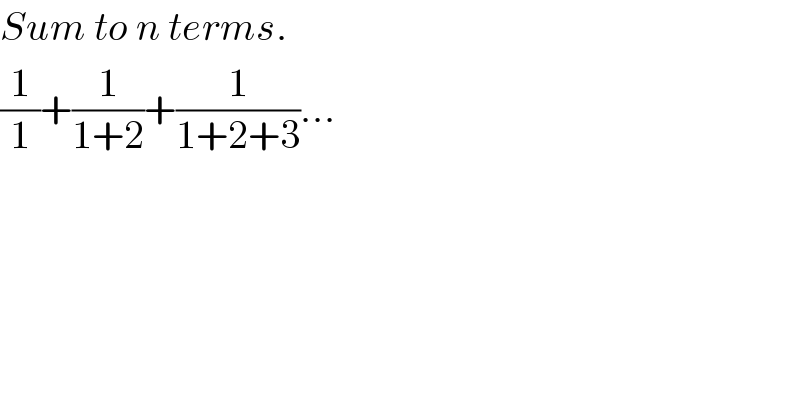
$${Sum}\:{to}\:{n}\:{terms}. \\ $$$$\frac{\mathrm{1}}{\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}+\mathrm{3}}... \\ $$
Answered by Yozzi last updated on 12/Nov/15
![For an A.P with first term a and common difference d, its sum to n terms is given by S(n)=(n/2)(2a+(n−1)d). For A.Ps in demominator, the first term is a=1 and common difference is d=1. Thus, the sum to r terms of each A.P in the denominators, is given by S(r)=(r/2)(2×1+(r−1)×1) =(r/2)(2+r−1) S(r)=(r/2)(r+1). The general term U_r for the given series is U_r =(1/(S(r)))=(2/(r(r+1))). Observe that (1/r)−(1/(r+1))=((r+1−r)/(r(r+1)))=(1/(r(r+1))). ∴U_r =2((1/r)−(1/(r+1))) Now let f(r)=1/r (r∈N) ⇒U_r =2(f(r)−f(r+1)) ∴ Σ_(r=1) ^n U_r =2[f(1)−f(2)]+2[f(2)−f(3)] +2[f(3)−f(4)]+2[f(4)−f(5)]+2[f(5) −f(6)]+...+2[f(n−3)−f(n−2)]+ 2[f(n−2)−f(n−1)]+2[f(n−1)−f(n)] +2[f(n)−f(n+1)] ⇒Σ_(r=1) ^n U_r =2[f(1)−f(n+1)]=2((1/1)−(1/(n+1))) Σ_(r=1) ^n (2/(r(r+1)))=2(1−(1/(n+1))) Corollary: lim_(n→∞) Σ_(r=1) ^n (2/(r(r+1)))=lim_(n→∞) [2(1−(1/(n+1)))] =2−(2/∞) =2](Q2274.png)
$${For}\:{an}\:{A}.{P}\:{with}\:{first}\:{term}\:{a}\:{and} \\ $$$${common}\:{difference}\:{d},\:{its}\:{sum}\:{to}\:{n} \\ $$$${terms}\:{is}\:{given}\:{by} \\ $$$${S}\left({n}\right)=\frac{{n}}{\mathrm{2}}\left(\mathrm{2}{a}+\left({n}−\mathrm{1}\right){d}\right). \\ $$$${For}\:{A}.{Ps}\:\:{in}\:{demominator},\:{the} \\ $$$${first}\:{term}\:{is}\:{a}=\mathrm{1}\:{and}\:{common}\: \\ $$$${difference}\:{is}\:{d}=\mathrm{1}.\:{Thus},\:{the}\:{sum}\:{to}\:{r} \\ $$$${terms}\:{of}\:{each}\:{A}.{P}\:{in}\:{the}\:{denominators}, \\ $$$${is}\:{given}\:{by} \\ $$$${S}\left({r}\right)=\frac{{r}}{\mathrm{2}}\left(\mathrm{2}×\mathrm{1}+\left({r}−\mathrm{1}\right)×\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:=\frac{{r}}{\mathrm{2}}\left(\mathrm{2}+{r}−\mathrm{1}\right) \\ $$$${S}\left({r}\right)=\frac{{r}}{\mathrm{2}}\left({r}+\mathrm{1}\right). \\ $$$$ \\ $$$${The}\:{general}\:{term}\:{U}_{{r}} \:{for}\:{the}\:{given} \\ $$$${series}\:{is}\: \\ $$$${U}_{{r}} =\frac{\mathrm{1}}{{S}\left({r}\right)}=\frac{\mathrm{2}}{{r}\left({r}+\mathrm{1}\right)}. \\ $$$${Observe}\:{that} \\ $$$$\frac{\mathrm{1}}{{r}}−\frac{\mathrm{1}}{{r}+\mathrm{1}}=\frac{{r}+\mathrm{1}−{r}}{{r}\left({r}+\mathrm{1}\right)}=\frac{\mathrm{1}}{{r}\left({r}+\mathrm{1}\right)}.\: \\ $$$$\therefore{U}_{{r}} =\mathrm{2}\left(\frac{\mathrm{1}}{{r}}−\frac{\mathrm{1}}{{r}+\mathrm{1}}\right) \\ $$$${Now}\:{let}\:{f}\left({r}\right)=\mathrm{1}/{r}\:\:\:\left({r}\in\mathbb{N}\right) \\ $$$$\Rightarrow{U}_{{r}} =\mathrm{2}\left({f}\left({r}\right)−{f}\left({r}+\mathrm{1}\right)\right) \\ $$$$\therefore\:\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}{U}_{{r}} =\mathrm{2}\left[{f}\left(\mathrm{1}\right)−{f}\left(\mathrm{2}\right)\right]+\mathrm{2}\left[{f}\left(\mathrm{2}\right)−{f}\left(\mathrm{3}\right)\right] \\ $$$$+\mathrm{2}\left[{f}\left(\mathrm{3}\right)−{f}\left(\mathrm{4}\right)\right]+\mathrm{2}\left[{f}\left(\mathrm{4}\right)−{f}\left(\mathrm{5}\right)\right]+\mathrm{2}\left[{f}\left(\mathrm{5}\right)\right. \\ $$$$\left.−{f}\left(\mathrm{6}\right)\right]+...+\mathrm{2}\left[{f}\left({n}−\mathrm{3}\right)−{f}\left({n}−\mathrm{2}\right)\right]+ \\ $$$$\mathrm{2}\left[{f}\left({n}−\mathrm{2}\right)−{f}\left({n}−\mathrm{1}\right)\right]+\mathrm{2}\left[{f}\left({n}−\mathrm{1}\right)−{f}\left({n}\right)\right] \\ $$$$+\mathrm{2}\left[{f}\left({n}\right)−{f}\left({n}+\mathrm{1}\right)\right] \\ $$$$\Rightarrow\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}{U}_{{r}} =\mathrm{2}\left[{f}\left(\mathrm{1}\right)−{f}\left({n}+\mathrm{1}\right)\right]=\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{1}}−\frac{\mathrm{1}}{{n}+\mathrm{1}}\right) \\ $$$$\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{2}}{{r}\left({r}+\mathrm{1}\right)}=\mathrm{2}\left(\mathrm{1}−\frac{\mathrm{1}}{{n}+\mathrm{1}}\right)\: \\ $$$${Corollary}: \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{2}}{{r}\left({r}+\mathrm{1}\right)}=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left[\mathrm{2}\left(\mathrm{1}−\frac{\mathrm{1}}{{n}+\mathrm{1}}\right)\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{2}−\frac{\mathrm{2}}{\infty} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{2} \\ $$
Commented by Rasheed Soomro last updated on 13/Nov/15

$$\:\overset{\mathcal{VERY}} {\:\mathcal{NICE}} \\ $$
Answered by Rasheed Soomro last updated on 13/Nov/15
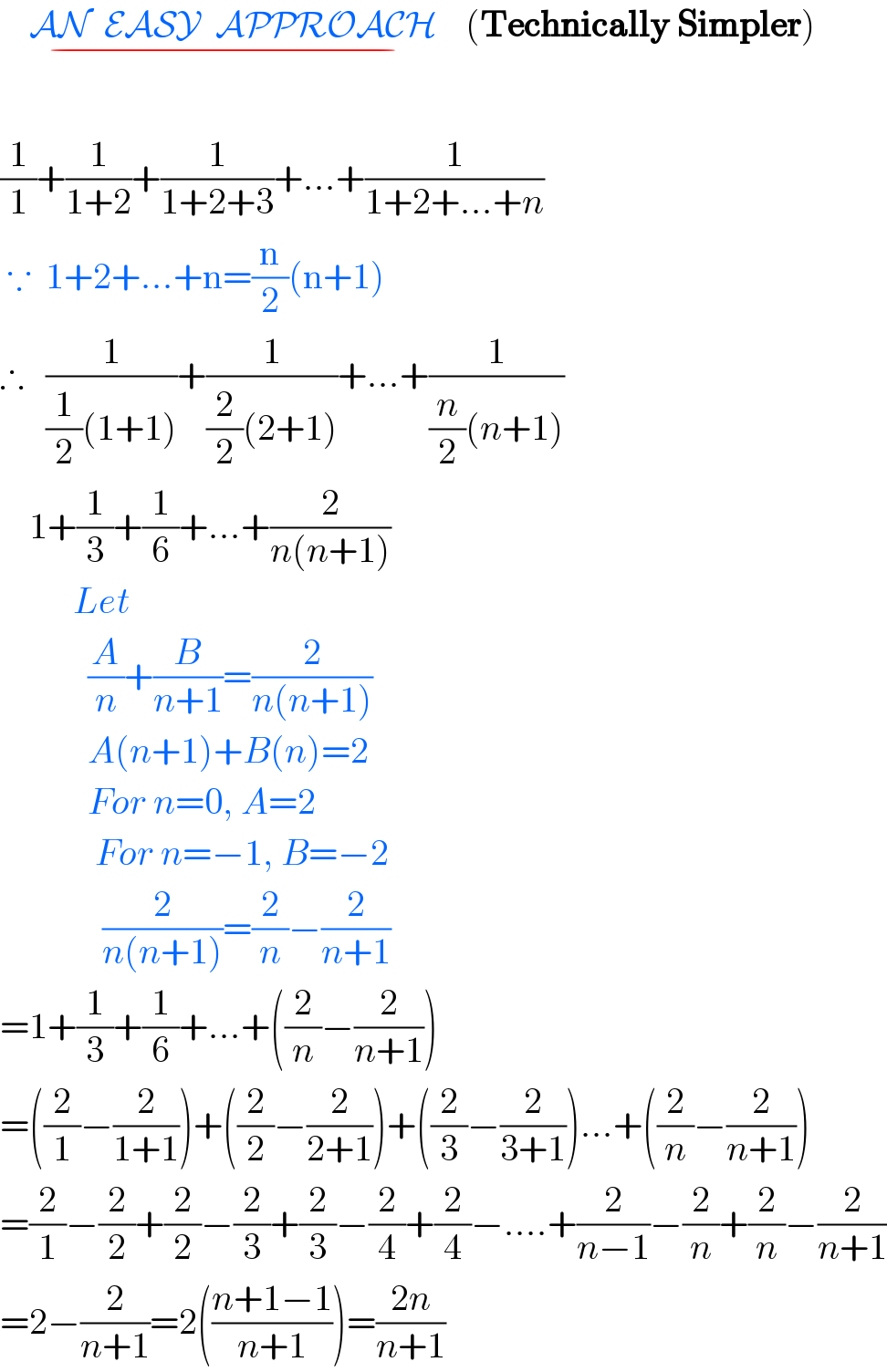
$$\underset{−} {\:\:\:\:\mathcal{AN}\:\:\mathcal{EASY}\:\:\mathcal{APPROACH}\:\:\:\:}\left(\boldsymbol{\mathrm{Technically}}\:\boldsymbol{\mathrm{Simpler}}\right) \\ $$$$ \\ $$$$\frac{\mathrm{1}}{\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}+\mathrm{3}}+...+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}+...+{n}} \\ $$$$\:\because\:\:\mathrm{1}+\mathrm{2}+...+\mathrm{n}=\frac{\mathrm{n}}{\mathrm{2}}\left(\mathrm{n}+\mathrm{1}\right) \\ $$$$\therefore\:\:\:\frac{\mathrm{1}}{\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\mathrm{1}\right)}+\frac{\mathrm{1}}{\frac{\mathrm{2}}{\mathrm{2}}\left(\mathrm{2}+\mathrm{1}\right)}+...+\frac{\mathrm{1}}{\frac{{n}}{\mathrm{2}}\left({n}+\mathrm{1}\right)} \\ $$$$\:\:\:\:\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{6}}+...+\frac{\mathrm{2}}{{n}\left({n}+\mathrm{1}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:{Let}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\frac{{A}}{{n}}+\frac{{B}}{{n}+\mathrm{1}}=\frac{\mathrm{2}}{{n}\left({n}+\mathrm{1}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{A}\left({n}+\mathrm{1}\right)+{B}\left({n}\right)=\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{For}\:{n}=\mathrm{0},\:{A}=\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:{For}\:{n}=−\mathrm{1},\:{B}=−\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{2}}{{n}\left({n}+\mathrm{1}\right)}=\frac{\mathrm{2}}{{n}}−\frac{\mathrm{2}}{{n}+\mathrm{1}} \\ $$$$=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{6}}+...+\left(\frac{\mathrm{2}}{{n}}−\frac{\mathrm{2}}{{n}+\mathrm{1}}\right) \\ $$$$=\left(\frac{\mathrm{2}}{\mathrm{1}}−\frac{\mathrm{2}}{\mathrm{1}+\mathrm{1}}\right)+\left(\frac{\mathrm{2}}{\mathrm{2}}−\frac{\mathrm{2}}{\mathrm{2}+\mathrm{1}}\right)+\left(\frac{\mathrm{2}}{\mathrm{3}}−\frac{\mathrm{2}}{\mathrm{3}+\mathrm{1}}\right)...+\left(\frac{\mathrm{2}}{{n}}−\frac{\mathrm{2}}{{n}+\mathrm{1}}\right) \\ $$$$=\frac{\mathrm{2}}{\mathrm{1}}−\frac{\mathrm{2}}{\mathrm{2}}+\frac{\mathrm{2}}{\mathrm{2}}−\frac{\mathrm{2}}{\mathrm{3}}+\frac{\mathrm{2}}{\mathrm{3}}−\frac{\mathrm{2}}{\mathrm{4}}+\frac{\mathrm{2}}{\mathrm{4}}−....+\frac{\mathrm{2}}{{n}−\mathrm{1}}−\frac{\mathrm{2}}{{n}}+\frac{\mathrm{2}}{{n}}−\frac{\mathrm{2}}{{n}+\mathrm{1}} \\ $$$$=\mathrm{2}−\frac{\mathrm{2}}{{n}+\mathrm{1}}=\mathrm{2}\left(\frac{{n}+\mathrm{1}−\mathrm{1}}{{n}+\mathrm{1}}\right)=\frac{\mathrm{2}{n}}{{n}+\mathrm{1}} \\ $$
