Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 24227 by tawa tawa last updated on 14/Nov/17

Commented by tawa tawa last updated on 14/Nov/17

please help.

$$\mathrm{please}\:\mathrm{help}. \\ $$

Answered by $@ty@m last updated on 14/Nov/17

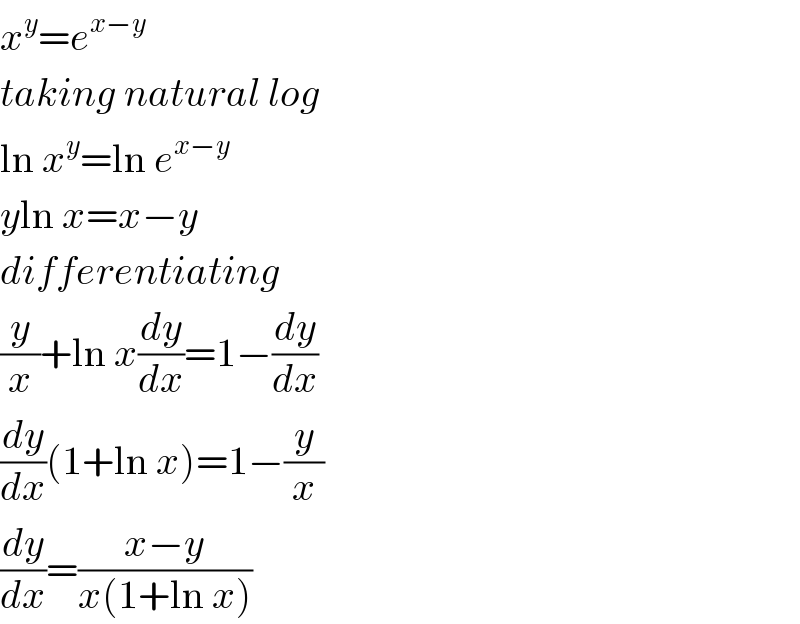
x^y =e^(x−y) taking natural log ln x^y =ln e^(x−y) yln x=x−y differentiating (y/x)+ln x(dy/dx)=1−(dy/dx) (dy/dx)(1+ln x)=1−(y/x) (dy/dx)=((x−y)/(x(1+ln x)))

$${x}^{{y}} ={e}^{{x}−{y}} \\ $$$${taking}\:{natural}\:{log} \\ $$$$\mathrm{ln}\:{x}^{{y}} =\mathrm{ln}\:{e}^{{x}−{y}} \\ $$$${y}\mathrm{ln}\:{x}={x}−{y} \\ $$$${differentiating} \\ $$$$\frac{{y}}{{x}}+\mathrm{ln}\:{x}\frac{{dy}}{{dx}}=\mathrm{1}−\frac{{dy}}{{dx}} \\ $$$$\frac{{dy}}{{dx}}\left(\mathrm{1}+\mathrm{ln}\:{x}\right)=\mathrm{1}−\frac{{y}}{{x}} \\ $$$$\frac{{dy}}{{dx}}=\frac{{x}−{y}}{{x}\left(\mathrm{1}+\mathrm{ln}\:{x}\right)} \\ $$

Commented by tawa tawa last updated on 16/Nov/17

God bless you sir

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Commented by tawa tawa last updated on 14/Nov/17

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Commented by abwayh last updated on 16/Nov/17

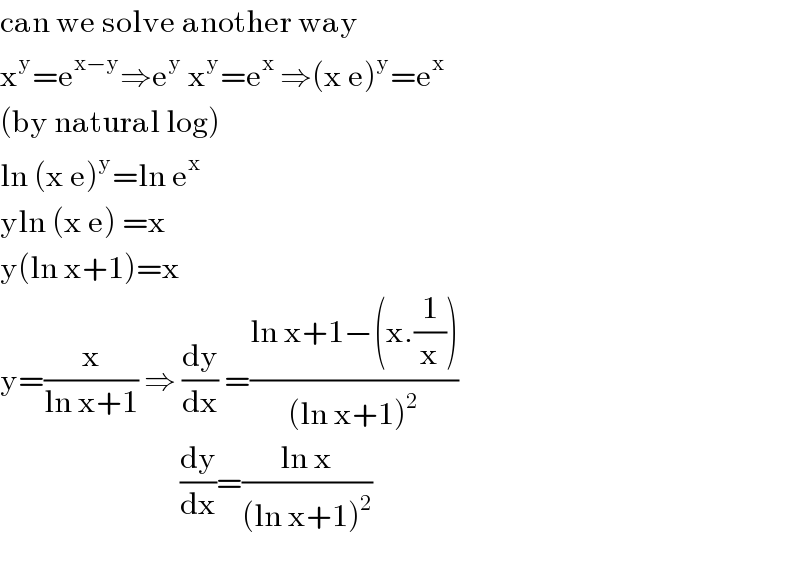
can we solve another way x^y =e^(x−y) ⇒e^y x^y =e^x ⇒(x e)^y =e^x (by natural log) ln (x e)^y =ln e^x yln (x e) =x y(ln x+1)=x y=(x/(ln x+1)) ⇒ (dy/dx) =((ln x+1−(x.(1/x)))/((ln x+1)^2 )) (dy/dx)=((ln x)/((ln x+1)^2 ))

$$\mathrm{can}\:\mathrm{we}\:\mathrm{solve}\:\mathrm{another}\:\mathrm{way} \\ $$$$\mathrm{x}^{\mathrm{y}} =\mathrm{e}^{\mathrm{x}−\mathrm{y}} \Rightarrow\mathrm{e}^{\mathrm{y}} \:\mathrm{x}^{\mathrm{y}} =\mathrm{e}^{\mathrm{x}} \:\Rightarrow\left(\mathrm{x}\:\mathrm{e}\right)^{\mathrm{y}} =\mathrm{e}^{\mathrm{x}} \\ $$$$\left(\mathrm{by}\:\mathrm{natural}\:\mathrm{log}\right) \\ $$$$\mathrm{ln}\:\left(\mathrm{x}\:\mathrm{e}\right)^{\mathrm{y}} =\mathrm{ln}\:\mathrm{e}^{\mathrm{x}} \: \\ $$$$\mathrm{yln}\:\left(\mathrm{x}\:\mathrm{e}\right)\:=\mathrm{x} \\ $$$$\mathrm{y}\left(\mathrm{ln}\:\mathrm{x}+\mathrm{1}\right)=\mathrm{x} \\ $$$$\mathrm{y}=\frac{\mathrm{x}}{\mathrm{ln}\:\mathrm{x}+\mathrm{1}}\:\Rightarrow\:\frac{\mathrm{dy}}{\mathrm{dx}}\:=\frac{\mathrm{ln}\:\mathrm{x}+\mathrm{1}−\left(\mathrm{x}.\frac{\mathrm{1}}{\mathrm{x}}\right)}{\left(\mathrm{ln}\:\mathrm{x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{ln}\:\mathrm{x}}{\left(\mathrm{ln}\:\mathrm{x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$ \\ $$

Commented by $@ty@m last updated on 16/Nov/17

Its same

$${Its}\:{same} \\ $$

Answered by $@ty@m last updated on 14/Nov/17

∫x^n ln xdx Integrating by parts =ln x∫x^n dx−∫(x^(n+1) /(x.(n+1)))dx =(x^(n+1) /(n+1))ln x−∫(x^n /(n+1)) =(x^(n+1) /(n+1))ln x−(x^(n+1) /((n+1)^2 ))+C

$$\int{x}^{{n}} \mathrm{ln}\:{xdx} \\ $$$${Integrating}\:{by}\:{parts} \\ $$$$=\mathrm{ln}\:{x}\int{x}^{{n}} {dx}−\int\frac{{x}^{{n}+\mathrm{1}} }{{x}.\left({n}+\mathrm{1}\right)}{dx} \\ $$$$=\frac{{x}^{{n}+\mathrm{1}} }{{n}+\mathrm{1}}\mathrm{ln}\:{x}−\int\frac{{x}^{{n}} }{{n}+\mathrm{1}} \\ $$$$=\frac{{x}^{{n}+\mathrm{1}} }{{n}+\mathrm{1}}\mathrm{ln}\:{x}−\frac{{x}^{{n}+\mathrm{1}} }{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }+{C} \\ $$

Commented by tawa tawa last updated on 14/Nov/17

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Answered by $@ty@m last updated on 14/Nov/17

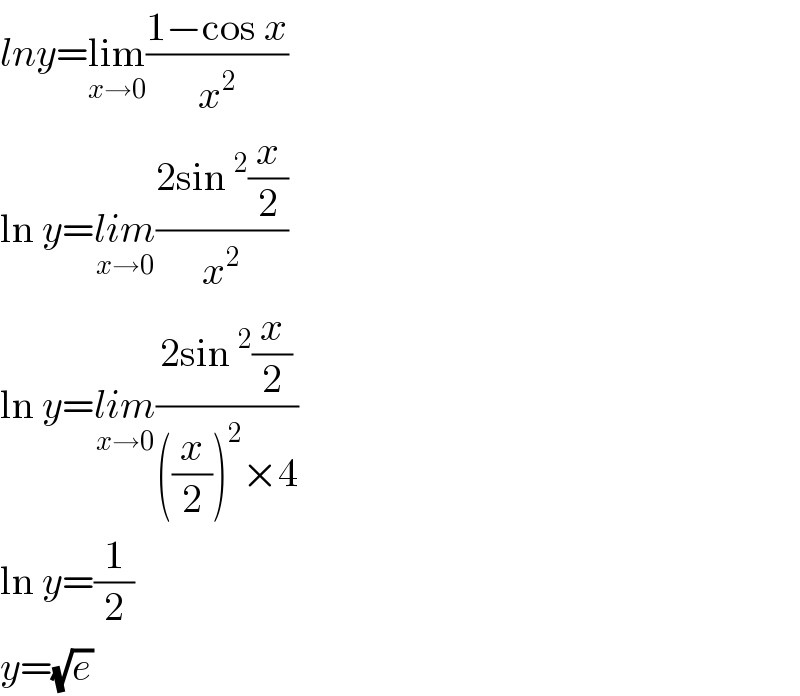
lny=lim_(x→0) ((1−cos x)/x^2 ) ln y=lim_(x→0) ((2sin^2 (x/2))/x^2 ) ln y=lim_(x→0) ((2sin^2 (x/2))/(((x/2))^2 ×4)) ln y=(1/2) y=(√e)

$${lny}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\mathrm{cos}\:{x}}{{x}^{\mathrm{2}} } \\ $$$$\mathrm{ln}\:{y}=\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{\mathrm{2sin}\:^{\mathrm{2}} \frac{{x}}{\mathrm{2}}}{{x}^{\mathrm{2}} } \\ $$$$\mathrm{ln}\:{y}=\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{\mathrm{2sin}\:^{\mathrm{2}} \frac{{x}}{\mathrm{2}}}{\left(\frac{{x}}{\mathrm{2}}\right)^{\mathrm{2}} ×\mathrm{4}} \\ $$$$\mathrm{ln}\:{y}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${y}=\sqrt{{e}} \\ $$

Commented by tawa tawa last updated on 16/Nov/17

God bless you sir

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Commented by tawa tawa last updated on 14/Nov/17

God bless you sir

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Commented by tawa tawa last updated on 14/Nov/17

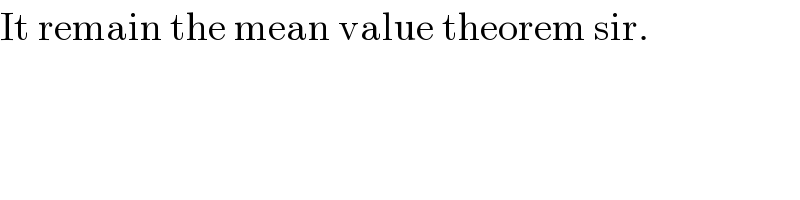
It remain the mean value theorem sir.

$$\mathrm{It}\:\mathrm{remain}\:\mathrm{the}\:\mathrm{mean}\:\mathrm{value}\:\mathrm{theorem}\:\mathrm{sir}. \\ $$

Commented by abwayh last updated on 16/Nov/17

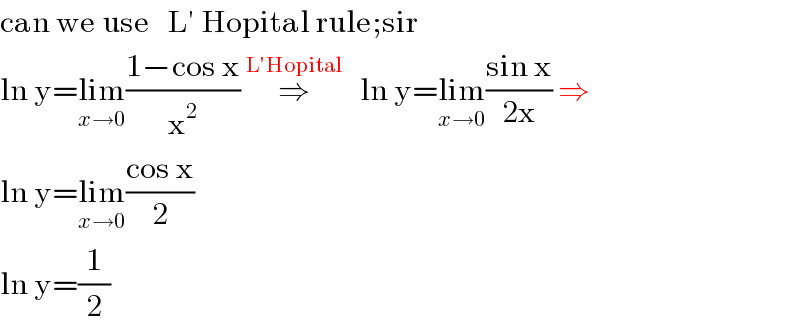
can we use L′ Hopital rule;sir ln y=lim_(x→0) ((1−cos x)/x^2 ) ⇒^(L′Hopital) ln y=lim_(x→0) ((sin x)/(2x)) ⇒ ln y=lim_(x→0) ((cos x)/2) ln y=(1/2)

$$\mathrm{can}\:\mathrm{we}\:\mathrm{use}\:\:\:\mathrm{L}'\:\mathrm{Hopital}\:\mathrm{rule};\mathrm{sir} \\ $$$$\mathrm{ln}\:\mathrm{y}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\mathrm{cos}\:\mathrm{x}}{\mathrm{x}^{\mathrm{2}} }\:\overset{\mathrm{L}'\mathrm{Hopital}} {\Rightarrow}\:\:\:\mathrm{ln}\:\mathrm{y}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{2x}}\:\Rightarrow \\ $$$$\mathrm{ln}\:\mathrm{y}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{cos}\:\mathrm{x}}{\mathrm{2}} \\ $$$$\mathrm{ln}\:\mathrm{y}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Commented by $@ty@m last updated on 16/Nov/17

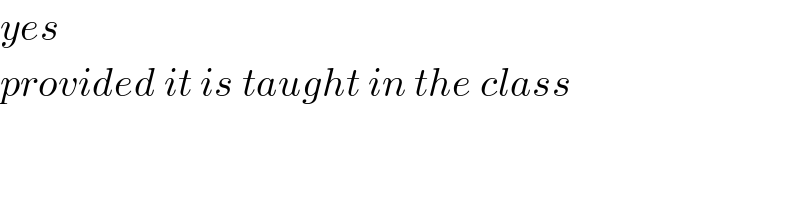
yes provided it is taught in the class

$${yes} \\ $$$${provided}\:{it}\:{is}\:{taught}\:{in}\:{the}\:{class} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com