
Question Number 24447 by Tinkutara last updated on 18/Nov/17

$${Figure}\:{shows}\:{two}\:{discs}\:{of}\:{same}\:{mass} \\ $$$${m}.\:{They}\:{are}\:{rigidly}\:{attached}\:{to}\:{a}\:{spring} \\ $$$${of}\:{stiffness}\:{k}.\:{The}\:{system}\:{is}\:{in} \\ $$$${equilibrium}.\:{From}\:{this}\:{equilibrium} \\ $$$${position},\:{the}\:{upper}\:{disc}\:{is}\:{pressed} \\ $$$${down}\:{slowly}\:{by}\:{a}\:{distance}\:{x}\:{and} \\ $$$${released}.\:{Find}\:{the}\:{minimum}\:{value}\:{of} \\ $$$${x},\:{if}\:{the}\:{lower}\:{disc}\:{is}\:{just}\:{lifted}\:{off} \\ $$$${the}\:{ground}. \\ $$
Commented by Tinkutara last updated on 18/Nov/17

Commented by ajfour last updated on 18/Nov/17
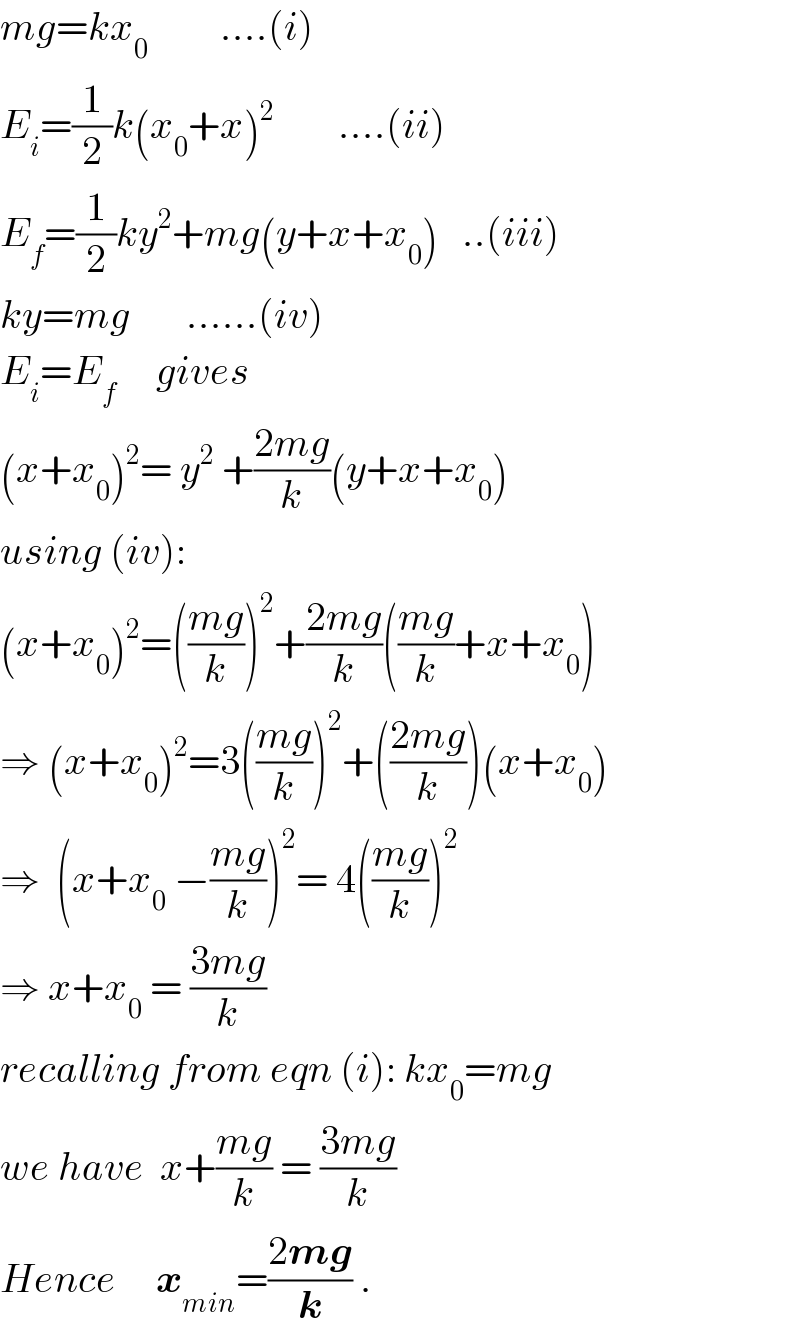
$${mg}={kx}_{\mathrm{0}} \:\:\:\:\:\:\:\:\:....\left({i}\right) \\ $$$${E}_{{i}} =\frac{\mathrm{1}}{\mathrm{2}}{k}\left({x}_{\mathrm{0}} +{x}\right)^{\mathrm{2}} \:\:\:\:\:\:\:\:....\left({ii}\right) \\ $$$${E}_{{f}} =\frac{\mathrm{1}}{\mathrm{2}}{ky}^{\mathrm{2}} +{mg}\left({y}+{x}+{x}_{\mathrm{0}} \right)\:\:\:..\left({iii}\right) \\ $$$${ky}={mg}\:\:\:\:\:\:\:......\left({iv}\right) \\ $$$${E}_{{i}} ={E}_{{f}} \:\:\:\:\:{gives} \\ $$$$\left({x}+{x}_{\mathrm{0}} \right)^{\mathrm{2}} =\:{y}^{\mathrm{2}} \:+\frac{\mathrm{2}{mg}}{{k}}\left({y}+{x}+{x}_{\mathrm{0}} \right) \\ $$$${using}\:\left({iv}\right): \\ $$$$\left({x}+{x}_{\mathrm{0}} \right)^{\mathrm{2}} =\left(\frac{{mg}}{{k}}\right)^{\mathrm{2}} +\frac{\mathrm{2}{mg}}{{k}}\left(\frac{{mg}}{{k}}+{x}+{x}_{\mathrm{0}} \right) \\ $$$$\Rightarrow\:\left({x}+{x}_{\mathrm{0}} \right)^{\mathrm{2}} =\mathrm{3}\left(\frac{{mg}}{{k}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{2}{mg}}{{k}}\right)\left({x}+{x}_{\mathrm{0}} \right) \\ $$$$\Rightarrow\:\:\left({x}+{x}_{\mathrm{0}} \:−\frac{{mg}}{{k}}\right)^{\mathrm{2}} =\:\mathrm{4}\left(\frac{{mg}}{{k}}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\:{x}+{x}_{\mathrm{0}} \:=\:\frac{\mathrm{3}{mg}}{{k}} \\ $$$${recalling}\:{from}\:{eqn}\:\left({i}\right):\:{kx}_{\mathrm{0}} ={mg} \\ $$$${we}\:{have}\:\:{x}+\frac{{mg}}{{k}}\:=\:\frac{\mathrm{3}{mg}}{{k}} \\ $$$${Hence}\:\:\:\:\:\boldsymbol{{x}}_{{min}} =\frac{\mathrm{2}\boldsymbol{{mg}}}{\boldsymbol{{k}}}\:. \\ $$
Commented by Tinkutara last updated on 18/Nov/17

$${Why}\:{not}\:{E}_{{i}} =\frac{\mathrm{1}}{\mathrm{2}}{kx}_{\mathrm{0}} ^{\mathrm{2}} \:{and}\:{how}\:{it}\:{become} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\left({x}+{x}_{\mathrm{0}} \right)^{\mathrm{2}} ?\:{We}\:{pressed}\:{it}\:{down}\:{so}\:{it} \\ $$$${should}\:{be}\:{subtracted}. \\ $$
Commented by ajfour last updated on 18/Nov/17

$${the}\:{spring}\:{was}\:{compressed}\:{by} \\ $$$${x}_{\mathrm{0}} \:{due}\:{to}\:{weight}\:{of}\:{upper}\:{disc} \\ $$$${when}\:{it}\:{was}\:{in}\:{equilibrium}. \\ $$$${Pressing}\:{it}\:{down}\:{further}\:{by}\:{x} \\ $$$${increases}\:{spring}\:{potential}\:{energy} \\ $$$${to}\:\:\:\:\:\frac{\mathrm{1}}{\mathrm{2}}{k}\left({x}+{x}_{\mathrm{0}} \right)^{\mathrm{2}} \:\:.\:{This}\:{instant} \\ $$$${is}\:{taken}\:{as}\:{the}\:{initial}\:{position} \\ $$$${in}\:{my}\:{solution}.\:{Gravitational} \\ $$$${potential}\:{energy}\:{is}\:{taken}\:{zero}\:{here}. \\ $$$${From}\:{here}\:{upper}\:{disc}\:{rises}\: \\ $$$${through}\:{a}\:{height}\:\:\:=\:{y}−\left(−{x}_{\mathrm{0}} −{x}\right) \\ $$$$\:\:\:=\:{y}+{x}_{\mathrm{0}} +{x} \\ $$$${y}\:{is}\:{the}\:{mximum}\:{extension}\:{in} \\ $$$${spring}\:{that}\:{comes}\:{about}\:{in}\:{the} \\ $$$${spring}.\:{For}\:{minimum}\:{x},\: \\ $$$${upper}\:{disc}\:{is}\:{at}\:{its}\:{maximum} \\ $$$${height}\:{then}.\:{Spring}\:{pulls}\:{both} \\ $$$${discs}\:{towards}\:{each}\:{other},\:{and} \\ $$$${this}\:{pull}\:{is}\:{just}\:{sufficient}\:{to} \\ $$$${make}\:{the}\:{normal}\:{reaction}\:\left({of}\right. \\ $$$$\left.{ground}\right)\:{to}\:{the}\:{lower}\:{disc}\:{become} \\ $$$${zero},\:{that}\:{is}\:{to}\:{say},\:{the}\:{lower}\: \\ $$$${disc}\:{is}\:{lifted}\:{off}\:{the}\:{ground}\:{for} \\ $$$${any}\:{value}\:{of}\:{x}\:{superior}\:{to}\:\:\frac{\mathrm{2}{mg}}{{k}}\:. \\ $$
Commented by Tinkutara last updated on 18/Nov/17
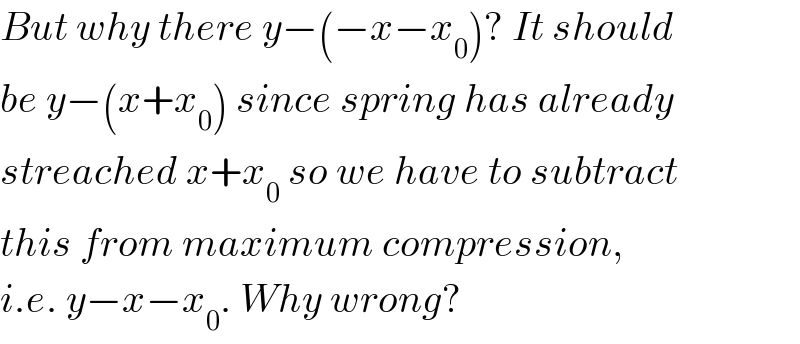
$${But}\:{why}\:{there}\:{y}−\left(−{x}−{x}_{\mathrm{0}} \right)?\:{It}\:{should} \\ $$$${be}\:{y}−\left({x}+{x}_{\mathrm{0}} \right)\:{since}\:{spring}\:{has}\:{already} \\ $$$${streached}\:{x}+{x}_{\mathrm{0}} \:{so}\:{we}\:{have}\:{to}\:{subtract} \\ $$$${this}\:{from}\:{maximum}\:{compression}, \\ $$$${i}.{e}.\:{y}−{x}−{x}_{\mathrm{0}} .\:{Why}\:{wrong}? \\ $$
Commented by ajfour last updated on 18/Nov/17

Commented by ajfour last updated on 18/Nov/17
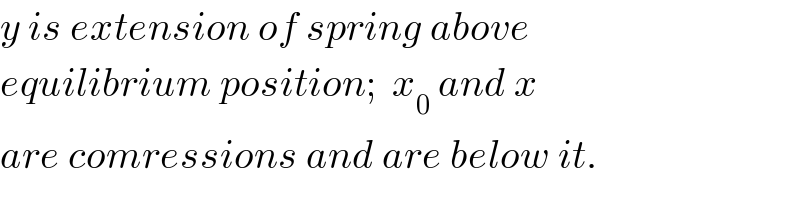
$${y}\:{is}\:{extension}\:{of}\:{spring}\:{above} \\ $$$${equilibrium}\:{position};\:\:{x}_{\mathrm{0}} \:{and}\:{x} \\ $$$${are}\:{comressions}\:{and}\:{are}\:{below}\:{it}. \\ $$
Commented by Tinkutara last updated on 18/Nov/17
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$
