
Question Number 24576 by math solver last updated on 21/Nov/17

Commented by math solver last updated on 21/Nov/17

$$\mathrm{sum}\:\mathrm{of}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{is}\:? \\ $$
Answered by mrW1 last updated on 21/Nov/17

$$\frac{{x}+\mathrm{1}}{\mathrm{2}}=\mathrm{log}_{\mathrm{2}} \:\left(\mathrm{2}^{{x}} +\mathrm{3}\right)−\frac{\mathrm{log}_{\mathrm{2}} \left(\mathrm{1980}−\mathrm{2}^{−{x}} \right)\:}{\mathrm{log}_{\mathrm{2}} \:\mathrm{4}} \\ $$$$\frac{{x}+\mathrm{1}}{\mathrm{2}}=\mathrm{log}_{\mathrm{2}} \:\left(\mathrm{2}^{{x}} +\mathrm{3}\right)−\frac{\mathrm{log}_{\mathrm{2}} \left(\mathrm{1980}−\mathrm{2}^{−{x}} \right)\:}{\mathrm{2}} \\ $$$${x}+\mathrm{1}=\mathrm{2log}_{\mathrm{2}} \:\left(\mathrm{2}^{{x}} +\mathrm{3}\right)−\mathrm{log}_{\mathrm{2}} \:\left(\mathrm{1980}−\mathrm{2}^{−{x}} \right) \\ $$$${x}+\mathrm{1}=\mathrm{log}_{\mathrm{2}} \:\frac{\left(\mathrm{2}^{{x}} +\mathrm{3}\right)^{\mathrm{2}} }{\mathrm{1980}−\mathrm{2}^{−{x}} } \\ $$$$\mathrm{2}^{{x}+\mathrm{1}} =\frac{\left(\mathrm{2}^{{x}} +\mathrm{3}\right)^{\mathrm{2}} }{\mathrm{1980}−\mathrm{2}^{−{x}} } \\ $$$$\mathrm{2}×\mathrm{2}^{{x}} =\frac{\left(\mathrm{2}^{{x}} +\mathrm{3}\right)^{\mathrm{2}} }{\mathrm{1980}−\mathrm{2}^{−{x}} } \\ $$$$\mathrm{2}×\mathrm{1980}×\mathrm{2}^{{x}} −\mathrm{2}=\left(\mathrm{2}^{{x}} +\mathrm{3}\right)^{\mathrm{2}} =\left(\mathrm{2}^{{x}} \right)^{\mathrm{2}} +\mathrm{6}\left(\mathrm{2}^{{x}} \right)+\mathrm{9} \\ $$$$\left(\mathrm{2}^{{x}} \right)^{\mathrm{2}} −\mathrm{3954}\left(\mathrm{2}^{{x}} \right)+\mathrm{11}=\mathrm{0}\:\:\:\:\:\:...\left({i}\right) \\ $$$$\Rightarrow\mathrm{2}^{{x}} =\frac{\mathrm{3954}\pm\sqrt{\mathrm{3954}^{\mathrm{2}} −\mathrm{4}×\mathrm{11}}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{2}^{{x}} =\mathrm{1977}\pm\sqrt{\mathrm{3908518}} \\ $$$$\Rightarrow{x}=\mathrm{log}_{\mathrm{2}} \:\left(\mathrm{1977}\pm\sqrt{\mathrm{3908518}}\right) \\ $$$$\left(\approx−\mathrm{8}.\mathrm{49}\:{or}\:\mathrm{11}.\mathrm{95}\right) \\ $$$$\Rightarrow\Sigma{x}=\mathrm{log}_{\mathrm{2}} \:\left(\mathrm{1977}+\sqrt{\mathrm{3908518}}\right)\left(\mathrm{1977}−\sqrt{\mathrm{3908518}}\right) \\ $$$$\Rightarrow\Sigma{x}=\mathrm{log}_{\mathrm{2}} \:\left(\mathrm{1977}^{\mathrm{2}} −\mathrm{3908518}\right)=\mathrm{log}_{\mathrm{2}} \:\mathrm{11}\approx\mathrm{3}.\mathrm{46} \\ $$$$ \\ $$$${or}\:{directly}\:{from}\:\left({i}\right) \\ $$$$\mathrm{2}^{{x}_{\mathrm{1}} } ×\mathrm{2}^{{x}_{\mathrm{2}} } =\mathrm{11} \\ $$$$\Rightarrow\mathrm{2}^{{x}_{\mathrm{1}} +{x}_{\mathrm{2}} } =\mathrm{11} \\ $$$$\Rightarrow{x}_{\mathrm{1}} +{x}_{\mathrm{2}} =\mathrm{log}_{\mathrm{2}} \:\mathrm{11} \\ $$
Commented by mrW1 last updated on 22/Nov/17
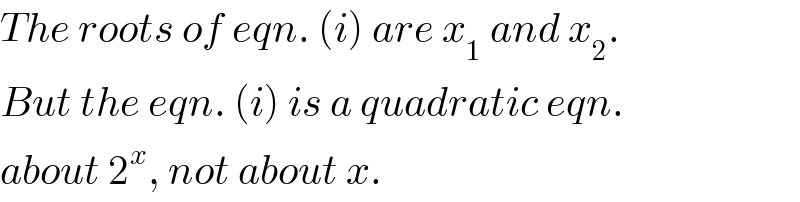
$${The}\:{roots}\:{of}\:{eqn}.\:\left({i}\right)\:{are}\:{x}_{\mathrm{1}} \:{and}\:{x}_{\mathrm{2}} . \\ $$$${But}\:{the}\:{eqn}.\:\left({i}\right)\:{is}\:{a}\:{quadratic}\:{eqn}.\: \\ $$$${about}\:\mathrm{2}^{{x}} ,\:{not}\:{about}\:{x}. \\ $$
Commented by math solver last updated on 22/Nov/17

$$\mathrm{i}\:\mathrm{want}\:\mathrm{to}\:\mathrm{ask}\:: \\ $$$$\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{eq}.\:\left({i}\right)\:\mathrm{are}:\:{x}_{\mathrm{1}} \:{and}\:{x}_{\mathrm{2}} . \\ $$$${or}\:\mathrm{2}^{{x}_{\mathrm{1}} } \:{and}\:\mathrm{2}^{{x}_{\mathrm{2}} } \:\:? \\ $$
Commented by mrW1 last updated on 22/Nov/17

$${The}\:{original}\:{question}\:{asks}\:{how}\:{much} \\ $$$${is}\:{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +...,\:{not}\:{how}\:{much}\:{is} \\ $$$$\mathrm{2}^{{x}_{\mathrm{1}} } +\mathrm{2}^{{x}_{\mathrm{2}} } +... \\ $$
Commented by math solver last updated on 22/Nov/17

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}! \\ $$
