Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 2489 by Filup last updated on 21/Nov/15

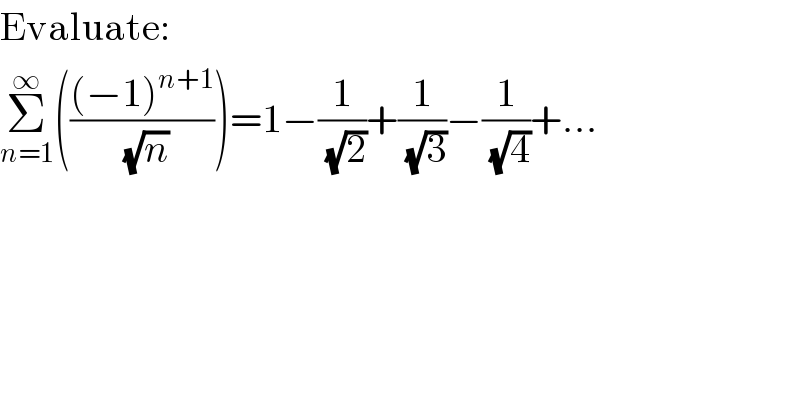
Evaluate: Σ_(n=1) ^∞ ((((−1)^(n+1) )/(√n)))=1−(1/(√2))+(1/(√3))−(1/(√4))+...

$$\mathrm{Evaluate}: \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\sqrt{{n}}}\right)=\mathrm{1}−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}−\frac{\mathrm{1}}{\sqrt{\mathrm{4}}}+... \\ $$

Commented by Yozzi last updated on 21/Nov/15

Σ_(r=1) ^∞ (((−1)^(r+1) )/(√n))=Σ_(r=1) ^∞ (1/(√(2r−1)))−Σ_(r=1) ^∞ (1/(√(2r))) Let f(x)=(2x−1)^(−1/2) . f is decreasing and positive for x≥1 so the integral test could show whether Σ_(r=1) ^∞ (1/(√(2r−1))) is convergent or not. Let I=∫_1 ^∞ f(x)dx=lim_(m→∞) ∫_1 ^m (2x−1)^(−1/2) dx I=lim_(m→∞) ((((2x−1)^(1/2) )/(2×1/2)))∣_1 ^m I=lim_(m→∞) [(√(2m−1))−(√(2×1−1))] I=lim_(m→∞) [(√(2m−1))−1]=(√(2×∞−1))−1=∞ Thus,since I does not exist,Σ_(r=1) ^∞ (1/(√(2r−1))) is divergent. {Hence, Σ_(r=1) ^∞ (((−1)^(n+1) )/(√n)) is divergent.} (×)

$$\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{r}+\mathrm{1}} }{\sqrt{{n}}}=\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\sqrt{\mathrm{2}{r}−\mathrm{1}}}−\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\sqrt{\mathrm{2}{r}}} \\ $$$${Let}\:{f}\left({x}\right)=\left(\mathrm{2}{x}−\mathrm{1}\right)^{−\mathrm{1}/\mathrm{2}} .\:{f}\:{is}\:{decreasing} \\ $$$${and}\:{positive}\:{for}\:{x}\geqslant\mathrm{1}\:{so}\:{the}\:{integral} \\ $$$${test}\:{could}\:{show}\:{whether}\:\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\sqrt{\mathrm{2}{r}−\mathrm{1}}}\:{is} \\ $$$${convergent}\:{or}\:{not}. \\ $$$${Let}\:{I}=\int_{\mathrm{1}} ^{\infty} {f}\left({x}\right){dx}=\underset{{m}\rightarrow\infty} {\mathrm{lim}}\int_{\mathrm{1}} ^{{m}} \left(\mathrm{2}{x}−\mathrm{1}\right)^{−\mathrm{1}/\mathrm{2}} {dx} \\ $$$${I}=\underset{{m}\rightarrow\infty} {\mathrm{lim}}\left(\frac{\left(\mathrm{2}{x}−\mathrm{1}\right)^{\mathrm{1}/\mathrm{2}} }{\mathrm{2}×\mathrm{1}/\mathrm{2}}\right)\mid_{\mathrm{1}} ^{{m}} \\ $$$${I}=\underset{{m}\rightarrow\infty} {\mathrm{lim}}\left[\sqrt{\mathrm{2}{m}−\mathrm{1}}−\sqrt{\mathrm{2}×\mathrm{1}−\mathrm{1}}\right] \\ $$$${I}=\underset{{m}\rightarrow\infty} {\mathrm{lim}}\left[\sqrt{\mathrm{2}{m}−\mathrm{1}}−\mathrm{1}\right]=\sqrt{\mathrm{2}×\infty−\mathrm{1}}−\mathrm{1}=\infty \\ $$$${Thus},{since}\:{I}\:{does}\:{not}\:{exist},\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\sqrt{\mathrm{2}{r}−\mathrm{1}}} \\ $$$${is}\:{divergent}.\: \\ $$$$\left\{{Hence},\:\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\sqrt{{n}}}\:{is}\:{divergent}.\right\}\:\left(×\right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Answered by Yozzi last updated on 21/Nov/15

Σ_(r=1) ^∞ ((((−1)^(n+1) )/(√n))) does not converge. (Incorrect−disregard this contribution).

$$\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\sqrt{{n}}}\right)\:{does}\:{not}\:{converge}. \\ $$$$\left({Incorrect}−{disregard}\:{this}\:{contribution}\right). \\ $$

Commented by prakash jain last updated on 21/Nov/15

The sequence may be conditinally convergent. I think the tests in comment only prove that it is not absolutely convergent.

$$\mathrm{The}\:\mathrm{sequence}\:\mathrm{may}\:\mathrm{be}\:\mathrm{conditinally}\:\mathrm{convergent}. \\ $$$$\mathrm{I}\:\mathrm{think}\:\mathrm{the}\:\mathrm{tests}\:\mathrm{in}\:\mathrm{comment}\:\mathrm{only}\:\mathrm{prove} \\ $$$$\mathrm{that}\:\mathrm{it}\:\mathrm{is}\:\mathrm{not}\:\mathrm{absolutely}\:\mathrm{convergent}. \\ $$

Commented by 123456 last updated on 21/Nov/15

0<a_(n+1) <a_n (by alternating test it converge) its coditionly converge since Σ1/(√n) diverge by p series test

$$\mathrm{0}<{a}_{{n}+\mathrm{1}} <{a}_{{n}} \:\left(\mathrm{by}\:\mathrm{alternating}\:\mathrm{test}\:\mathrm{it}\:\mathrm{converge}\right) \\ $$$$\mathrm{its}\:\mathrm{coditionly}\:\mathrm{converge}\:\mathrm{since} \\ $$$$\Sigma\mathrm{1}/\sqrt{{n}} \\ $$$$\mathrm{diverge}\:\mathrm{by}\:{p}\:\mathrm{series}\:\mathrm{test} \\ $$

Answered by prakash jain last updated on 21/Nov/15

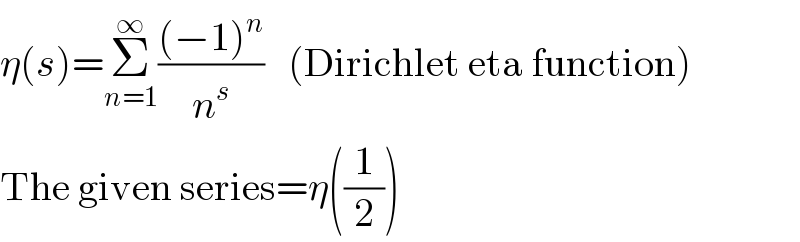
η(s)=Σ_(n=1) ^∞ (((−1)^n )/n^s ) (Dirichlet eta function) The given series=η((1/2))

$$\eta\left({s}\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}^{{s}} }\:\:\:\left(\mathrm{Dirichlet}\:\mathrm{eta}\:\mathrm{function}\right) \\ $$$$\mathrm{The}\:\mathrm{given}\:\mathrm{series}=\eta\left(\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com