Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 2499 by Syaka last updated on 21/Nov/15

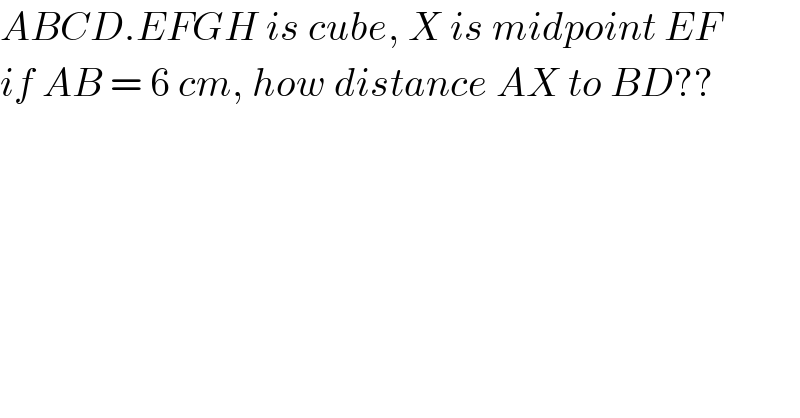
ABCD.EFGH is cube, X is midpoint EF if AB = 6 cm, how distance AX to BD??

$${ABCD}.{EFGH}\:{is}\:{cube},\:{X}\:{is}\:{midpoint}\:{EF} \\ $$$${if}\:{AB}\:=\:\mathrm{6}\:{cm},\:{how}\:{distance}\:{AX}\:{to}\:{BD}?? \\ $$

Commented by prakash jain last updated on 21/Nov/15

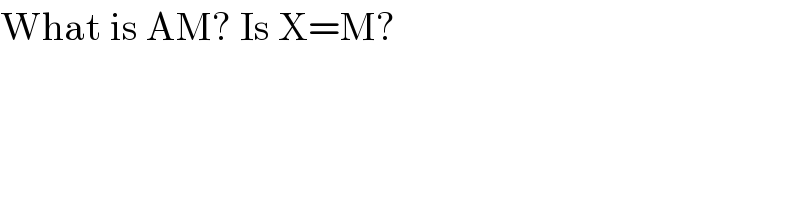
What is AM? Is X=M?

$$\mathrm{What}\:\mathrm{is}\:\mathrm{AM}?\:\mathrm{Is}\:\mathrm{X}=\mathrm{M}? \\ $$

Commented by Syaka last updated on 21/Nov/15

sorry Sir, I mistake when write this question and now I′ve corrected

$${sorry}\:{Sir},\:{I}\:{mistake}\:{when}\:{write}\:{this}\:{question} \\ $$$${and}\:{now}\:{I}'{ve}\:{corrected} \\ $$

Answered by prakash jain last updated on 21/Nov/15

Let A(0,0,0) A=(0,0,0) B=(6,0,0) C=(6,6,0) D=(0,6,0) E=(0,0,6) F=(6,0,6) G=(6,6,6) H=(0,6,6) X=(3,0,6) direction vector d_1 ^→ =BD^(→) =(−6,6,0) BD: ((x−6)/(−6))=(y/6)=t, z=0 x=6−6t, y=6t, z=0 (some point Q on line BD) direction vector d_2 ^→ =AX^(→) =(3,0,6) AX: (x/3)=(z/6)=s, y=0 x=3s, z=6s, y=0 (some point R on line AX) QR^(→) =(3s−6+6t,−6t,6s) To calculate the distance we need to solve for s and t such QR^(→) ⊥BD^(→) and QR^(→) ⊥AX^(→) . QR^(→) •BD^(→) =0 and QR^(→) •AX^(→) =0 Once s and t are found we can find coordinates of Q and R and calculate the distance. QR^(→) •BD^(→) =0 ⇒−18s+36−36t−36t=0 s+4t=2 ....(1) QR^(→) •AX^(→) =0 ⇒9s−18+18t+36s=0 5s+2t=2 ....(2) From(1) and (2) t=(4/9),s=(2/9) x=6−((24)/9)=((10)/3), y=(8/3), z=0 ( point Q on line BD) x=(2/3), z=(4/3), y=0 (point R on line AX) QR^(→) is ⊥ to both BD and AX and hence its length gives the distance. QR=(√(((8/3))^2 +((8/3))^2 +((4/3))^2 ))=(1/3)(√(128+16))=4

$$\mathrm{Let}\:\mathrm{A}\left(\mathrm{0},\mathrm{0},\mathrm{0}\right) \\ $$$$\mathrm{A}=\left(\mathrm{0},\mathrm{0},\mathrm{0}\right)\:\mathrm{B}=\left(\mathrm{6},\mathrm{0},\mathrm{0}\right)\:\mathrm{C}=\left(\mathrm{6},\mathrm{6},\mathrm{0}\right)\:\mathrm{D}=\left(\mathrm{0},\mathrm{6},\mathrm{0}\right) \\ $$$$\mathrm{E}=\left(\mathrm{0},\mathrm{0},\mathrm{6}\right)\:\mathrm{F}=\left(\mathrm{6},\mathrm{0},\mathrm{6}\right)\:\mathrm{G}=\left(\mathrm{6},\mathrm{6},\mathrm{6}\right)\:\mathrm{H}=\left(\mathrm{0},\mathrm{6},\mathrm{6}\right) \\ $$$$\mathrm{X}=\left(\mathrm{3},\mathrm{0},\mathrm{6}\right) \\ $$$$\mathrm{direction}\:\mathrm{vector}\:\overset{\rightarrow} {{d}}_{\mathrm{1}} =\overset{\rightarrow} {\mathrm{BD}}=\left(−\mathrm{6},\mathrm{6},\mathrm{0}\right) \\ $$$$\mathrm{BD}:\:\:\:\frac{{x}−\mathrm{6}}{−\mathrm{6}}=\frac{{y}}{\mathrm{6}}={t},\:{z}=\mathrm{0} \\ $$$${x}=\mathrm{6}−\mathrm{6}{t},\:{y}=\mathrm{6}{t},\:{z}=\mathrm{0}\:\left(\mathrm{some}\:{point}\:{Q}\:\mathrm{on}\:\mathrm{line}\:\mathrm{BD}\right) \\ $$$$\mathrm{direction}\:\mathrm{vector}\:\overset{\rightarrow} {{d}}_{\mathrm{2}} =\overset{\rightarrow} {\mathrm{AX}}=\left(\mathrm{3},\mathrm{0},\mathrm{6}\right) \\ $$$$\mathrm{AX}:\:\frac{{x}}{\mathrm{3}}=\frac{{z}}{\mathrm{6}}={s},\:{y}=\mathrm{0}\: \\ $$$${x}=\mathrm{3}{s},\:{z}=\mathrm{6}{s},\:{y}=\mathrm{0}\:\:\:\:\left(\mathrm{some}\:\mathrm{point}\:\mathrm{R}\:\mathrm{on}\:\mathrm{line}\:\mathrm{AX}\right) \\ $$$$\overset{\rightarrow} {\mathrm{QR}}=\left(\mathrm{3}{s}−\mathrm{6}+\mathrm{6}{t},−\mathrm{6}{t},\mathrm{6}{s}\right) \\ $$$$\mathrm{To}\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{distance}\:\mathrm{we}\:\mathrm{need}\:\mathrm{to}\:\mathrm{solve} \\ $$$$\mathrm{for}\:{s}\:\mathrm{and}\:{t}\:\mathrm{such}\:\overset{\rightarrow} {\mathrm{QR}}\bot\overset{\rightarrow} {\mathrm{BD}}\:\mathrm{and}\:\overset{\rightarrow} {\mathrm{QR}}\bot\overset{\rightarrow} {\mathrm{AX}}. \\ $$$$\overset{\rightarrow} {\mathrm{QR}}\bullet\overset{\rightarrow} {\mathrm{BD}}\:=\mathrm{0}\:\mathrm{and}\:\overset{\rightarrow} {\mathrm{QR}}\bullet\overset{\rightarrow} {\mathrm{AX}}=\mathrm{0} \\ $$$$\mathrm{Once}\:{s}\:\mathrm{and}\:{t}\:\mathrm{are}\:\mathrm{found}\:\mathrm{we}\:\mathrm{can}\:\mathrm{find}\:\mathrm{coordinates} \\ $$$$\mathrm{of}\:\mathrm{Q}\:\mathrm{and}\:\mathrm{R}\:\mathrm{and}\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{distance}. \\ $$$$\overset{\rightarrow} {\mathrm{QR}}\bullet\overset{\rightarrow} {\mathrm{BD}}\:=\mathrm{0}\:\Rightarrow−\mathrm{18}{s}+\mathrm{36}−\mathrm{36}{t}−\mathrm{36}{t}=\mathrm{0} \\ $$$${s}+\mathrm{4}{t}=\mathrm{2}\:\:\:\:\:\:\:....\left(\mathrm{1}\right) \\ $$$$ \\ $$$$\overset{\rightarrow} {\mathrm{QR}}\bullet\overset{\rightarrow} {\mathrm{AX}}\:=\mathrm{0}\:\Rightarrow\mathrm{9}{s}−\mathrm{18}+\mathrm{18}{t}+\mathrm{36}{s}=\mathrm{0} \\ $$$$\mathrm{5}{s}+\mathrm{2}{t}=\mathrm{2}\:\:\:\:\:....\left(\mathrm{2}\right) \\ $$$$\mathrm{From}\left(\mathrm{1}\right)\:\mathrm{and}\:\left(\mathrm{2}\right)\:{t}=\frac{\mathrm{4}}{\mathrm{9}},{s}=\frac{\mathrm{2}}{\mathrm{9}} \\ $$$${x}=\mathrm{6}−\frac{\mathrm{24}}{\mathrm{9}}=\frac{\mathrm{10}}{\mathrm{3}},\:{y}=\frac{\mathrm{8}}{\mathrm{3}},\:{z}=\mathrm{0}\:\left(\:{point}\:{Q}\:\mathrm{on}\:\mathrm{line}\:\mathrm{BD}\right) \\ $$$${x}=\frac{\mathrm{2}}{\mathrm{3}},\:{z}=\frac{\mathrm{4}}{\mathrm{3}},\:{y}=\mathrm{0}\:\:\:\:\left(\mathrm{point}\:\mathrm{R}\:\mathrm{on}\:\mathrm{line}\:\mathrm{AX}\right) \\ $$$$\overset{\rightarrow} {\mathrm{QR}}\:\mathrm{is}\:\bot\:\mathrm{to}\:\mathrm{both}\:\mathrm{BD}\:\mathrm{and}\:\mathrm{AX}\:\mathrm{and}\:\mathrm{hence}\:\mathrm{its} \\ $$$$\mathrm{length}\:\mathrm{gives}\:\mathrm{the}\:\mathrm{distance}. \\ $$$$\mathrm{QR}=\sqrt{\left(\frac{\mathrm{8}}{\mathrm{3}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{8}}{\mathrm{3}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{4}}{\mathrm{3}}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{3}}\sqrt{\mathrm{128}+\mathrm{16}}=\mathrm{4} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com