Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 25589 by behi.8.3.4.17@gmail.com last updated on 11/Dec/17

$$\mathit{p}\mathit{o}\mathit{s}\mathit{s}\mathit{i}\mathit{b}\mathit{l}\mathit{e}\mathit{o}\mathit{r}\mathit{n}\mathit{o}\mathit{t}?$$ $${\mathit{a}}^{\mathit{a}}+{\mathit{b}}^{\mathit{b}}>{\mathit{a}}^{\mathit{b}}+{\mathit{b}}^{\mathit{a}}$$ $$\mathit{w}\mathit{i}\mathit{t}\mathit{h}\mathit{b}\mathit{e}\mathit{l}\mathit{o}\mathit{w}\mathit{c}\mathit{o}\mathit{n}\mathit{d}\mathit{i}\mathit{t}\mathit{i}\mathit{o}\mathit{n}\mathit{s}:$$ $$\mathit{c}\mathit{a}\mathit{s}\mathit{e}1)\mathit{a}>\mathit{b}>1$$ $$\mathit{c}\mathit{a}\mathit{s}\mathit{e}2)0<\mathit{b}<\mathit{a}<1$$

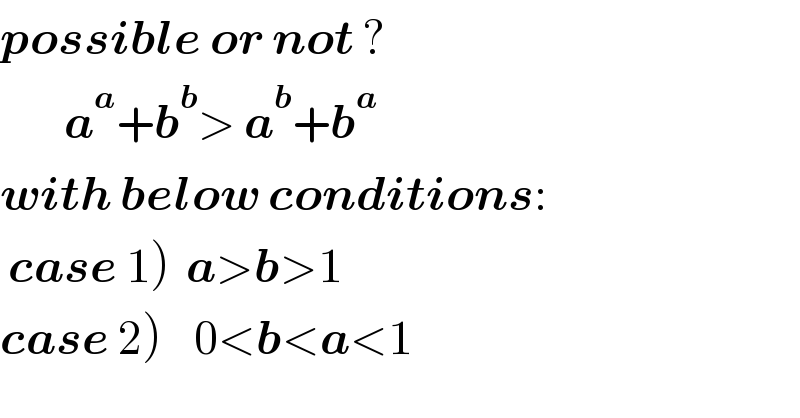
Commented byprakash jain last updated on 12/Dec/17

$$a=3,b=2$$ $$27+4=31>9+8=17$$

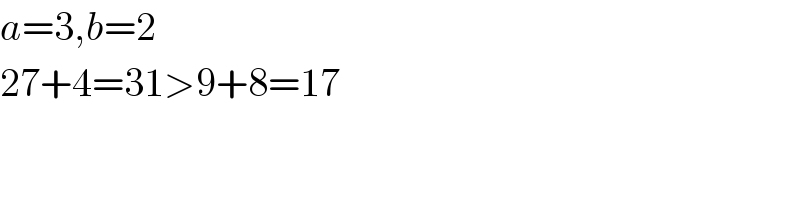
Commented bymoxhix last updated on 12/Dec/17

$$a^b+b^a<a^a+b^b,b<a$$ $$\iff a^b-b^b-(a^a-b^a)<0$$ $$\iff \frac{(a^b-b^b)-(a^a-b^a)}{b-a}>0(\because b-a<0)$$ $$$$ $$letf\left(x\right)=a^x-b^x(x>0)$$ $$f{}^{\prime }\left(x\right)=a^{x}lna-b^{x}lnb$$ $$\left(i\right)1<b<a$$ $$0<lnb<lna,0<b^x<a^x$$ $$\therefore f{}^{\prime }\left(x\right)>0(\mathrm{\forall }x>0)$$ $$\left(ii\right)0<b<a<1$$ $$lnb<lna<0,0<b^x<a^x$$ $$\therefore f{}^{\prime }\left(x\right)<0(\mathrm{\forall }x>0)$$ $$$$ $$MeanValueTheorem$$ $$\mathrm{\exists }c\in (b,a)s.t.f{}^{\prime }\left(c\right)=\frac{f\left(b\right)-f\left(a\right)}{b-a}$$ $$\therefore$$ $$1<b<a\Rightarrow f{}^{\prime }\left(c\right)=\frac{a^b-b^b-(a^a-b^a)}{b-a}>0$$ $$0<b<a\Rightarrow f{}^{\prime }\left(c\right)<0$$

Commented bybehi.8.3.4.17@gmail.com last updated on 12/Dec/17

$$\mathrm{thank}\mathrm{you}\mathrm{very}\mathrm{much}\mathrm{dear}.\mathrm{your}$$ $$\mathrm{solution}\mathrm{is}\mathrm{so}\mathrm{beautiful}.$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com