
Question Number 26148 by ajfour last updated on 21/Dec/17

Commented by ajfour last updated on 21/Dec/17
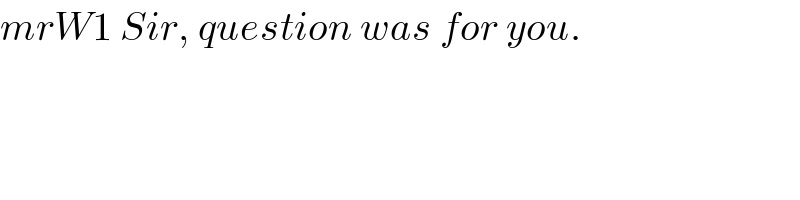
$${mrW}\mathrm{1}\:{Sir},\:{question}\:{was}\:{for}\:{you}. \\ $$
Commented by mrW1 last updated on 21/Dec/17

$${Interesting}\:{question}!\:{I}'{ll}\:{take}\:{a}\:{try}. \\ $$
Answered by ajfour last updated on 21/Dec/17

Answered by ajfour last updated on 21/Dec/17
![let a and b be roots of equation ((x−h)/(k−px^2 ))=2px ⇒ 2p^2 x^3 −2pkx+(x−h)=0 where x=x_(A ) or x_B hence ((2kx)/(2x^3 ))=a+b , ((x−h)/(2x^3 ))=ab ⇒ x^2 =(k/(a+b)) and ((2kx)/(x−h))=((a+b)/(ab)) =c (say) ....(i) hence 2kx=cx−ch x=((ch)/(c−2k)) or x−h=((2hk)/(c−2k)) ....(ii) and x^2 = (k/(a+b))=((c^2 h^2 )/((c−2k)^2 )) ...(iii) Also (x−h)^2 +(k−px^2 )^2 =r^2 and k−px^2 =((x−h)/(2px)) ⇒ (x−h)^2 [1+(1/(4p^2 x^2 ))]=r^2 using (ii): ((4h^2 k^2 )/((c−2k)^2 ))[4p^2 x^2 +1]=4r^2 p^2 x^2 ..(iv) from (i) x^2 =(k/(a+b)) , hence from (iii): (h^2 /((c−2k)^2 )) =(k/((a+b)c^2 )) so eq. (iv) becomes (k^3 /((a+b)c^2 ))[((4p^2 k)/(a+b))+1]=4r^2 p^2 ((k/(a+b))) ⇒ (k^2 /c^2 )(((4p^2 k)/(a+b))+1)=p^2 r^2 since p=a and p=b both satisfy above equation, so subtracting above eq. for p=a from that for p=b, (k^2 /c^2 )(((4k)/(a+b)))(b^2 −a^2 )=r^2 (b^2 −a^2 ) ⇒ k^3 =((c^2 r^2 (a+b))/4) and as c=((a+b)/(ab)) [from (i)], so k=(a+b)((r^2 /(4a^2 b^2 )))^(1/3) and from (iii), h^2 =((k(c−2k)^2 )/(c^2 (a+b))) .](Q26182.png)
$${let}\:{a}\:{and}\:{b}\:{be}\:{roots}\:{of}\:{equation} \\ $$$$\frac{{x}−{h}}{{k}−{px}^{\mathrm{2}} }=\mathrm{2}{px}\:\:\:\:\: \\ $$$$\Rightarrow\:\:\mathrm{2}{p}^{\mathrm{2}} {x}^{\mathrm{3}} −\mathrm{2}{pkx}+\left({x}−{h}\right)=\mathrm{0} \\ $$$${where}\:\:\:\:{x}={x}_{{A}\:} {or}\:\:{x}_{{B}} \: \\ $$$${hence}\:\:\:\frac{\mathrm{2}{kx}}{\mathrm{2}{x}^{\mathrm{3}} }={a}+{b}\:\:,\:\frac{{x}−{h}}{\mathrm{2}{x}^{\mathrm{3}} }={ab} \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} =\frac{{k}}{{a}+{b}}\:\:{and}\:\:\frac{\mathrm{2}{kx}}{{x}−{h}}=\frac{{a}+{b}}{{ab}}\:={c}\:\left({say}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:....\left({i}\right) \\ $$$${hence}\:\:\:\:\mathrm{2}{kx}={cx}−{ch} \\ $$$$\:{x}=\frac{{ch}}{{c}−\mathrm{2}{k}}\:\:\:{or}\:\:\:{x}−{h}=\frac{\mathrm{2}{hk}}{{c}−\mathrm{2}{k}}\:\:....\left({ii}\right) \\ $$$${and}\:\:{x}^{\mathrm{2}} =\:\frac{\boldsymbol{{k}}}{\boldsymbol{{a}}+\boldsymbol{{b}}}=\frac{\boldsymbol{{c}}^{\mathrm{2}} \boldsymbol{{h}}^{\mathrm{2}} }{\left(\boldsymbol{{c}}−\mathrm{2}\boldsymbol{{k}}\right)^{\mathrm{2}} }\:\:\:\:...\left({iii}\right) \\ $$$${Also}\:\:\left({x}−{h}\right)^{\mathrm{2}} +\left({k}−{px}^{\mathrm{2}} \right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$${and}\:\:\:\:{k}−{px}^{\mathrm{2}} =\frac{{x}−{h}}{\mathrm{2}{px}} \\ $$$$\Rightarrow\:\:\left({x}−{h}\right)^{\mathrm{2}} \left[\mathrm{1}+\frac{\mathrm{1}}{\mathrm{4}{p}^{\mathrm{2}} {x}^{\mathrm{2}} }\right]={r}^{\mathrm{2}} \\ $$$${using}\:\:\left({ii}\right): \\ $$$$\:\frac{\mathrm{4}{h}^{\mathrm{2}} {k}^{\mathrm{2}} }{\left({c}−\mathrm{2}{k}\right)^{\mathrm{2}} }\left[\mathrm{4}{p}^{\mathrm{2}} {x}^{\mathrm{2}} +\mathrm{1}\right]=\mathrm{4}{r}^{\mathrm{2}} {p}^{\mathrm{2}} {x}^{\mathrm{2}} \:\:..\left({iv}\right) \\ $$$${from}\:\:\left({i}\right)\:\:\:\:{x}^{\mathrm{2}} =\frac{{k}}{{a}+{b}}\:\:,\:{hence} \\ $$$${from}\:\left({iii}\right):\:\:\:\frac{{h}^{\mathrm{2}} }{\left({c}−\mathrm{2}{k}\right)^{\mathrm{2}} }\:=\frac{{k}}{\left({a}+{b}\right){c}^{\mathrm{2}} } \\ $$$${so}\:{eq}.\:\left({iv}\right)\:{becomes} \\ $$$$\frac{{k}^{\mathrm{3}} }{\left({a}+{b}\right){c}^{\mathrm{2}} }\left[\frac{\mathrm{4}{p}^{\mathrm{2}} {k}}{{a}+{b}}+\mathrm{1}\right]=\mathrm{4}{r}^{\mathrm{2}} {p}^{\mathrm{2}} \left(\frac{{k}}{{a}+{b}}\right) \\ $$$$\Rightarrow\:\:\:\:\:\:\frac{{k}^{\mathrm{2}} }{{c}^{\mathrm{2}} }\left(\frac{\mathrm{4}{p}^{\mathrm{2}} {k}}{{a}+{b}}+\mathrm{1}\right)={p}^{\mathrm{2}} {r}^{\mathrm{2}} \\ $$$${since}\:{p}={a}\:\:{and}\:\:{p}={b}\:{both}\:{satisfy} \\ $$$${above}\:{equation},\:{so}\:{subtracting} \\ $$$${above}\:{eq}.\:{for}\:{p}={a}\:{from}\:{that}\:{for}\:{p}={b}, \\ $$$$\:\:\:\:\:\:\:\frac{\boldsymbol{{k}}^{\mathrm{2}} }{\boldsymbol{{c}}^{\mathrm{2}} }\left(\frac{\mathrm{4}\boldsymbol{{k}}}{\boldsymbol{{a}}+\boldsymbol{{b}}}\right)\left(\boldsymbol{{b}}^{\mathrm{2}} −\boldsymbol{{a}}^{\mathrm{2}} \right)=\boldsymbol{{r}}^{\mathrm{2}} \left(\boldsymbol{{b}}^{\mathrm{2}} −\boldsymbol{{a}}^{\mathrm{2}} \right) \\ $$$$\Rightarrow\:\:\:\boldsymbol{{k}}^{\mathrm{3}} =\frac{\boldsymbol{{c}}^{\mathrm{2}} \boldsymbol{{r}}^{\mathrm{2}} \left(\boldsymbol{{a}}+\boldsymbol{{b}}\right)}{\mathrm{4}} \\ $$$${and}\:{as}\:\:\:{c}=\frac{{a}+{b}}{{ab}}\:\:\:\left[{from}\:\left({i}\right)\right],\:{so} \\ $$$$\:\:\:\:\:\:\:\boldsymbol{{k}}=\left(\boldsymbol{{a}}+\boldsymbol{{b}}\right)\left(\frac{\boldsymbol{{r}}^{\mathrm{2}} }{\mathrm{4}\boldsymbol{{a}}^{\mathrm{2}} \boldsymbol{{b}}^{\mathrm{2}} }\right)^{\mathrm{1}/\mathrm{3}} \\ $$$${and}\:\:{from}\:\:\left({iii}\right), \\ $$$$\:\:\:\:\:\:\:\boldsymbol{{h}}^{\mathrm{2}} =\frac{\boldsymbol{{k}}\left(\boldsymbol{{c}}−\mathrm{2}\boldsymbol{{k}}\right)^{\mathrm{2}} }{\boldsymbol{{c}}^{\mathrm{2}} \left(\boldsymbol{{a}}+\boldsymbol{{b}}\right)}\:. \\ $$
Commented by mrW1 last updated on 22/Dec/17
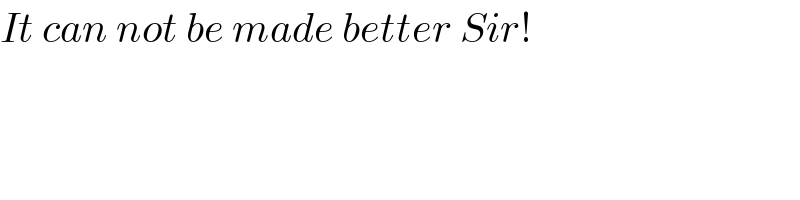
$${It}\:{can}\:{not}\:{be}\:{made}\:{better}\:{Sir}! \\ $$
Commented by ajfour last updated on 22/Dec/17

$${thank}\:{you}\:{Sir}\:! \\ $$
