Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 26755 by abdo imad last updated on 28/Dec/17

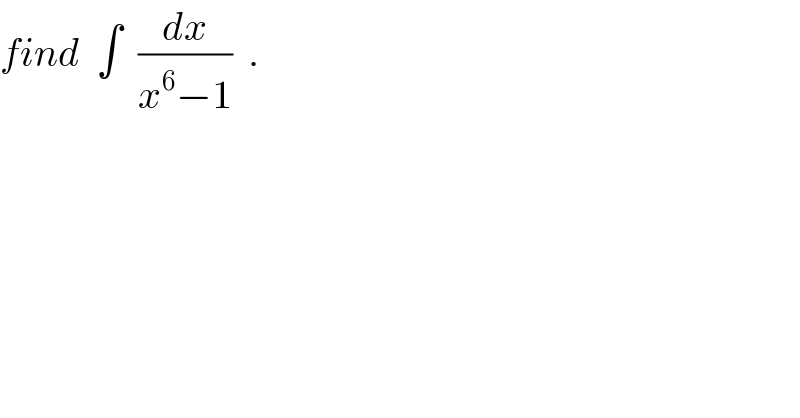
find ∫ (dx/(x^6 −1)) .

$${find}\:\:\int\:\:\frac{{dx}}{{x}^{\mathrm{6}} −\mathrm{1}}\:\:. \\ $$

Answered by $@ty@m last updated on 29/Dec/17

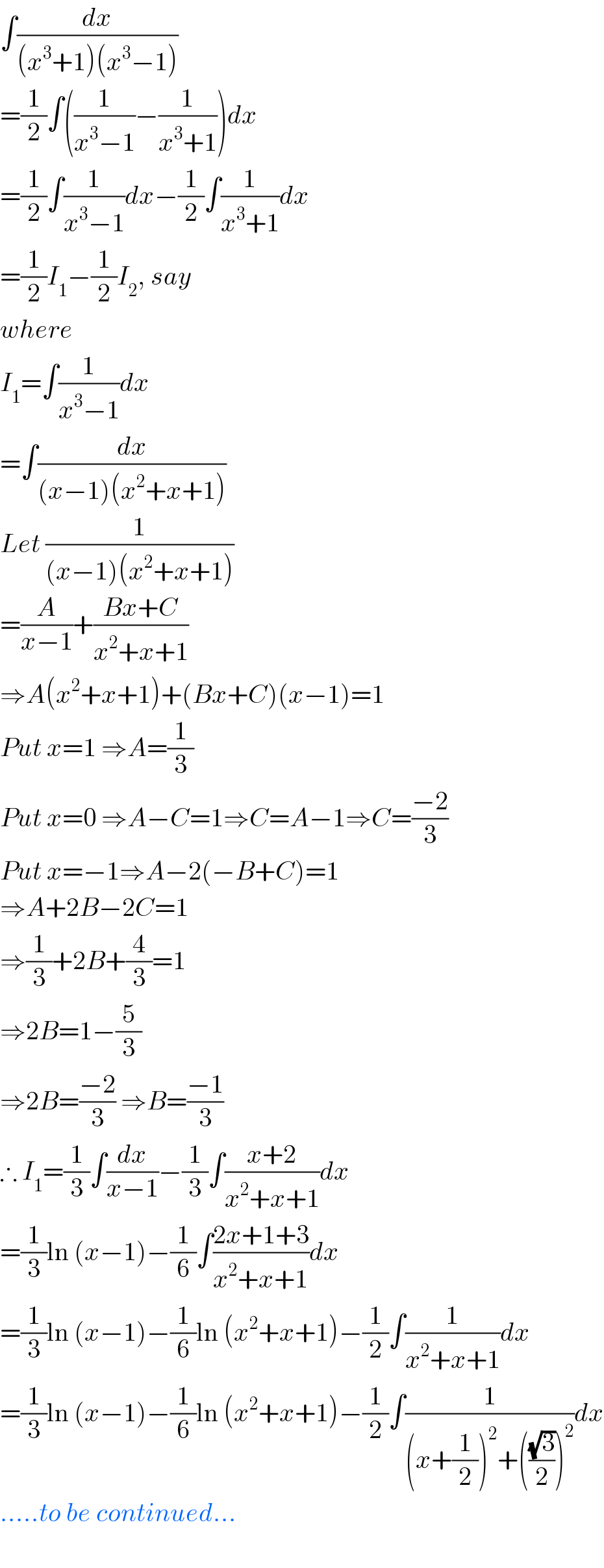
∫(dx/((x^3 +1)(x^3 −1))) =(1/2)∫((1/(x^3 −1))−(1/(x^3 +1)))dx =(1/2)∫(1/(x^3 −1))dx−(1/2)∫(1/(x^3 +1))dx =(1/2)I_1 −(1/2)I_2 , say where I_1 =∫(1/(x^3 −1))dx =∫(dx/((x−1)(x^2 +x+1))) Let (1/((x−1)(x^2 +x+1))) =(A/(x−1))+((Bx+C)/(x^2 +x+1)) ⇒A(x^2 +x+1)+(Bx+C)(x−1)=1 Put x=1 ⇒A=(1/3) Put x=0 ⇒A−C=1⇒C=A−1⇒C=((−2)/3) Put x=−1⇒A−2(−B+C)=1 ⇒A+2B−2C=1 ⇒(1/3)+2B+(4/3)=1 ⇒2B=1−(5/3) ⇒2B=((−2)/3) ⇒B=((−1)/3) ∴ I_1 =(1/3)∫(dx/(x−1))−(1/3)∫((x+2)/(x^2 +x+1))dx =(1/3)ln (x−1)−(1/6)∫((2x+1+3)/(x^2 +x+1))dx =(1/3)ln (x−1)−(1/6)ln (x^2 +x+1)−(1/2)∫(1/(x^2 +x+1))dx =(1/3)ln (x−1)−(1/6)ln (x^2 +x+1)−(1/2)∫(1/((x+(1/2))^2 +(((√3)/2))^2 ))dx .....to be continued...

$$\int\frac{{dx}}{\left({x}^{\mathrm{3}} +\mathrm{1}\right)\left({x}^{\mathrm{3}} −\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\left(\frac{\mathrm{1}}{{x}^{\mathrm{3}} −\mathrm{1}}−\frac{\mathrm{1}}{{x}^{\mathrm{3}} +\mathrm{1}}\right){dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}}{{x}^{\mathrm{3}} −\mathrm{1}}{dx}−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}}{{x}^{\mathrm{3}} +\mathrm{1}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{I}_{\mathrm{1}} −\frac{\mathrm{1}}{\mathrm{2}}{I}_{\mathrm{2}} ,\:{say} \\ $$$${where} \\ $$$${I}_{\mathrm{1}} =\int\frac{\mathrm{1}}{{x}^{\mathrm{3}} −\mathrm{1}}{dx} \\ $$$$=\int\frac{{dx}}{\left({x}−\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)} \\ $$$${Let}\:\frac{\mathrm{1}}{\left({x}−\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)} \\ $$$$=\frac{{A}}{{x}−\mathrm{1}}+\frac{{Bx}+{C}}{{x}^{\mathrm{2}} +{x}+\mathrm{1}} \\ $$$$\Rightarrow{A}\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)+\left({Bx}+{C}\right)\left({x}−\mathrm{1}\right)=\mathrm{1} \\ $$$${Put}\:{x}=\mathrm{1}\:\Rightarrow{A}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$${Put}\:{x}=\mathrm{0}\:\Rightarrow{A}−{C}=\mathrm{1}\Rightarrow{C}={A}−\mathrm{1}\Rightarrow{C}=\frac{−\mathrm{2}}{\mathrm{3}} \\ $$$${Put}\:{x}=−\mathrm{1}\Rightarrow{A}−\mathrm{2}\left(−{B}+{C}\right)=\mathrm{1} \\ $$$$\Rightarrow{A}+\mathrm{2}{B}−\mathrm{2}{C}=\mathrm{1} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{2}{B}+\frac{\mathrm{4}}{\mathrm{3}}=\mathrm{1} \\ $$$$\Rightarrow\mathrm{2}{B}=\mathrm{1}−\frac{\mathrm{5}}{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{2}{B}=\frac{−\mathrm{2}}{\mathrm{3}}\:\Rightarrow{B}=\frac{−\mathrm{1}}{\mathrm{3}} \\ $$$$\therefore\:{I}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{3}}\int\frac{{dx}}{{x}−\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{3}}\int\frac{{x}+\mathrm{2}}{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\mathrm{ln}\:\left({x}−\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{6}}\int\frac{\mathrm{2}{x}+\mathrm{1}+\mathrm{3}}{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\mathrm{ln}\:\left({x}−\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{6}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}}{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\mathrm{ln}\:\left({x}−\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{6}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}}{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)^{\mathrm{2}} }{dx} \\ $$$$.....{to}\:{be}\:{continued}... \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com