Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 2735 by prakash jain last updated on 25/Nov/15

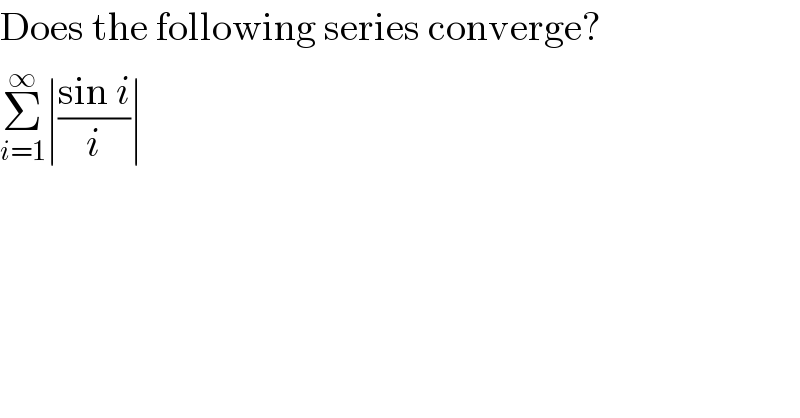
Does the following series converge? Σ_(i=1) ^∞ ∣((sin i)/i)∣

$$\mathrm{Does}\:\mathrm{the}\:\mathrm{following}\:\mathrm{series}\:\mathrm{converge}? \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\mid\frac{\mathrm{sin}\:{i}}{{i}}\mid \\ $$

Commented by Filup last updated on 26/Nov/15

There is a limit lim_(x→∞) ∣((sin x)/x)∣=∣lim_(x→∞) ((sin x)/x)∣ sine is bound such that sin(x)∈[0, 1] =∣((0 to 1)/∞)∣=∣0∣=0 ∴There is a limit such that: lim_(x→∞) ∣((sin x)/x)∣=0

$$\mathrm{There}\:\mathrm{is}\:\mathrm{a}\:\mathrm{limit} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\mid\frac{\mathrm{sin}\:{x}}{{x}}\mid=\mid\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{sin}\:{x}}{{x}}\mid \\ $$$$\mathrm{sine}\:\mathrm{is}\:\mathrm{bound}\:\mathrm{such}\:\mathrm{that}\:\mathrm{sin}\left({x}\right)\in\left[\mathrm{0},\:\mathrm{1}\right] \\ $$$$=\mid\frac{\mathrm{0}\:\mathrm{to}\:\mathrm{1}}{\infty}\mid=\mid\mathrm{0}\mid=\mathrm{0} \\ $$$$ \\ $$$$\therefore\mathrm{There}\:\mathrm{is}\:\mathrm{a}\:\mathrm{limit}\:\mathrm{such}\:\mathrm{that}: \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\mid\frac{\mathrm{sin}\:{x}}{{x}}\mid=\mathrm{0} \\ $$

Commented by Rasheed Soomro last updated on 26/Nov/15

Is (0/∞)=0? Is this not indeterminte case?

$${Is}\:\frac{\mathrm{0}}{\infty}=\mathrm{0}?\:{Is}\:{this}\:{not}\:{indeterminte}\:{case}? \\ $$

Commented by Filup last updated on 26/Nov/15

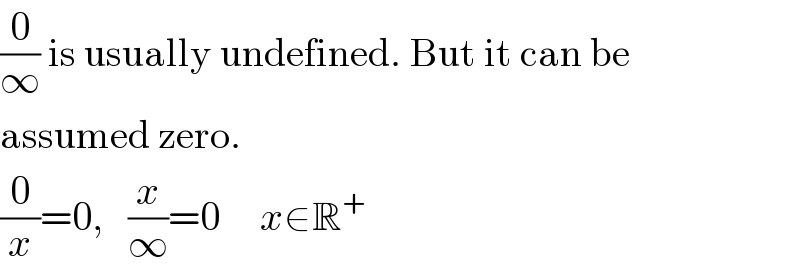
(0/∞) is usually undefined. But it can be assumed zero. (0/x)=0, (x/∞)=0 x∈R^+

$$\frac{\mathrm{0}}{\infty}\:\mathrm{is}\:\mathrm{usually}\:\mathrm{undefined}.\:\mathrm{But}\:\mathrm{it}\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{assumed}\:\mathrm{zero}. \\ $$$$\frac{\mathrm{0}}{{x}}=\mathrm{0},\:\:\:\frac{{x}}{\infty}=\mathrm{0}\:\:\:\:\:{x}\in\mathbb{R}^{+} \\ $$

Commented by prakash jain last updated on 26/Nov/15

0/∞ is not indeterminate, it is 0. Also 0^∞ is also zero. Indeterminate forms are 0^0 ,0∙∞, (∞/∞),∞−∞,∞^0 ,1^∞ ,(0/0)

$$\mathrm{0}/\infty\:\mathrm{is}\:\mathrm{not}\:\mathrm{indeterminate},\:\mathrm{it}\:\mathrm{is}\:\mathrm{0}. \\ $$$$\mathrm{Also}\:\mathrm{0}^{\infty} \:\mathrm{is}\:\mathrm{also}\:\mathrm{zero}. \\ $$$$\mathrm{Indeterminate}\:\mathrm{forms}\:\mathrm{are} \\ $$$$\mathrm{0}^{\mathrm{0}} ,\mathrm{0}\centerdot\infty,\:\frac{\infty}{\infty},\infty−\infty,\infty^{\mathrm{0}} ,\mathrm{1}^{\infty} ,\frac{\mathrm{0}}{\mathrm{0}} \\ $$

Commented by Filup last updated on 27/Nov/15

Oh? Well I learnt something!

$${O}\mathrm{h}?\:\mathrm{Well}\:\mathrm{I}\:\mathrm{learnt}\:\mathrm{something}! \\ $$

Commented by Filup last updated on 27/Nov/15

Why is ∞^0 and 1^∞ undefined? 1^n =1×1×...×1 n times 1^∞ is still 1 regardless, is it not?

$$\mathrm{Why}\:\mathrm{is}\:\infty^{\mathrm{0}} \:\mathrm{and}\:\mathrm{1}^{\infty} \:\mathrm{undefined}? \\ $$$$\mathrm{1}^{\mathrm{n}} =\mathrm{1}×\mathrm{1}×...×\mathrm{1}\:{n}\:\mathrm{times} \\ $$$$\mathrm{1}^{\infty} \:\mathrm{is}\:\mathrm{still}\:\mathrm{1}\:\mathrm{regardless},\:\mathrm{is}\:\mathrm{it}\:\mathrm{not}? \\ $$

Commented by RasheedAhmad last updated on 27/Nov/15

Are (0/∞)=0 and 0.∞=0 not equivalent? Why? What about (∞/0) ?

$${Are}\:\:\frac{\mathrm{0}}{\infty}=\mathrm{0}\:\:{and}\:\mathrm{0}.\infty=\mathrm{0}\:{not} \\ $$$${equivalent}?\:{Why}? \\ $$$${What}\:{about}\:\frac{\infty}{\mathrm{0}}\:? \\ $$

Commented by 123456 last updated on 27/Nov/15

1^∞ is undertemined because its depends of function A=lim_(x→0^+ ) (1+x)^(1/x) =e B=lim_(x→0^+ ) (1+x^2 )^(1/x) =1 A and B are limits of 1^∞ but take differents value same to ∞^0 C=lim_(x→+∞) x^(1/x) =1 D=lim_(x→+∞) (x^x )^(1/x) =+∞

$$\mathrm{1}^{\infty} \:\mathrm{is}\:\mathrm{undertemined}\:\mathrm{because}\:\mathrm{its}\:\mathrm{depends} \\ $$$$\mathrm{of}\:\mathrm{function} \\ $$$$\mathrm{A}=\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} ={e}\: \\ $$$$\mathrm{B}=\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{1}/{x}} =\mathrm{1} \\ $$$$\mathrm{A}\:\mathrm{and}\:\mathrm{B}\:\mathrm{are}\:\mathrm{limits}\:\mathrm{of}\:\mathrm{1}^{\infty} \:\mathrm{but}\:\mathrm{take} \\ $$$$\mathrm{differents}\:\mathrm{value} \\ $$$$\mathrm{same}\:\mathrm{to}\:\infty^{\mathrm{0}} \\ $$$$\mathrm{C}=\underset{{x}\rightarrow+\infty} {\mathrm{lim}}\:{x}^{\mathrm{1}/{x}} =\mathrm{1} \\ $$$$\mathrm{D}=\underset{{x}\rightarrow+\infty} {\mathrm{lim}}\:\left({x}^{{x}} \right)^{\mathrm{1}/{x}} =+\infty \\ $$

Answered by Filup last updated on 26/Nov/15

for ∣sin i∣ as i→∞, it is a repetition of the sum of values between [0, 1] The sum can be evaluated as: Σ_(i=1) ^∞ ((∣sin i∣)/i) ∵i>0, ∴∣i∣=i for (a/b)=c, For a∈[0, +∞), as b→∞, c→0 For i∈[1, +∞), ∣sin i∣∈(0, 1)=c Σ_(i=1) ^∞ c(1/i)=c×∞ Harmonic sequence More correctly: Σ_(i=1) ^∞ ∣((sin i)/i)∣=Σ_(i=1) ^∞ ((0 to 1)/i)=cΣ_(i=1) ^∞ (1/i) =c×∞ Does not converge

$${for}\:\mid\mathrm{sin}\:{i}\mid\:\mathrm{as}\:{i}\rightarrow\infty, \\ $$$$\mathrm{it}\:\mathrm{is}\:\mathrm{a}\:\mathrm{repetition}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{values} \\ $$$$\mathrm{between}\:\left[\mathrm{0},\:\mathrm{1}\right] \\ $$$$ \\ $$$$\mathrm{The}\:\mathrm{sum}\:\mathrm{can}\:\mathrm{be}\:\mathrm{evaluated}\:\mathrm{as}: \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mid\mathrm{sin}\:{i}\mid}{{i}}\:\:\:\:\:\:\:\:\:\because{i}>\mathrm{0},\:\therefore\mid{i}\mid={i} \\ $$$$ \\ $$$$\mathrm{for}\:\frac{{a}}{{b}}={c},\:\:\:\mathrm{For}\:{a}\in\left[\mathrm{0},\:+\infty\right),\:\mathrm{as}\:{b}\rightarrow\infty,\:\:{c}\rightarrow\mathrm{0} \\ $$$$\mathrm{For}\:{i}\in\left[\mathrm{1},\:+\infty\right),\:\mid\mathrm{sin}\:{i}\mid\in\left(\mathrm{0},\:\mathrm{1}\right)={c} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}{c}\frac{\mathrm{1}}{{i}}={c}×\infty\:\:\mathrm{Harmonic}\:\mathrm{sequence} \\ $$$${More}\:{correctly}: \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\mid\frac{\mathrm{sin}\:{i}}{{i}}\mid=\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{0}\:\mathrm{to}\:\mathrm{1}}{{i}}={c}\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{i}} \\ $$$$={c}×\infty \\ $$$${Does}\:{not}\:{converge} \\ $$

Commented by Filup last updated on 26/Nov/15

The proof above is kind of informal, but I do believe the concept is correct.

$$\mathrm{The}\:\mathrm{proof}\:\mathrm{above}\:\mathrm{is}\:\mathrm{kind}\:\mathrm{of}\:\mathrm{informal}, \\ $$$$\mathrm{but}\:\mathrm{I}\:\mathrm{do}\:\mathrm{believe}\:\mathrm{the}\:\mathrm{concept}\:\mathrm{is}\:\mathrm{correct}. \\ $$

Commented by prakash jain last updated on 26/Nov/15

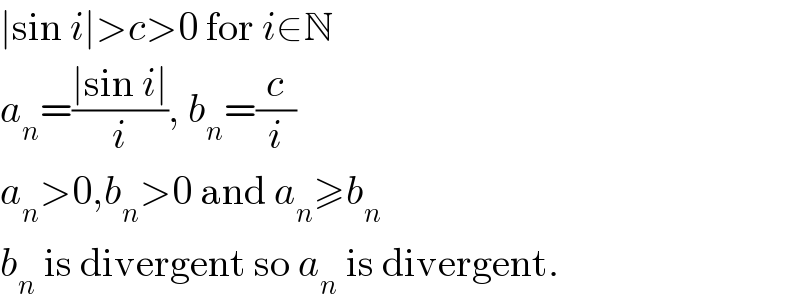
∣sin i∣>c>0 for i∈N a_n =((∣sin i∣)/i), b_n =(c/i) a_n >0,b_n >0 and a_n ≥b_n b_n is divergent so a_n is divergent.

$$\mid\mathrm{sin}\:{i}\mid>{c}>\mathrm{0}\:\mathrm{for}\:{i}\in\mathbb{N} \\ $$$${a}_{{n}} =\frac{\mid\mathrm{sin}\:{i}\mid}{{i}},\:{b}_{{n}} =\frac{{c}}{{i}} \\ $$$${a}_{{n}} >\mathrm{0},{b}_{{n}} >\mathrm{0}\:\mathrm{and}\:{a}_{{n}} \geqslant{b}_{{n}} \\ $$$${b}_{{n}} \:\mathrm{is}\:\mathrm{divergent}\:\mathrm{so}\:{a}_{{n}} \:\mathrm{is}\:\mathrm{divergent}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com