Question Number 27445 by Tinkutara last updated on 07/Jan/18
$${If}\:{a}\:{planet}\:{is}\:{suddenly}\:{stopped}\:{in}\:{its} \\ $$$${orbit},\:{supposed}\:{to}\:{be}\:{circular},\:{then}\:{it} \\ $$$${would}\:{fall}\:{into}\:{the}\:{sun}\:{in}\:{a}\:{time}\:\frac{{T}}{\mathrm{4}\sqrt{\mathrm{2}}}, \\ $$$${where}\:{T}\:{is}\:{the}\:{time}\:{period}\:{of} \\ $$$${revolution}.\:{Prove}\:{this}. \\ $$
Answered by mrW1 last updated on 07/Jan/18
![Gravitation force between planet and sun: F=((GMm)/L^2 ) with M = mass of sun m = mass of planet L = distance between them F=((mv^2 )/L)=((GMm)/L^2 ) ⇒v=(√((GM)/L))=velocity of planet in orbit T=((2πL)/v)=2πL(√(L/(GM))) free fall of planet into sun from height x= L to 0: F(x)=((GMm)/x^2 )=ma ⇒a=((GM)/x^2 )=−v(dv/dx) ⇒vdv=−GM (dx/x^2 ) ⇒∫_0 ^( v) vdv=−GM ∫_L ^( x) (dx/x^2 ) ⇒(1/2)v^2 =GM((1/x)−(1/L))=((GM(L−x))/(Lx)) ⇒v=(√((2GM)/L)) (√((L−x)/x))=−(dx/dt) ⇒−(√((2GM)/L)) dt=(√(x/(L−x))) dx ⇒−(√((2GM)/L)) ∫_0 ^( T_1 ) dt=∫_L ^( 0) (√(x/(L−x))) dx ⇒−(√((2GM)/L)) T_1 =[−(√(x(L−x)))−L tan^(−1) (√((L−x)/x))]_L ^0 =−((Lπ)/2) ⇒T_1 =((πL)/2)×(√(L/(2GM)))=(1/(4(√2)))×2πL (√(L/(GM))) ⇒T_1 =(T/(4(√2)))](Q27455.png)
$${Gravitation}\:{force}\:{between}\:{planet} \\ $$$${and}\:{sun}: \\ $$$${F}=\frac{{GMm}}{{L}^{\mathrm{2}} } \\ $$$${with}\:{M}\:=\:{mass}\:{of}\:{sun} \\ $$$${m}\:=\:{mass}\:{of}\:{planet} \\ $$$${L}\:=\:{distance}\:{between}\:{them} \\ $$$${F}=\frac{{mv}^{\mathrm{2}} }{{L}}=\frac{{GMm}}{{L}^{\mathrm{2}} } \\ $$$$\Rightarrow{v}=\sqrt{\frac{{GM}}{{L}}}={velocity}\:{of}\:{planet}\:{in}\:{orbit} \\ $$$${T}=\frac{\mathrm{2}\pi{L}}{{v}}=\mathrm{2}\pi{L}\sqrt{\frac{{L}}{{GM}}} \\ $$$$ \\ $$$${free}\:{fall}\:{of}\:{planet}\:{into}\:{sun}\:{from} \\ $$$${height}\:{x}=\:{L}\:{to}\:\mathrm{0}: \\ $$$${F}\left({x}\right)=\frac{{GMm}}{{x}^{\mathrm{2}} }={ma} \\ $$$$\Rightarrow{a}=\frac{{GM}}{{x}^{\mathrm{2}} }=−{v}\frac{{dv}}{{dx}} \\ $$$$\Rightarrow{vdv}=−{GM}\:\frac{{dx}}{{x}^{\mathrm{2}} } \\ $$$$\Rightarrow\int_{\mathrm{0}} ^{\:{v}} {vdv}=−{GM}\:\int_{{L}} ^{\:{x}} \frac{{dx}}{{x}^{\mathrm{2}} } \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{2}}{v}^{\mathrm{2}} ={GM}\left(\frac{\mathrm{1}}{{x}}−\frac{\mathrm{1}}{{L}}\right)=\frac{{GM}\left({L}−{x}\right)}{{Lx}} \\ $$$$\Rightarrow{v}=\sqrt{\frac{\mathrm{2}{GM}}{{L}}}\:\sqrt{\frac{{L}−{x}}{{x}}}=−\frac{{dx}}{{dt}} \\ $$$$\Rightarrow−\sqrt{\frac{\mathrm{2}{GM}}{{L}}}\:{dt}=\sqrt{\frac{{x}}{{L}−{x}}}\:{dx} \\ $$$$\Rightarrow−\sqrt{\frac{\mathrm{2}{GM}}{{L}}}\:\int_{\mathrm{0}} ^{\:{T}_{\mathrm{1}} } {dt}=\int_{{L}} ^{\:\mathrm{0}} \sqrt{\frac{{x}}{{L}−{x}}}\:{dx} \\ $$$$\Rightarrow−\sqrt{\frac{\mathrm{2}{GM}}{{L}}}\:{T}_{\mathrm{1}} =\left[−\sqrt{{x}\left({L}−{x}\right)}−{L}\:\mathrm{tan}^{−\mathrm{1}} \sqrt{\frac{{L}−{x}}{{x}}}\right]_{{L}} ^{\mathrm{0}} =−\frac{{L}\pi}{\mathrm{2}} \\ $$$$\Rightarrow{T}_{\mathrm{1}} =\frac{\pi{L}}{\mathrm{2}}×\sqrt{\frac{{L}}{\mathrm{2}{GM}}}=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}×\mathrm{2}\pi{L}\:\sqrt{\frac{{L}}{{GM}}} \\ $$$$\Rightarrow{T}_{\mathrm{1}} =\frac{{T}}{\mathrm{4}\sqrt{\mathrm{2}}} \\ $$
Commented by Tinkutara last updated on 07/Jan/18
How do you solved the integral? As in question 27400 it is giving another answer.
Commented by mrW1 last updated on 07/Jan/18
![I=∫_L ^( 0) (√(x/(L−x))) dx =L∫_L ^( 0) (√((x/L)/(1−(x/L)))) d(x/L) =L∫_1 ^( 0) (√(λ/(1−λ))) dλ with λ=(x/L) let (1/t)=(√(λ/(1−λ))) ⇒1−λ=t^2 λ ⇒λ=(1/(1+t^2 )) dλ=−((2t)/((1+t^2 )^2 )) ∫(√(λ/(1−λ))) dλ=−2∫(1/((1+t^2 )^2 ))dt =−2[(t/(2(1+t^2 )))+(1/2)tan^(−1) t] =−(t/(1+t^2 ))−tan^(−1) t =−(√(λ(1−λ)))−tan^(−1) (√((1−λ)/λ)) =−(√((x/L)(1−(x/L))))−tan^(−1) (√((1−(x/L))/(x/L))) =−(1/L)(√(x(L−x)))−tan^(−1) (√((L−x)/x)) I=∫_L ^( 0) (√(x/(L−x))) dx =[−(√(x(L−x)))−L tan^(−1) (√((L−x)/x))]_L ^0 =−((Lπ)/2)](Q27462.png)
$${I}=\int_{{L}} ^{\:\mathrm{0}} \sqrt{\frac{{x}}{{L}−{x}}}\:{dx} \\ $$$$={L}\int_{{L}} ^{\:\mathrm{0}} \sqrt{\frac{\frac{{x}}{{L}}}{\mathrm{1}−\frac{{x}}{{L}}}}\:{d}\frac{{x}}{{L}} \\ $$$$={L}\int_{\mathrm{1}} ^{\:\mathrm{0}} \sqrt{\frac{\lambda}{\mathrm{1}−\lambda}}\:{d}\lambda \\ $$$${with}\:\lambda=\frac{{x}}{{L}} \\ $$$$ \\ $$$${let}\:\frac{\mathrm{1}}{{t}}=\sqrt{\frac{\lambda}{\mathrm{1}−\lambda}} \\ $$$$\Rightarrow\mathrm{1}−\lambda={t}^{\mathrm{2}} \lambda \\ $$$$\Rightarrow\lambda=\frac{\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${d}\lambda=−\frac{\mathrm{2}{t}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$$\int\sqrt{\frac{\lambda}{\mathrm{1}−\lambda}}\:{d}\lambda=−\mathrm{2}\int\frac{\mathrm{1}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }{dt} \\ $$$$=−\mathrm{2}\left[\frac{{t}}{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} {t}\right] \\ $$$$=−\frac{{t}}{\mathrm{1}+{t}^{\mathrm{2}} }−\mathrm{tan}^{−\mathrm{1}} {t} \\ $$$$=−\sqrt{\lambda\left(\mathrm{1}−\lambda\right)}−\mathrm{tan}^{−\mathrm{1}} \sqrt{\frac{\mathrm{1}−\lambda}{\lambda}} \\ $$$$=−\sqrt{\frac{{x}}{{L}}\left(\mathrm{1}−\frac{{x}}{{L}}\right)}−\mathrm{tan}^{−\mathrm{1}} \sqrt{\frac{\mathrm{1}−\frac{{x}}{{L}}}{\frac{{x}}{{L}}}} \\ $$$$=−\frac{\mathrm{1}}{{L}}\sqrt{{x}\left({L}−{x}\right)}−\mathrm{tan}^{−\mathrm{1}} \sqrt{\frac{{L}−{x}}{{x}}} \\ $$$$ \\ $$$${I}=\int_{{L}} ^{\:\mathrm{0}} \sqrt{\frac{{x}}{{L}−{x}}}\:{dx} \\ $$$$=\left[−\sqrt{{x}\left({L}−{x}\right)}−{L}\:\mathrm{tan}^{−\mathrm{1}} \sqrt{\frac{{L}−{x}}{{x}}}\right]_{{L}} ^{\mathrm{0}} \\ $$$$=−\frac{{L}\pi}{\mathrm{2}} \\ $$
Commented by Tinkutara last updated on 07/Jan/18
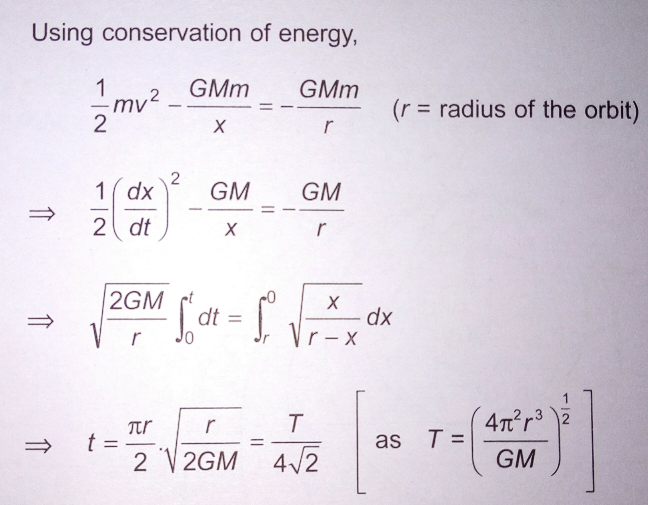
Commented by Tinkutara last updated on 07/Jan/18
This was book's solution. Where is mistake here then? The integral comes out negative but here it shows positive.
Commented by mrW1 last updated on 07/Jan/18
$${To}\:{be}\:{exact},\:{the}\:\mathrm{3}{rd}\:{and}\:\mathrm{4}{th}\:{line}\:{in}\:{book}\:{is}\:{not}\:{correct}. \\ $$$${in}\:\mathrm{3}{rd}\:{line}\:{it}\:{should}\:{be}\:\left(−\frac{{dx}}{{dt}}\right)^{\mathrm{2}} \:{as} \\ $$$${replacement}\:{for}\:{v}^{\mathrm{2}} ,\:{since} \\ $$$${with}\:{the}\:{definition}\:{for}\:{x}−{axis}\:{we} \\ $$$${have}\:{v}=−\frac{{dx}}{{dt}},\:{on}\:{one}\:{side}\:{of}\:\mathrm{4}{th}\:{line} \\ $$$${a}\:``−''\:{sign}\:{is}\:{missing}. \\ $$$$ \\ $$$${sincef}\left({x}\right)=\:\sqrt{\frac{{x}}{{r}−{x}}\:}\:{is}\:{positive}, \\ $$$$\int_{{r}} ^{\:\mathrm{0}} {f}\left({x}\right){dx}\:{is}\:{always}\:{negative}. \\ $$$$ \\ $$$${I}\:{think}\:{the}\:{book}\:{doesn}'{t}\:{work}\:{so} \\ $$$${cleanly}. \\ $$